Momentum of a rack and pinion gear system excited by a time variant force

Question

Background

I have a rack and pinion gear system as shown in the image below

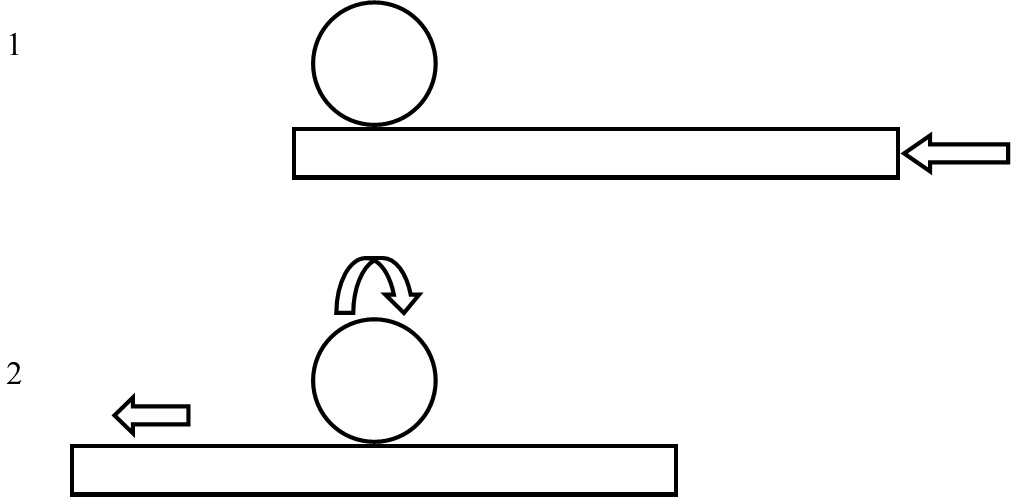
The pinion gear is attached to a flywheel at the back.

The first state of the system, none of the gears or the flywheel move. A time variant force is then applied to the rack, denoted by the arrow. The force function is similar in shape to a normal distribution.

The second state of the system, the pinion gear and flywheel rotate, and the rack moves in the direction of the arrow.

I would like to know what the angular velocity of the flywheel is. I have read somewhere that rack and pinion system have a 85-90% mechanical efficiency. Does that mean that 85-90% of the translation energy is converted to rotational energy or only 10-15%?

What I have done thus far

$$ m\frac {d\ddot{x}}{dt} = F(t)-loss$$

$$ I_1\frac {d\ddot{\theta}_1}{dt} = F(t)*r_1 - F_{f1}*r_2 $$

$$ I_2\frac {d\ddot{\theta}_2}{dt} = max(F(t)*r_3) - F_{f2}*r_2 $$

$F(t)$ is the applied force

$ {I_1} $ is the inertia of the pinion

$ \ddot\theta_1 $ is the angular acceleration of the pinion

$r_1$ is the radius of the pinion

$F_{f1}$ is the friction force between the pinion and the shaft ($ \mu_s*F_N $)

r2 is the radius of the shaft the pinion is on

$ {I_2} $ is the inertia of the flywheel

$ \ddot\theta_2 $ is the angular acceleration of the flywheel

$r_3$ is the radius of the flywheel

$F_{f2}$ is the friction force between the flywheel and the shaft ($ \mu_s*F_N $)

The question

Now, I know that something is not right here. How does the kinetic energy of the rack transfer to the pinion gear? This deceleration of the rack is the $Loss$ term, since I do not know how to formulate it, and then the same force (reduced by 10-15% or 85-90%) must then be transferred to the pinion and subsequently the flywheel.

Answer 1

The loss of energy (efficiency<1) manifests itself in two ways

1. Friction. When doing free body diagrams add frictional forces at the sliding contacts and find which coefficient of friction $\mu$ gives you the efficiency values you expect.
2. Structural damping (hysteresis). This is more difficult to put in the equations of motion, because they assume perfectly rigid bodies, and this phenomenon manifests itself when bodies flex. In your situation you might consider the shaft between the pinion and the flywheel as flexible and include a rotational spring damper system connecting the two. Again you can tune the parameters to get the desired effect.

To simulate the dynamics you recognize there are two degrees of freedom in the system. One is the rotation of the flywheel $\theta$ and the other the location of the rack $x$ (and corresponding pinion rotation $\varphi$). The applied transient force on the rack is $F(t)$ and the tangential force on the pinion $F_G$. Proportional to the tangential force, there is a separating force on the gear (radial load) because the teeth contact at a wedge angle, such that $S_G = F_H \tan \eta$. This force causes friction on the rack which is estimated with $F_{friction} = \mu\, S_G = \mu (\tan \eta F_G)$.

• Kinematics: $$\left. x = r \varphi \right\} \left. \dot{x} = r \dot{\theta} \right\} \ddot{x} = r \ddot{\theta}$$
• Forces on Rack: $$F(t)-F_G - F_{friction} = m_{rack} \ddot{x}$$
• Torques on Pinion: $$ r\, F_G - \tau = I_{pinion} \ddot{\varphi} $$
• Torques on Flywheel: $$ \tau = I_{flywheel} \ddot{\theta} $$
• Transmitted Torque on shaft: $$ \tau = k\,(\varphi-\theta) + c\,(\dot{\varphi}-\dot{\theta}) $$

For the rotational stiffness $k$ and damping $c$, I would parametrize them according to the (undamped) natural frequency of the system $\omega_n$ and the damping ratio $\zeta$. $$\begin{align} I_{sys} & = I_{flywheel} + I_{pin} + m_{rack} r^2 \\ k & = I_{sys} \omega^2_n \\ c & = 2 \zeta I_{sys} \omega_n \end{align} $$

Now you have all the elements of a differential system of equations to be solved for $\ddot{\theta}$ and $\ddot{\varphi}$ (and hence $\ddot{x}$).

Answer 2

First of all, the transmission of force between any two pairs of mating gears happens due to surface contact between the two and hence through friction. Next, any loss is a dissipation in energy. Though I am not that well-versed in vibration analysis, I know that an periodic dissipation of energy is accounted in the force-equation using a damping coefficient. You might like to read up on 'Coulomb Damping'.

As far as the question of efficiency goes, 85 % efficient means that 85 % of the input energy is transmitted from the driver shaft to the driven shaft and that 15 % is lost in transmission.