2
$\begingroup$

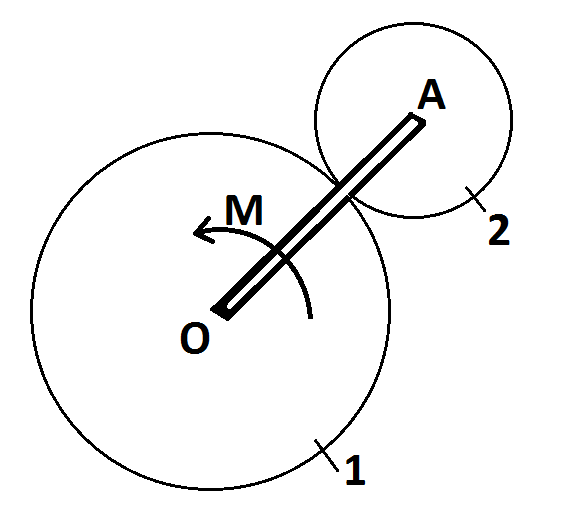
I am having a homework: enter image description here

"A planetary gear system with a fixed gear 1 (radius r1); gear 2 (radius r2) is movable". At begining, the system is stationary. Apply a constant torque M to OA bar. OA bar rotates about O and cause gear 2 moving. OA has weight Q, Gear 2 has weight P. Calculate the angular acceleration of OA bar."

I am doing this homework with two approach and they gives different answers:

Approach 1: Energy method

Let the angular velocity of OA bar is $\omega$

Kinetic energy of OA bar = $\frac{1}{2}\frac{Q}{g}\frac{(r_1+r_2)^2}{3}\omega^2$

Kinetic energy of gear 2 = $\frac{1}{2}(\frac{1}{2}\frac{P}{g}r_2^2)\omega_2^2+ \frac{1}{2}\frac{P}{g}v_A^2$

$\omega_2 = \frac{r_1+r_2}{r_2}\omega$

$v_A = (r_1+r_2)\omega$

Hence, total kinetic energy = $\frac{1}{2}\frac{2Q+9P}{6g}(r_1+r_2)^2\omega^2$ = total work = M$\phi$

Differentiate two side, give angular acceleration $\gamma$= $\frac{6Mg}{(2Q+9P)(r_1+r_2)^2}$

Approach 2: angular momentum method

Angular momentum of OA with respect to point O = $\frac{1}{3}\frac{Q}{g}(r_1+r_2)^2\omega$

Angular momentum of gear 2 with respect to point A = $\frac{1}{2}\frac{P}{g}r_2^2\omega_2$

Angular momentum of gear 2 with respect to point O = Angular momentum of gear 2 with respect to point A + $\frac{P}{g}OAv_A$ = $\frac{1}{2}\frac{P}{g}r_2^2\omega_2 + \frac{P}{g}\omega(r_1+r_2)^2$

Hence, total angular momentum of system with respect to point O = $\frac{1}{3}\frac{Q}{g}(r_1+r_2)^2\omega + \frac{1}{2}\frac{P}{g}r_2^2\omega(r_1+r_2)/r_2 + \frac{P}{g}\omega(r_1+r_2)^2$

Differentiate above term give us: $\gamma(\frac{1}{3}\frac{Q}{g}(r_1+r_2)^2 + \frac{1}{2}\frac{P}{g}r_2^2(r_1+r_2)/r_2 + \frac{P}{g}(r_1+r_2)^2) = M$

Hence $\gamma = \frac{6Mg}{(2Q+9P)(r_1+r_2)^2-3Pr_1(r_1+r_2)}$

The two results are different, what I am missing ?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Approach 1 is correct.

You haven't considered all the torques acting on the system (rod + gear 2) in approach 2.

The force corresponding to the missing torque, is responsible for maintaining the pure-rolling motion of gear 2 (constraint: point of contact instantaneously at rest) on the surface of gear 1 at all times. The torque you're missing doesn't do work on the system which is why approach 1 gave the correct answer even though you didn't realize the presence of this torque. I will leave you to figure out this missing torque.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Is the missing torque is the torque to keep gear 1 stationary?. Without it, gear 1 would be moving? $\endgroup$
    – Dat
    Commented Dec 2, 2019 at 11:54
  • $\begingroup$ No. The missing torque arises from the force of interaction between gear 1 and 2: the force is very similar to the frictional force that is responsible for enforcing the "no-slipping" constraint between the two discs. This missing torque acting on gear 1 can be counter-acted by the torque applied by the ground/pivot to give a zero net torque: That's why gear 1 remains stationary. And this missing torque acting on gear 2 is responsible for the angular acceleration of gear 2 ($\dot{\omega}_2$). To see this, write down $\frac{dL_{CM}}{dt}= \tau_{CM}$ considering the system to be gear 2 alone. $\endgroup$
    – Ajay Mohan
    Commented Dec 2, 2019 at 12:37
  • $\begingroup$ (contd.) CM is the center of mass of gear 2: point A in the figure. Your first step should be to find the force of interaction between gear 1 and gear 2 that's tangential to the two discs. Let me know if you're unable to understand/proceed. $\endgroup$
    – Ajay Mohan
    Commented Dec 2, 2019 at 12:43
  • $\begingroup$ I can only express the force of interation between gear 1 and gear 2 to a function of $\gamma$. Then add momen of this force with respect to O in approach 2, then solve the equation for $\gamma$ $\endgroup$
    – Dat
    Commented Dec 2, 2019 at 16:50
  • $\begingroup$ Yes, it is a function of $\gamma$ since $\dot{\omega}_2$ is a function of $\gamma$. Did the result from approach 2 now match with the result from approach 1? $\endgroup$
    – Ajay Mohan
    Commented Dec 2, 2019 at 17:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.