1
$\begingroup$

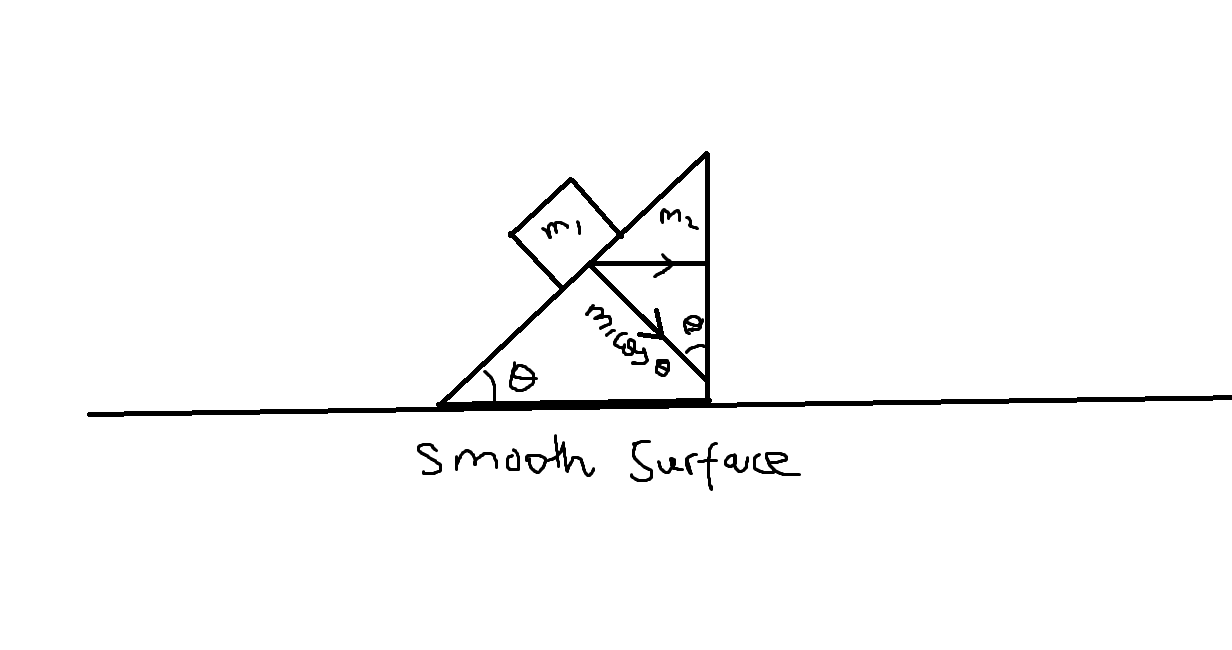
enter image description here

In the above diagram, both the surfaces are frictionless. The force exerted by $m_1$ perpendicular to the inclined plane $m_2$'s surface is $m_1g\cos\theta$, and the horizontal component of that force is $m_1g\cos\theta \sin\theta$. Now if I want to find the acceleration of $m_2$, why is $$a = \frac{m_1g\cos\theta \sin\theta}{m_1+m_2}$$ wrong? To calculate the acceleration wouldn't we have to consider the mass of the entire system rather than only $m_2$?

Additional question: since the inclined plane $m_2$ is moving to the right with an acceleration, shouldn't $m_1$ "fall" and land on $m_2$ again? what causes it to stick to $m_2$?

Improve this question
$\endgroup$
6
  • $\begingroup$ Your assumption that the normal reaction between m1 and m2 is m1gsin(theta) is wrong. This only applies when there is no component of acceleration perpendicular to the interface, eg when m2 is stationary. In this case there is such a component of acceleration. The effect is that it reduces the normal reaction, as though the value of g were reduced. The situation is more complicated than it appears. $\endgroup$
    – sammy gerbil
    Commented Apr 25, 2016 at 16:58
  • $\begingroup$ The way I solved the problem years ago was to resolve all forces and accelerations into components parallel and perpendicular to the interface between m1 and m2. Also note that the CM of the sytem remains in the same horizontal position as the blocks move. $\endgroup$
    – sammy gerbil
    Commented Apr 25, 2016 at 17:02
  • $\begingroup$ If you resolve m1g, the component normal to the plane would be m1gcos(theta) . The inclined plane would have to provide a normal reaction of m1gcos(theta) or the block would sink into the plane. Where am I going wrong here? $\endgroup$
    – xasthor
    Commented Apr 25, 2016 at 17:19
  • $\begingroup$ Sorry I made a typo with sin instead of cos for normal reaction.... The wedge is accelerating to the right, away from m1; this acceleration has a component in the direction opposite to the normal reaction. It is as though m1 is in a lift which is accelerating downwards; the normal reaction between m1 and m2 will be reduced. $\endgroup$
    – sammy gerbil
    Commented Apr 26, 2016 at 1:25
  • $\begingroup$ The way I'm looking at it is: The horizontal component of m1gcos(theta) which is usually balanced by the force of friction when the wedge is at rest, is not balanced this time since the surface is frictionless and is accelerating to the right therefore the normal contact force is decreased. Is this correct? $\endgroup$
    – xasthor
    Commented Apr 26, 2016 at 2:06

1 Answer 1

Reset to default
2
$\begingroup$

  1. If you want to find the acceleration of a mass $m_2$, you divide the force $F$ acting on it by the mass: $a=F/m_2$. No exceptions.

  2. You seem confused about how this system is intended to work - the two masses are separately moving - the $m_1$ slides down the inclined plane and pushes the inclined plane to the right during that motion. It doesn't "fall" when the plane is moving, and it doesn't stick to it either; it's sliding, and the two motions ($m_1$ sliding down $m_2$, $m_2$ moving to the right) occur simultaneously, it's not that one of the two objects moves and then the other catches up (that sort of physics only happens in cartoons).

Improve this answer
$\endgroup$
9
  • $\begingroup$ Regarding 1), if the inclined plane is accelerating to the right with the block on top of it, wouldn't the two masses act as a system together making the acceleration = F/m1+m2? $\endgroup$
    – xasthor
    Commented Apr 25, 2016 at 15:32
  • $\begingroup$ @xasthor: No. The force you are computing there is the force $m_1$ exerts on $m_2$ - it doesn't make sense to say that $m_1$ also exerts that force on itself, does it? $\endgroup$
    – ACuriousMind ♦
    Commented Apr 25, 2016 at 15:33
  • $\begingroup$ Sorry if I'm bothering you, but I'm having a hard time understanding this. Because m1 is on top of m2, I'm thinking it would add to its mass and kind of "slow" it down. I know what I'm thinking is wrong, but could you please tell me where I'm going wrong and correct it? $\endgroup$
    – xasthor
    Commented Apr 25, 2016 at 15:45
  • $\begingroup$ @xasthor: Well, I don't understand what you are thinking - you have two bodies, and they exert forces upon each other, and then they move according to those forces. Why do you think there is something like "adding to its mass" going on? $\endgroup$
    – ACuriousMind ♦
    Commented Apr 25, 2016 at 15:48
  • $\begingroup$ If there's a block B with mass B on top of block A with mass A, and the system is free falling then the forces acting on A would be gB + gA, so the net force would be g(A+B). Now if I wanna calculate the acceleration I'd divide it by (A+B) instead of just A. So why can't I apply a similar logic to this case? $\endgroup$
    – xasthor
    Commented Apr 25, 2016 at 15:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.