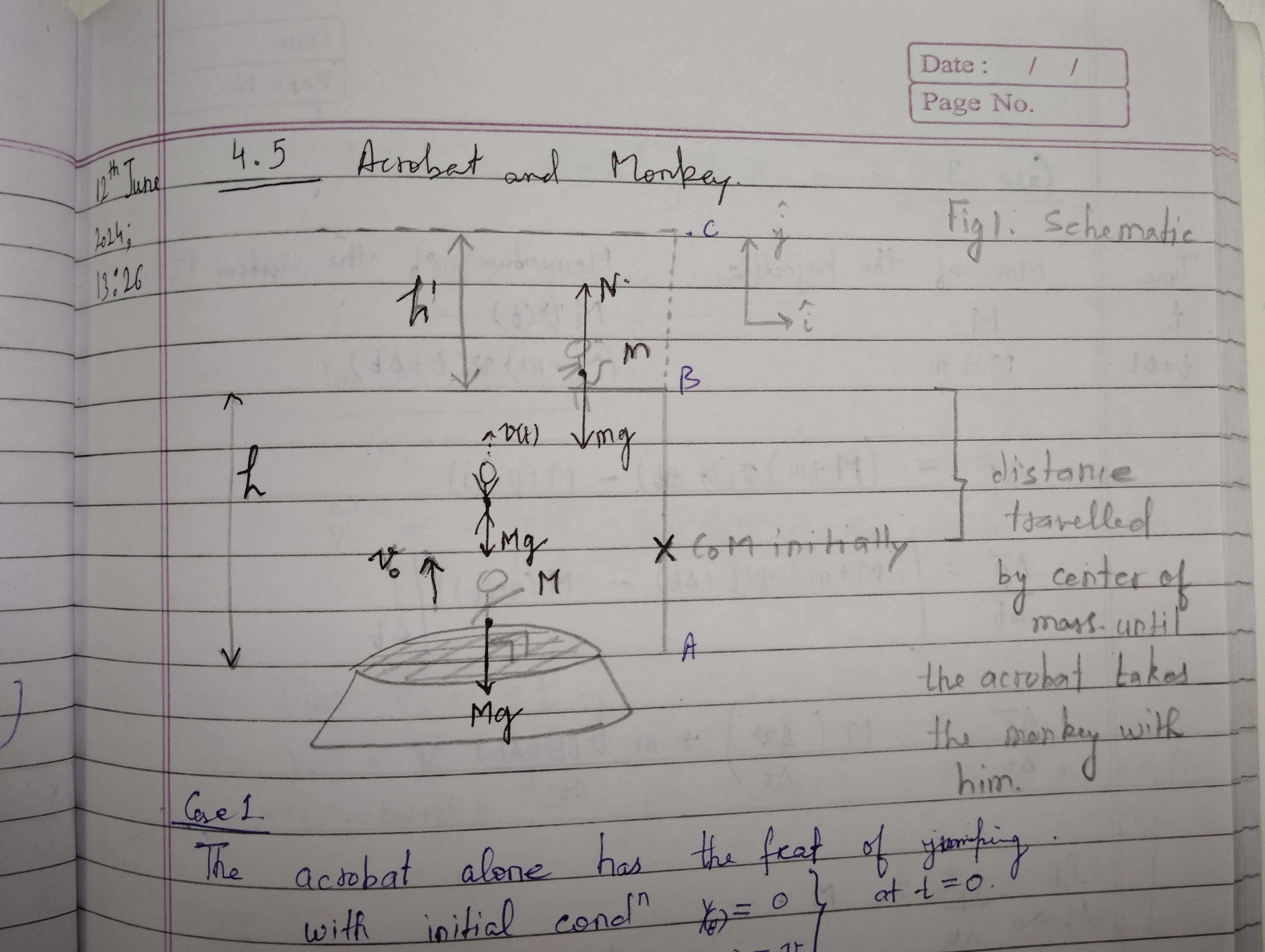
A circus acrobat of mass $M$ leaps straight up with initial velocity $v_{0}$ from a trampoline. As he rises up, he takes a trained monkey of mass $m$ off a perch at a height $h$ above the trampoline. What is the maximum height attained by the pair?
Center of Mass
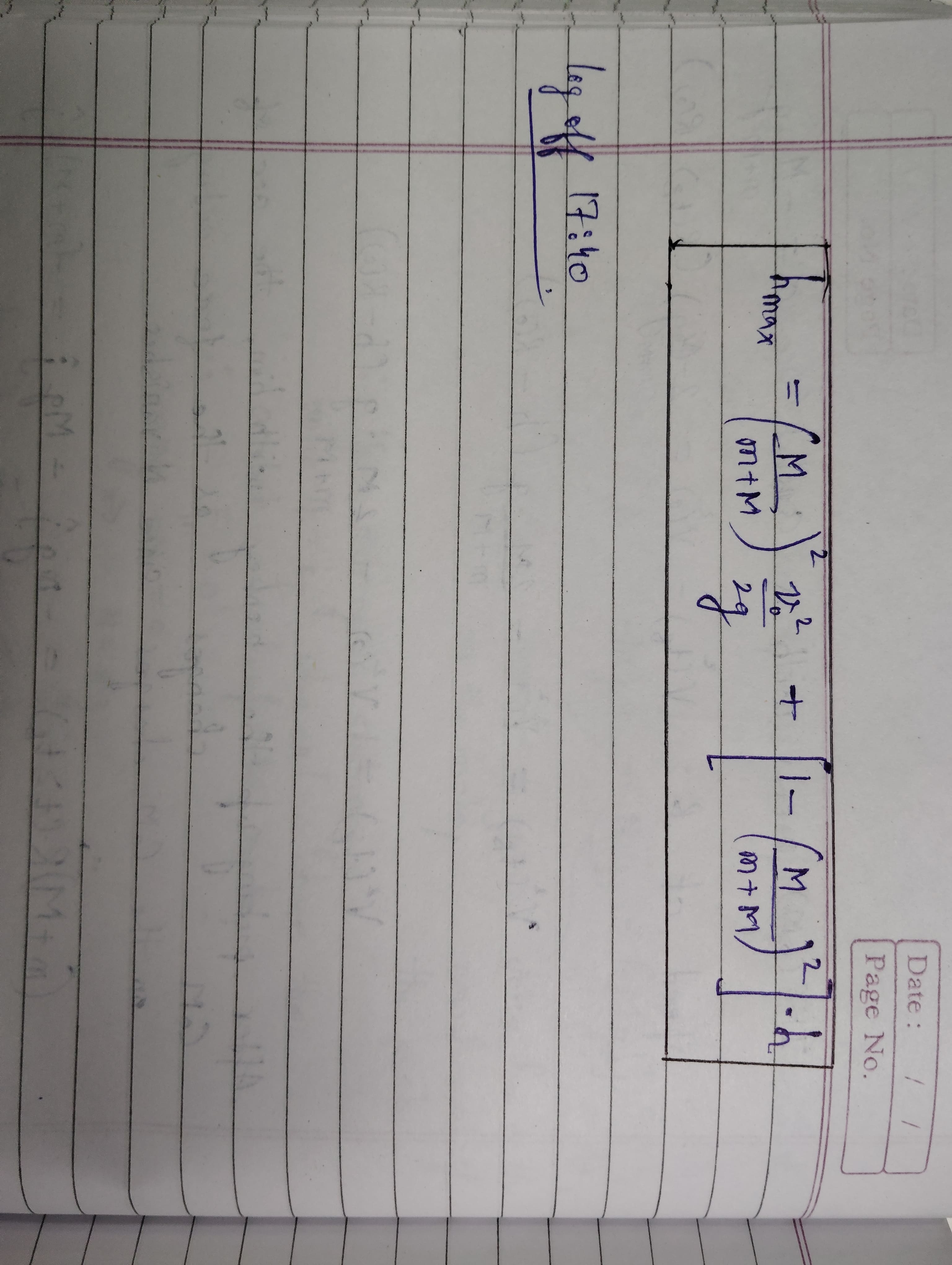
Initially the center of mass(CM) is at $y_{0} = \dfrac{mh}{M+m}$. The only force on the CM is gravity and its initial velocity is $\dfrac{Mv_{0}}{M+m}$. So it is going to reach its highest point traveling a distance of $\dfrac{M^{2}v_{0}^{2}}{2(M+m)^{2}g}$ from its initial position $y_{0}$. This leaves the pair at a final distance of $\dfrac{mh}{M+m} + \dfrac{M^{2}v_{0}^{2}}{2(M+m)^{2}g}$ above the ground
Conservation of Energy
Initial Energy of the pair, $E_{0} = \frac{1}{2}Mv_{0}^{2} + mgh$ Final Energy of the pair, $E_{1} = (M+m)gH$, where $H$ is the maximum height above the ground that the pair reaches.
By conservation of energy, $E_{0} = E_{1} \implies (M+m)gH = \frac{1}{2}MV_{0}^{2} + mgh \implies H = \dfrac{Mv_{0}^{2} + 2mgh}{2(M+m)g}$
Conservation of momentum
At a height of $h$, the velocity of M is $v = \sqrt{v_{0}^{2} - 2gh}$. Just after picking up the monkey, the velocity of the pair, $v^{'}$ is given by $Mv = (M+m)v^{'}$ (Why should the momentum be conserved for the acrobat-monkey system?), they will go on to a height of $\dfrac{v^{'2}}{2g}$ from here.
The three approaches don't seem to tally. Where am I wrong?