According to the definition of potential energy, we use $U= mhg . $ In the figure below ,
A thin uniform rod of mass m and length h is positioned vertically above an anchored frictionless pivot point.
Why does a author say that potential energy is $U =\dfrac{mgh}2$ why not $U =mgh$
Similar problem I faced on a problem, when I had to find out the potential energy for lifting water from a hole with height h.
So my problem is, when should I consider center of mass and when I should not?
Imagine your rod is made up from lots of little bricks stacked on each other. Then the total potential energy is the sum of the potential energy of each brick.
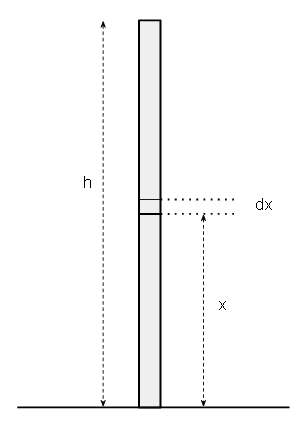
The diagram shows the rod and one of the bricks of size $dx$ and at a height $x$. If $\rho$ is the mass per unit length, then the mass of the brick is $\rho \space dx$, and the potential energy is $\rho \space dx \space g \space x$. To get the total potential energy we just have to sum up the potential energies of all the bricks. To do the sum we let $dx$ go to zero and replace the sum with an integral so:
$$ \begin{align} U &= \rho \space g \int_0^h dx . x \\ &= \rho \space g \left[\frac{x^2}{2}\right]_0^h \\ &= \frac{\rho g h^2}{2} \end{align} $$
And since $\rho h$ is just the mass $m$ this gives us:
$$ U = \frac{mgh}{2} $$
As you say, this is the same result you get by just considering the centre of mass, but note that we've got this result without involving the centre of mass at all. I think this is a better way to understand why the potential energy is $mgh/2$ without just invoking the centre of mass and waving your arms in the air.
You always consider centre of mass. The height $h$ in the equation $U = mgh$ is the height of the centre of mass. In your case, the rod is of height $h$, so the centre of mass is at $\dfrac{h}{2}$
No work is required to rotate the rod about its center by 90 degrees. The center of the rod is at height $h/2$. The PE of a horizontal rod at a height $h/2$ is $m g h/2$.
Another way to see this: If all of the mass were concentrated at the top of the rod, the PE would be $m g h$. If all of the mass were concentrated at the bottom of the rod, the PE would be 0. The average of these two values is $m g h/2$.
