0
$\begingroup$

I have one system in mind. Although I know it is not possible to accelerate a particle to a higher speed without spending energy, I would like to know why the proposed system won't work.

The system is as follows:-

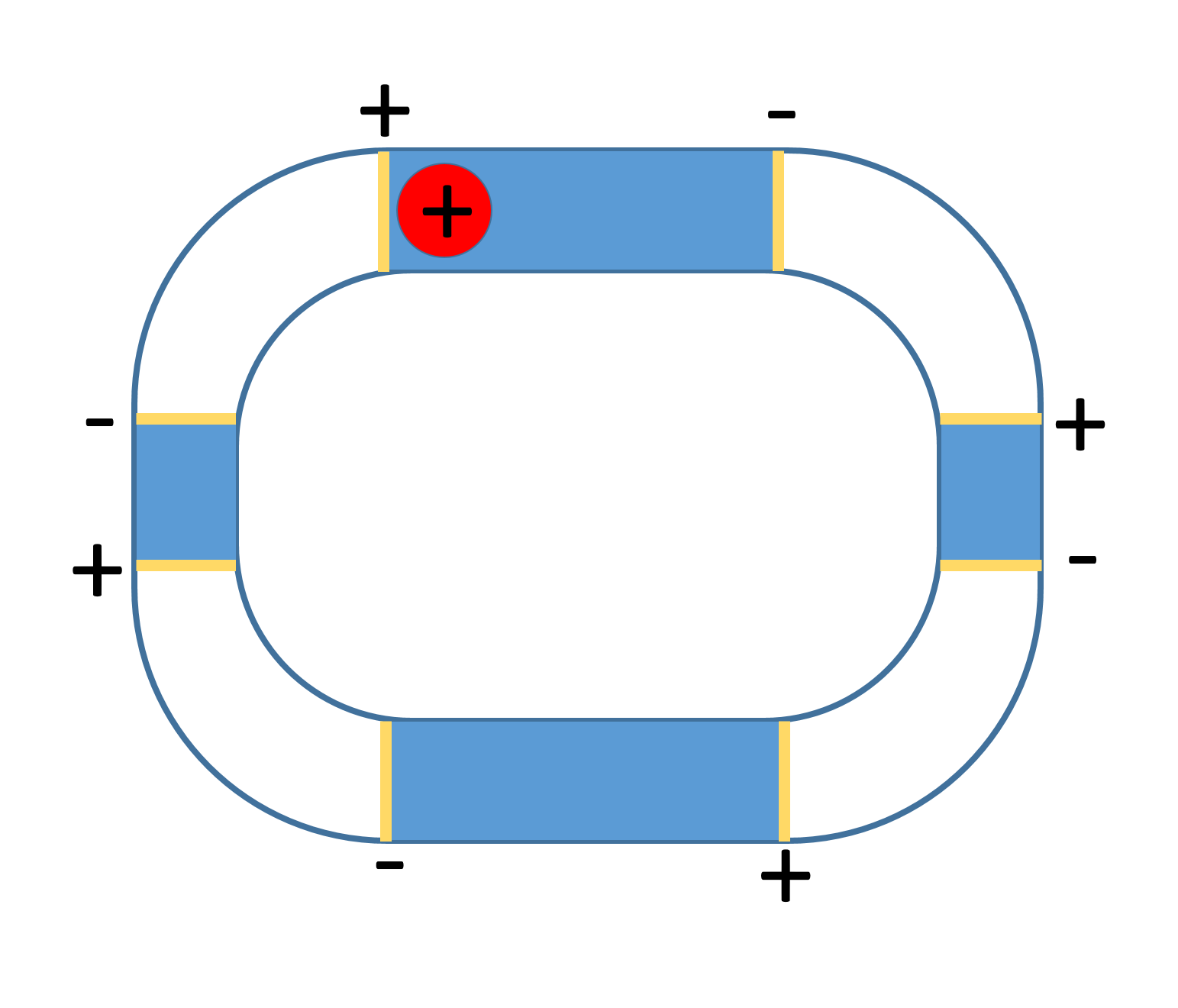
There are four tubes connected to each other through curved joining tubes giving it the shape of a square with rounded corners. Charges of opposite polarity are placed at the ends of each tube, in a convenient shape (a sheet or a sphere). A charged sphere is then brought near a tube ending with similar charge. The charge at this end of the tube repels the sphere and the charge at the other end attracts it. Then the charge begins moving and when it reaches the other end, it moves through the charge out of the tube and then through the connecting tube, enter another tube and the whole process repeats.

The tubes are made of a suitable material that prevents electric field from interacting with the charged sphere once it is out of the tube (and in the connecting tube). Also, the square is just a shape I thought of, it can also be a straight tube with no curves (so that the charge does not lose its velocity while changing direction).The blue region are the tubes (sorry they aren't in proportion). The colorless regions are the connecting tubes. The yellow lines are charges (preferably sheet charges). The red ball is the particle in motion.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ You should recognize that your question title can be rewritten as "can I build a perpetual motion machine" or "can I have free energy" (seriously: you're asking to add energy to the accelerated object without taking it from somewhere). $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 18, 2016 at 18:37

2 Answers 2

Reset to default
1
$\begingroup$

To answer the question in the title: No, because this would be akin to creating energy. Since this is ruled out by energy conservation, which is pretty established, we can safely say that it is impossible.

As to the question why your specific proposal does not work: Note that in between the blue areas, the particle will actually be decelerated. Inside the blue tubes, there is a net force in the direction of movement, outside, there is a net force in the opposite direction.

If you consider equal spacing between the charges along the ring and consider equal charges of + or -, it is quite simple to see that the force becomes zero at - and that it is symmetric with respect to every + or -. Starting at rest, the charge will then accelerate until it reaches the - point and then decelerate until it reaches the + point and oscillate between the two --> no infinite acceleration. The oscillation will be damped with friction.

If you don't have equal spacing or unequal charges, the maths becomes slightly less obvious, but the result will be the same - you'll only ever get an oscillation. In order to do this, write down the forces at any point and add them up.

Improve this answer
$\endgroup$
5
  • $\begingroup$ For this reason, I never proposed only the ring system. What about when the whole system is opened up into a straight line? Also, as the tube casings are made of material impenetrable to electric fields, the charge should not be enacted upon by any forces when outside the blue tubes. $\endgroup$
    – Shrey Gupta
    Commented Feb 18, 2016 at 18:55
  • 2
    $\begingroup$ A good superconductor is more or less a "material impenetrable to electric fields", but they develop surface charge distributions which defeat your proposal before it even gets off the ground. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 18, 2016 at 19:00
  • $\begingroup$ First of all, my reasoning never used the ring design, so the open system is irrelevant. Second, you can't have impenetrable material. "The charge should not be enacted upon by any forces when outside the blue tubes": And this is your problem: this doesn't exist in the way you want it. $\endgroup$
    – Martin
    Commented Feb 18, 2016 at 19:05
  • $\begingroup$ The only conventional way I can think of is to use a Faraday cage. This won't work, because the Faraday cage produces opposite charges to negate the effect and my reasoning will still apply. $\endgroup$
    – Martin
    Commented Feb 18, 2016 at 19:08
  • $\begingroup$ In a ring, a real accelerator is called a synchrotron, in a spiral a cyclotron, in a line a linac. However, while each is simple in concept, they are more complex than you idea. However, unlike your idea, they will actually accelerate ions to high energies. $\endgroup$
    – Jon Custer
    Commented Feb 18, 2016 at 19:48
1
$\begingroup$

First of all, no. In any system, the energy is conserved. So whatever happens the initial and final energies have to be equal.

In your experiment, you seem to have brought four charges near each other and then using another charged sphere to set these charges in motion. Not considering gravity or friction, The process of bringing these charges near each other itself will require energy as you will have to work against their potentials. So you cant just "place" these charges near each other.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I am not trying to move the fixed charges at the ends of each tube. I am just moving the test charge. $\endgroup$
    – Shrey Gupta
    Commented Feb 18, 2016 at 18:39
  • $\begingroup$ While I didnt think about the motion of the charges itself, Martin explains it excellently in his answer. What I am saying is, that you assumed the charges to be in the tube. You would have to work (spend energy) to get all those charges together. $\endgroup$
    – Marcus
    Commented Feb 18, 2016 at 19:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.