3
$\begingroup$

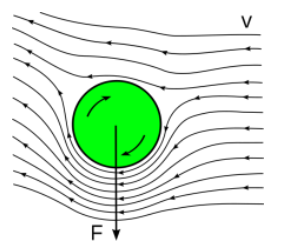
If you solve the Bernoulli equation: $$p=p_0-\rho_0{v^2 \over 2}$$ using a complex flow potential for a flow around a cylinder: $$W(z)=v_0 z + {v_0 R^2 \over z} - {\Gamma \over 2 \pi } \ln(z)$$

you get the forces per length $\vec f$ by using an integral over preassure $\vec f = - \int_{\text Cr} p \;\vec n ds $ which yields $F_x=0$ and $F_y=\rho_0 \Gamma v_0$

1) Does the direction of the force in this otherwise symmetrical problem stem from the direction of the circulation?

2)In all pictures like this one the cylinder is rotating why is that? Is it because drawing a circulation is hard, so the circulation in the picture is represented by a spinning cylinder?

Magnus effect with spinning cylinder

Improve this question
$\endgroup$
3
  • $\begingroup$ The magnus effect only applies to a translating and rotating object. Well, it could be stationary while rotating in a wind tunnel, but the point is that the rotation changes the location of the stagnation point and alters the flow around the object. This can lead to a force orthogonal to the direction of the bulk fluid flow... $\endgroup$
    – honeste_vivere
    Commented Feb 10, 2016 at 13:42
  • $\begingroup$ So what about my questions? Are my conclusions wrong or wright? $\endgroup$
    – Kuhlambo
    Commented Feb 11, 2016 at 15:18
  • $\begingroup$ @pinkakass - Yes to 1st and yes the circulation in that image is for a spinning cylinder. $\endgroup$
    – honeste_vivere
    Commented Feb 11, 2016 at 15:24

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.