8
$\begingroup$

When force is applied on extended objects at some point, the work done by this force on this object $\vec{F} \cdot \vec{dr_{P}}$ where $P$ is the point of application of force. When the object is rolling and friction is acting on it, the point at which it acts is moving with respect to the ground frame, yet work done by friction in rolling is zero.

I would have thought that work is force times distance. How can this be?

Improve this question
$\endgroup$
2
  • $\begingroup$ When force is applied there is no displacement. When it had displaced there is no force. $\endgroup$
    – Anubhav Goel
    Commented Feb 8, 2016 at 16:51
  • $\begingroup$ See this answer to the question Are we doing work when we compress a ball?. It clearly explains the difference between the 'usual' work and the 'centre of mass' work which would clarify doubts regarding this question. $\endgroup$
    – Vishnu
    Commented Jul 4, 2020 at 7:24

2 Answers 2

Reset to default
7
$\begingroup$

The point in which the friction acts is not actually moving, but rather as the object rolls past any given point, a new point of friction takes over. See in the animation below how a point on the circle touches briefly:

enter image description here

Improve this answer
$\endgroup$
1
  • 7
    $\begingroup$ More importantly, the motion of a point on the circle near the contact is perpendicular to the friction force. In the diagram above, the red curve is perpendicular to the surface at the point of contact. So the work (the dot product of force and displacement) is zero. $\endgroup$
    – John Alexiou
    Commented Oct 22, 2018 at 16:42
3
$\begingroup$

The interesting thing is that in the diagram shown above the frictional force is zero so there is no way a frictional force can do any work.
If there was a frictional force acting on rolling object then there would be a torque about its centre of mass and so there would be an angular acceleration.
Also if there was a frictional force acting on the rolling object there would be a net horizontal force acting on it and its centre of mass would undergo a translational acceleration.
Neither of these accelerations happen so there is no frictional force.

In such situation on horizontal surface the only time that there is a kinetic frictional force is when there is slipping at the point of contact. The frictional force will act in such a way as to make the rotating object reach a no slipping situation.

Suppose that the object is not rolling but its centre of mass has a translational velocity. A kinetic frictional force will act in the opposite direction to the translation motion. This frictional force will have two effects.

  • Because the frictional force is in the opposite direction to the motion of the object it will reduce the velocity of the centre of mass of the object.
  • Because the frictional frictional force is applying a torque about the centre of mass of the object it will make the object rotate.

This continues until $v = r \omega$ the no slipping condition. $v$ is the linear speed of the centre of mass of the object, $r$ is its radius and $\omega$ is the angular speed of the object.

enter image description here

Later
If an external force is applied then what happens next depends on whwere the force is applied.
I such case the external force does work on the rolling object and some of that work is converted into heat because of the work done by a frictional force and the rest increases both the rotational and translational kinetic energies.

Suppose that you have an object rolling (no slipping) along a horizontal surface so $v=r\omega$.

A force $F$ is applied which is parallel to the surface and through the centre of mass of the rolling object.

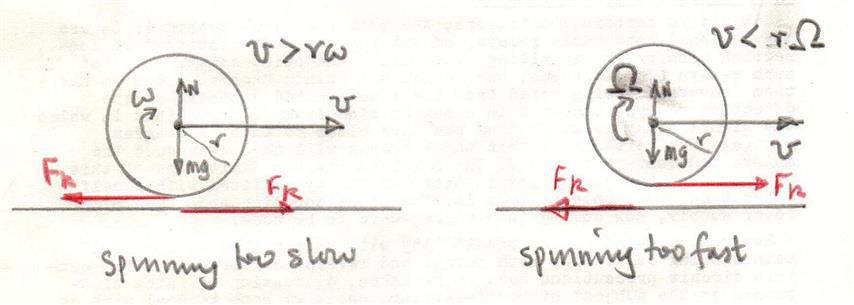
Assume that in the diagrams above the force acts from left to right.

You now have the rotating too slowly situation.

The net force which produces a translational accelerates of the centre of mass is $F-F_k$ and the torque about the centre of mass which produces the angular acceleration is $F_k r$.

Application of the force at other points are just variations of the description above.

Improve this answer
$\endgroup$
8
  • $\begingroup$ My question is the case when there is static friction acting on the object and is rolling. $\endgroup$
    – TESLA____
    Commented Feb 8, 2016 at 18:35
  • $\begingroup$ The value of the static frictional force is zero if there is no slipping. Have you read above what would happen if there was a frictional force? Remember that the static frictional force. Can take any value from $0$ to $\mu_s N$ depending on the situation. A lot of rotational dynamics is counterintuitive. $\endgroup$
    – Farcher
    Commented Feb 8, 2016 at 19:08
  • $\begingroup$ There will be static friction when an external force is acting on the body and it is rolling. $\endgroup$
    – TESLA____
    Commented Feb 9, 2016 at 4:47
  • $\begingroup$ i will add an answer to your comment at the end of my answer above. $\endgroup$
    – Farcher
    Commented Feb 9, 2016 at 6:29
  • $\begingroup$ You said work done by external force is converted to heat due to work done by frictional force. But work done by frictional force in rolling is zero and my doubt is how ? $\endgroup$
    – TESLA____
    Commented Feb 9, 2016 at 9:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.