I want to know how an atom is when it is excited.
If an atom, due to collision of another fast moving atom, becomes fast moving as well, is that also an "excited state"?
Edit: I guess the answer is that there are two explanation for "excitation":
Introduction
In general, a Physical System State is described by a set of variables Let’s consider the “System Internal Energy” variable
System States
A System is said to be in its “Ground State” when it is at the lowest possible energy level Any other State is then an “Excited State” and they would correspond to energy level greater than the ground state’s one (by definition it’s the minimum level)
Example
Let’s consider as Physical System the Hydrogen Atom which is formed by a proton and an electron.
It is a bound quantum mechanical system so energy level are discrete. Let’s assume the electron is in the lowest possible orbital (s1) : that’s the system’s ground state.
If you provide the right amount of energy (remember that the energy spectrum is discrete) by means of a photon hitting the system, then system will absorb the photon’s energy and store it as “internal energy” with the electron jumping to outer orbitals. So then the system moves to an excited state as it is not in its ground state anymore (its unique electron has changed orbital).
Consider that in general systems tend to minimize their potential energy so “excited states” are unstable: the longer the system stays excited, the higher the probability of a decay.
To conclude our example let’s consider the case of the atom becoming an ion, it happens if the amount of energy absorbed is so high that the electron can overcome the negative potential barrier of the bound state and become a “free particle” (actually you have to remember about particle-wave duality).
In that case you can’t say the system is excited, it has completely changed: it is not bound qm system composed of proton and electron anymore.
Excitation is an elevation in energy level above an arbitrary baseline energy state.
"In English, please!"
So what this is effectively saying is that an atom is considered "excited" when its energy level is higher than the rest. This can be manifested as heat, light, etc. For example, the Aurora Borealis. The Aurora is when radiation from the sun excited the atoms in the air. These atoms have to go back to the baseline, so they release energy as light.
Your kitchen stove is another example. The molecules of the water right next to the heater have sped up, so they start smashing into other molecules next to them, exciting them. And thus the pot heats up.
Basically electrons prefer to stay in least energy level in an atom. If certain amout of energy is given to it then it jumps to a higher energy level. There are discrete enegry levels so e- would accept only some particular energy to get exicted to higher energy level. When it returns to a lower state it gives out the energy in form of photons. Search hydrogen spectrum for more.
Consider the following model of an atom:
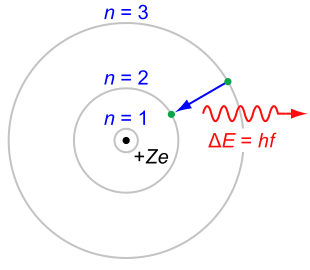
Keep in mind that it is only a model and while it is a good model that elevates our understanding of the subatomic world, it is still just a model and reality will look different. How exactly? We don't know. The model is good enough, though, to understand what an excited atom is.
With this caveat out of the way, we can first try to understand what the ground state is. In the middle you have the protons and the neutrons which are densely packed to form the nucleus. It is positively charged by $Ze$, where $Z$ is the number of protons and $e$ is the unit charge. The same number $Z$ of electrons must orbit the nucleus to make this atom neutral. If we consider $Z = 1$, we have only one electron, and we can ignore rules, where exactly the electron is allowed to be due to the presence of other electrons (see Pauli Principle for further info).
The energy levels of the atom are quantized. That means there are only certain levels where the electron is allowed to orbit. In the picture they are shown by the gray circles ("shells"). If the electron sits on the innermost shell ($n=1$), it has the lowest energy. If it sits on the second shell ($n=2$), it has more energy, and so on. Typically, an atom wants to be in its ground state, where it has the lowest possible energy, i.e. where the electron is on the first shell. When the electron orbits on another shell than the first one, we call the atom excited. Exciting an atom can happen e.g. by irradiation, where the electron absorbs energy from a photon (light) to get to one of the outer shells. After a while, the electron will go back to the innermost shell, i.e. the atom returns to its ground state. Since it is in a lower energy state, energy conservation tells us, that it needs to emit the left over energy. This is done by emitting a photon, with a very typical wavelength (see spectral lines for further info). In the picture, this is shown by the red wavy line, where $\Delta E$ is the energy difference between the 2nd and the 3rd shell and also the energy the photon will carry away.
