0
$\begingroup$

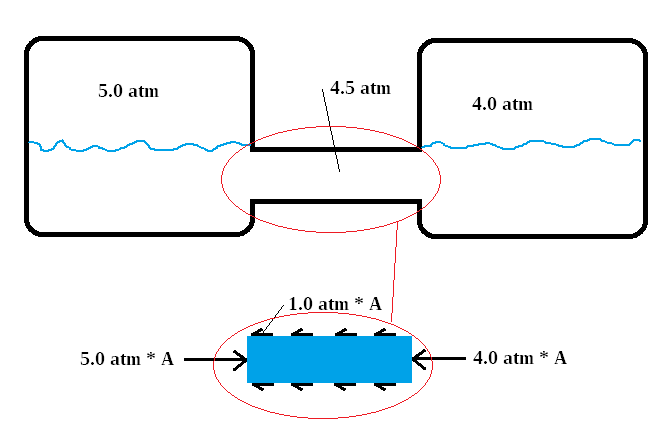
The problem is

How much work is done by pressure in forcing $1.4 m^3$ of water through a pipe having an internal diameter of $13 mm$ if the difference in pressure at the two ends of the pipe is $1.0 atm$?

I've seen the following answer:

$$ W = Fd = \left(\frac{F}{A}\right)Ad = PV $$

where $P$ is the pressure difference. Then plug and chug.

However, this last jump, from identifying $F/A$ with pressure at any point in a given area, to now identifying it with a pressure difference, makes no sense to me. Can anyone explain or suggest an alternate understanding of the problem?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

$F$ is the sum of two forces - the inward force at the start and the smaller inward force at the end. Since they act in opposite directions, it's also the difference in the magnitudes of the forces which directly corresponds to the difference in pressures. Eg if there's 5.0 atm at the start and 4.0 atm at the end, then $$ F = F_{start} + F_{end} $$ $$ =({5.0 \space atm \times A}) + - ({4.0 \space atm \times A}) $$ $$ = \Delta P \times A $$ $$ = 1.0 \space atm \times A $$

Pipe between two pressurized tanks

In the special case where the water is released into a vacuum, then $F_{end} = 0$ and you don't need to use any differences.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I feel like I might be fundamentally misunderstanding the picture that I should have for this. Because all the pressure differences that I've seen until now have been pressure due to elevation, I've been imagining a pipe running vertically. I've been left wondering how pressure is moving the water anywhere. Should I instead be imagining, say, a weightless scenario with water flowing because of, I don't know, a pressure behind it that weakens as it reaches the other end of the pipe? $\endgroup$
    – Addem
    Commented Jan 2, 2016 at 7:17
  • 1
    $\begingroup$ I don't think my answer works in a gravity field. I imagine something like a pressurized tank releasing fluid through a pipe into the air or into another tank with lower pressure. Yes, the pressure weakens along the pipe because there's less and less resistance ahead of it from the pipe walls. $\endgroup$
    – user1318499
    Commented Jan 2, 2016 at 8:45
0
$\begingroup$

The reason there is a pressure difference between the two ends of the pipe is that there is viscous friction acting in the opposite direction at the wall of the pipe. You need to figure out how much work it takes to push the volume V of water into the pipe, and the amount of work the water exiting the pipe does on the water in front of it in exiting the pipe. If P is the pressure at the inlet, the work you need to do in forcing the water into the pipe is $Fd=(PA)\times (V/A)=PV$. Similarly, if $P-\Delta P$ is the pressure at the outlet, the work the water does against the water ahead of it when exiting the pipe is $(P-\Delta P)V$. So the net work done in forcing the water through the pipe is $V\Delta P$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.