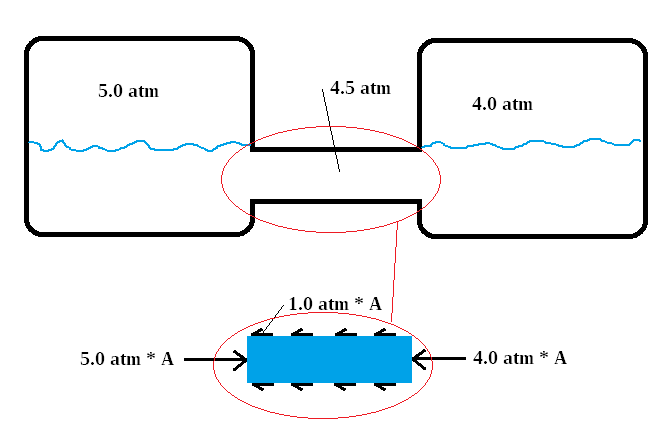
$F$ is the sum of two forces - the inward force at the start and the smaller inward force at the end. Since they act in opposite directions, it's also the difference in the magnitudes of the forces which directly corresponds to the difference in pressures. Eg if there's 5.0 atm at the start and 4.0 atm at the end, then $$ F = F_{start} + F_{end} $$ $$ =({5.0 \space atm \times A}) + - ({4.0 \space atm \times A}) $$ $$ = \Delta P \times A $$ $$ = 1.0 \space atm \times A $$
In the special case where the water is released into a vacuum, then $F_{end} = 0$ and you don't need to use any differences.