We know $$P = F\cdot v$$ where $v$ is the velocity vector. Since at the "displacement nodes" in a standing sound wave the velocity of the particles is always 0, the Power must be 0 and hence the Intensity of sound wave at these positions must also be 0 as $$I =\frac{P}{A}$$
where $A$ is area. But sound intensity is maximum at these points. Can someone explain this to me, please.
The easiest way is to join both the formulas in definition of sound intensity:
$$ i = pv $$
where $p$ and $v$ are acoustical pressure and velocity respectively. In a standing wave there is a $\pi/2$ phase shift between them and hence the intensity maxima out the nodes and antinodes of pressure and velocity.
However, usually you use and measure not the immediate intensity $i$, but it's mean value in time $I$ which is:
$$ I = \frac{p^2_{acoustic \ RMS}}{\rho_0 c_0} $$
It is called a standing wave for a reason.
The reason is that the wave profile does not move along as shown by the blue wave in this animation.
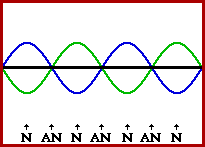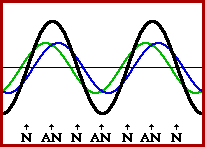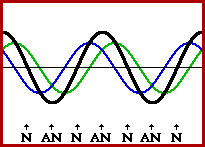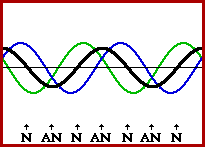
The animation also shows how such a standing wave can be formed by the superposition of two travelling waves of equal amplitude and speed by travelling in opposite directions.
So the net rate of transfer of energy by a standing wave is zero.
This rate of transfer of energy per unit area is the intensity which you have written about in your question and it is zero at all points for a standing wave.
The energy that the wave has is locked in the individual particles which make up the wave and this energy that each particle has is constant oscillating between potential energy and kinetic energy.
A particle at an antinode has the maximum amount of energy and one at a node has no energy.
So if you put a microphone at a node it does not responds as the amplitude of the wave is zero.
If you put a microphone at an antinode the response of the microphone will be the largest passible as this is where the wave oscillation is a maximum.
In sensing the motion of the particles the microphone will remove some of the energy from the standing wave but if that is a small fraction of the total amount of energy stored by the wave at that point then the standing wave is not disturbed very much and the output of the microphone is a measure of the amplitude of motion (energy stored) by the wave at that point or the rate of transfer of energy to the microphone.
That rate of transfer of energy per unit area is the intensity which is picked up by the microphone.
