Landau/Lifschitz's proof of Jacobi's identity

Question

The Poisson brackets of two quantities is defined as

$$[f,g]=\sum_k \Big( \frac{\partial f}{\partial p_k}\frac{\partial g}{ \partial q_k}- \frac{\partial f}{ \partial q_k}\frac{\partial g}{\partial p_k} \Big)$$

The Jacobi identity states that:

$$[f,[g,h]]+[g,[h,f]]+[h,[f,g]]=0$$

In "Mechanics" by Landau and Lifschitz the proof is as follows:

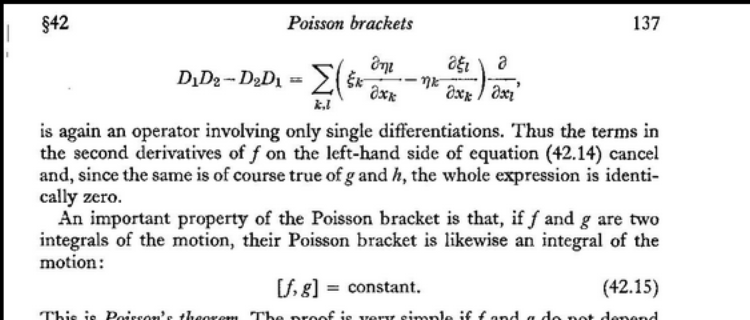
I don't understand the overall argument, they prove that $[g,[h,f]]+[h,[f,g]]$ for a general linear differential operator does not involve the second derivatives of $f$ (I understand that part), but the final part is:

Thus the terms of the second derivative of $f$ on the left-hand side of equation (42.14) cancel and, since the same is of course true for $g$ and $h$ the expression is identically zero

I understand that since $[g,[h,f]]$ is a linear homogeneous function of the derivatives of $f$ and $g$ and we proved that for a general differential operator for the expression of the Poisson brackets there is no second derivative of $f$ in $[g,[h,f]]+[h,[f,g]]$ that means that the terms that doesn't involve second derivative must cancel, but I still don't understand why the terms of $g$ and $h$ should cancel as well.

Answer 1

The argument is supposed to go as follows:

You have shown that $[f,[g,h]]+[g,[h,f]]+[h,[f,g]]$ contains no second derivative of $f$ because $[f,[g,h]]$ doesn't by inspection and $[g,[h,f]]+[h,[f,g]]$ doesn't by the proof presented. By the same logic, $[f,[g,h]]+[g,[h,f]]+[h,[f,g]]$ also does not contain second derivatives of $g$ or $h$.

Inspecting $[f,[g,h]]$, you can see that every summand in it contains at least one second derivative of $f,g$ or $h$. Thus, every summand in $[f,[g,h]]+[g,[h,f]]+[h,[f,g]]$ contains at least one second derivative.

But we know that that expression does not depend on any second derivatives at all. Therefore, all the summands in it must cancel each other, and it is identically zero.