Distinguishing between left-handed and right-handed weak coupling from electron-neutrino scattering

Question

This question comes from Schwartz's QFT book, exercise 13.6. In it we consider a coupling between fermions (neutrinos and electrons in this particular case) and the Z boson of the form $g_V \bar{\psi} \not Z \psi + g_A \bar{\psi} \not Z \gamma^5 \psi$; both the neutrinos and the electrons couple to the Z, so this interaction actually appears twice in the Lagrangian. Then he asks for the cross-section for $e^+ e^- \to \nu \bar{\nu}$, and I'm supposed to compare with the following simulation:

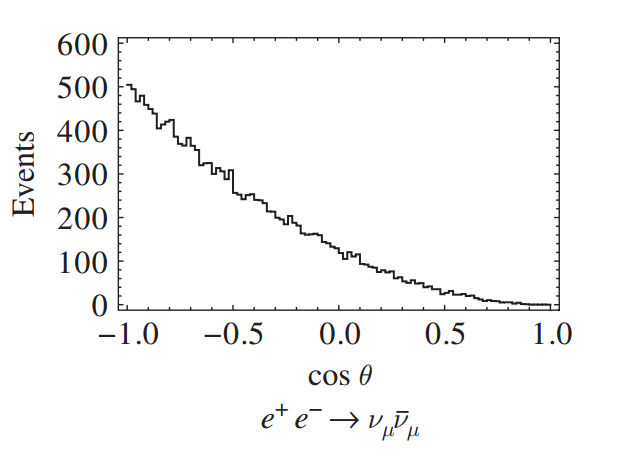
The point is to find some relation between $g_V$ and $g_A$ by analyzing the angular dependence of the cross section. I did that and found, quite predictably, that $g_V^2 = g_A^2$. The book says that this means that $Z$ only interacts with left-handed fields, and as far as I can see there's no way to arrive at that conclusion, because if $g_V=-g_A$ the coupling is left-handed (because of $\gamma^\mu(1-\gamma^5)$), but if $g_V=g_A$ the coupling is right handed.

There's a (very likely) possibility that my math is wrong, but that shouldn't affect the result: each coupling appears an even number of times in the squared amplitude, so there should be no way to distinguish the signs. Is my reasoning correct? Or is there some way to tell L and R coupling apart by looking at this "experiment"?

Answer 1

In real life, the SM, the situation is as follows:

For starters, I flip the definition of the axial coupling w.r.t. yours, so $$g_V \bar{\psi} \not Z \psi - g_A \bar{\psi} \not Z \gamma^5 \psi,$$ just to comport with the mainstream notation (10.2) of the standard PDG2017 review.

By (10.5) of that reference, or, frankly, anyone's notes, in the standard model $$ g^e_V= -1/2+2\sin^2 \theta_W \approx 0; \qquad g^e_A=-1/2;\qquad g^\nu_V=1/2\qquad g^e_A=1/2~. $$ So, your assertion that the e couplings just replicate the ν couplings is alarmingly unsound. While the ν couplings are pure V-A (what else could they be?) those of the electron are, by dramatic contrast, virtually pure Axial, by dint of the famous "accident" of the SM, namely of the weak mixing angle being so close to 30°: a glorious difference! (One always cheers after presenting it in class...)

Edit After having read the actual problem you are describing, I appreciated that the extreme departure from the actual Standard Model is not yours, but in the hypothetical nature of that problem, proffered long before the SM is introduced in that book. Indeed, Matt is considering a vanishing weak angle instead, in that extremely unrealistic hypothetical world.

And, indeed, you are right that, in that fictional case, the cross-section gives you no information on the relative sign of the axial versus vector coupling. The "simulated" result only tells you there is parity violation, as only one handedness is preferred. In fact, this is self-evident in the helicity formalism (recall masses are allowed to be ignorable), where the amp is specified by the rotation matrix $d^1_{11}$, squaring to the cross section in your conventions, $\propto (1-\cos \theta)^2$, for either L or R chiralities !

Still, putting in the above SM values, you may compute the actual cross section, similar to the ones utilized in practice to even pin down the weak angle.