Scattering Amplitudes from Feynman Diagrams (Spinor Helicity Formalism)

Question

$\require{cancel}$ I am trying to do an exercise from Scattering Amplitudes By Elvang (Exercise 2.9) which states:

Show that $A_5(f^-\bar{f}^-\phi\phi\phi) = g^3\frac{[12][34]^2}{[13][14][23][24]} + 3\leftrightarrow 5 + 4\leftrightarrow 5$ in Yukawa theory

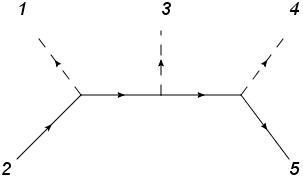
So, I draw the feynman diagram, which I think looks something like this (the interaction term is $L_i = g\phi\psi\bar{\psi}$):

Is this diagram correct? Using the Feynman rules for Yukawa theory (in the Massless Spinor Helicity formalism) I evaluate this to be: $$ A_5(f^-\bar{f}^-\phi\phi\phi) = g^3\langle2|\frac{(\cancel{p_1} + \cancel{p_2})}{(({p_1} + p_2)^2}\frac{(\cancel{p_1} + \cancel{p_2} + \cancel{p_3})}{(p_1 + p_2 + p_3)^2}|5\rangle \\~~~\\+ ~1\leftrightarrow 3 + ~1\leftrightarrow 4 + ~3\leftrightarrow 4 $$

My strategy thus far has been calculate the first term then simply do the permutations at the very end. In general, is this a good strategy to take with diagrams like this?

Doing this, I end up with the following for the first term: $$ A_5^{(1)} = g^3\langle2|\frac{s_{13}}{s_{12}(s_{12} + s_{13} + s_{23})}|5\rangle $$

Where $s_{ij} = -(p_i + p_j)^2 = 2p_i\cdot p_j$ and I have used the Weyl equation $\langle 2|p_2 = 0$.

I can go further, using the fact that $s_{ij} = \langle ij\rangle[ij]$, to end up with: $$ A_5^{(1)} = g^3\langle2|\frac{\langle 13\rangle[13]}{\langle 12\rangle[12](\langle 12\rangle[12] + \langle 13\rangle[13] + \langle 23\rangle[23])}|5\rangle $$

I can't seem to simplify this further. Am I going the right away about solving this? Are there any tricks I am missing?

Answer 1

I won't give you the whole solution, just some pointers which will hopefully help you get there.

1. Label your particles correctly to agree with the question. In this case, they've labelled $f^- = 1$, $\bar{f}^- = 2$, $\phi = 3,4,5$. You need to redraw your diagram with these conventions.

2. You are correct about the permutations. In general it is a good strategy to focus on one diagram and just include the permutations at the end, in the form $(3 \leftrightarrow 5) + (4\leftrightarrow 5)$. Once you've relabelled your particles correctly, this should be obvious.

3. Looking at the first term, you need to be more careful with your spinor-helicity variables. Remember that $\not{p} = -|p] \langle p| - |p \rangle [p |$ so in particular $\langle a| \not{p}\not{q}\ | b \rangle$ does not equal $\langle a \ b \rangle p\cdot q$ as you claim.

4. Once you've done the spinor multiplication correctly in the numerator of the first term, you should have the sum of several terms. I wouldn't be surprised if you need a Schouten identity to simplify it to the form they quote.

Let me know how that goes - I can provide more details if you get stuck. Good luck!

Update: More on Point #3

$$\langle a| \not{p}\not{q}\ | b \rangle = \langle a \ p \rangle[p \ q] \langle q \ b \rangle$$

where we have used

$$p^{\dot a b}=-|p\rangle^{\dot a}[p|^b$$

and a similar formula for $q$.

Note in particular that this is not equal to

$$\langle a \ b \rangle \langle p\ q \rangle [p \ q]$$

in general.