0
$\begingroup$

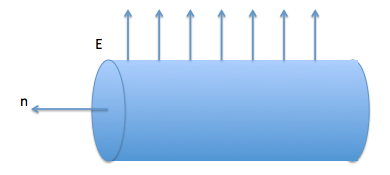
I came across a sentence which made no sense to me as I was studying the electric field of an infinite line charge:

The flux through the FLAT ENDS of our cylindrical Gaussian surface is zero because the radial electric field is parallel to these ends, and so $\vec E\cdot \vec n=0$.

What I don't understand is how can take radial electric field be parallel to the flat ends?(I cannot seem to form the mental picture) And haven't we already established that the radial electric field emenating from the line wire is radially outwards and PERPENDICULAR to the cylindrical surface? Thanks in advance. Sincerely, confused student.

Improve this question
$\endgroup$
1
  • $\begingroup$ Comment to the question (v2): It seems that OP mistakenly believes that we physically have removed the parts of the infinite wire which stick out of the imagined finite Gauss cylinder. $\endgroup$
    – Qmechanic ♦
    Commented Oct 1, 2015 at 14:46

2 Answers 2

Reset to default
4
$\begingroup$

The flat ends of a cylinder are perpendicular to its cylindrical surface. The electrical field is perpendicular to any cylindrical surface centered on the line charge, and so is parallel to the ends of any such cylinder.

$\vec E$ is the electric field, and I presume $\vec n$ is the surface normal of the ends of the Gaussian surface. Since the two vectors are perpendicular, their dot product $\vec E\cdot \vec n$ is zero.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Sorry but could you tell me why would that make E.n = 0 though ? $\endgroup$
    – H1629
    Commented Oct 1, 2015 at 13:31
2
$\begingroup$

I believe the following picture explains what's missing:

enter image description here

The cylinder is "infinite", but the Gaussian surface that is drawn as part of the analysis is in the shape of a finite cylinder with flat ends. And since the electric field is at every point perpendicular to the wire, it is parallel to these flat ends. Parallel to the surface means perpendicular to the normal to the surface - and the dot product is zero for two vectors that are perpendicular.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ The "flat ends" are of a Gaussian pillbox which is much smaller than the line charge is. $\endgroup$
    – CR Drost
    Commented Oct 1, 2015 at 13:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.