I was just studying something about The Plateau-Rayleigh Instability that I saw something called Growth rate of the instability.
My question is the exact physical definition of growth rate of instability in this field (Plateau-Rayleigh Instability) if you put a picture, I'll be more appreciated.
The growth rate of the instability is the characteristic rate $r$ at which a pertubation grows in an unstable dynamic system.
A good reference on this type of instabilities is:
Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves by Pierre-Gilles de Gennes, Francoise Brochard-Wyart, David Quere ISBN 0-387-00592-7
On page 120, it describes a Plateau-Rayleigh instability as occurs on a fibre with an initially uniform film of liquid on its surface. What follows is a summary of that analysis:
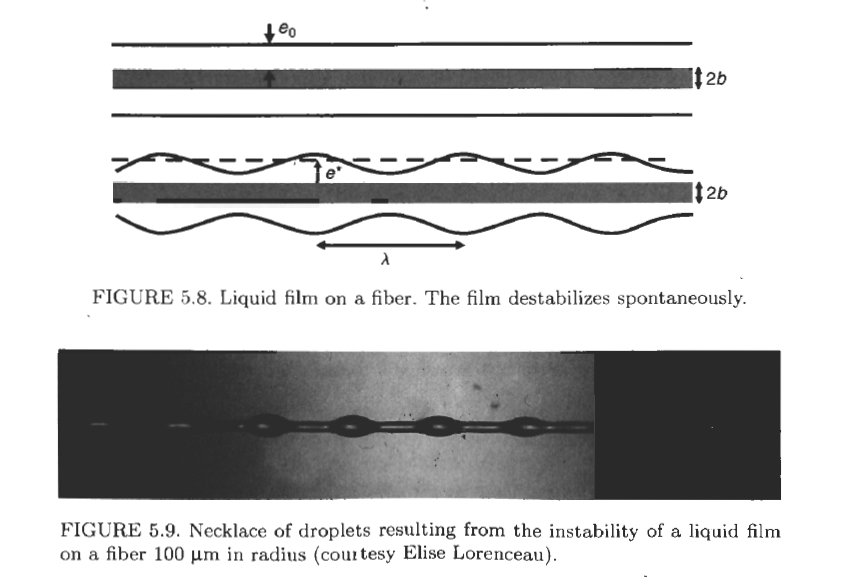
In the picture, $e$ is a film thickness at some time given by: $$ e = e_0 + \delta\left(t\right)\cos\left(\kappa x\right)$$ where $e_0$ is the initial film thickness, $\kappa=2\pi/\lambda$ is the wavenumber and $\delta$ is a time-dependent pertubation. The evolution of the pertubation is found to be: $$\frac{d\delta}{dt} = \frac{\gamma e_0^3}{3\eta b^2}\kappa^2\left[1-\left(\kappa b\right)^2\right]\delta = r\delta$$
where $\gamma$, $\eta$ and $b$ are surface tension, viscosity, and length scale of the surface (see picture) respectively.
For the pertubation to grow (i.e. $d_t\delta>0$), we require that $\kappa b<1$ which is the case when the initial film thickness is much smaller than the characteristic length scale of the surface ($\kappa b$ is a ratio of length scales). As mentioned by Bernhard in the comments, the pertubation then grows as $\exp\left(rt\right)$ with a characteristic rate for a particular wavelength: $$r = \frac{\gamma e_0^3}{3\eta b^2}\kappa^2\left[1-\left(\kappa b\right)^2\right]$$
The smallest wavelength in the system is $\lambda=2\pi\sqrt{2}b$, which yields the smallest (characteristic) time scale: $$r \approx \frac{1}{12}\frac{\gamma e_{0}^{3}}{\eta b^{4}}$$
Which shows that the length scale of the system and the initial thickness have a large impact on the growth rate.
