I'm studying the book Polarimetric Radar Imaging: From Basics To Applications and on page 31, there's this sentence:
In the following, we shall consider the propagation of an electromagnetic wave in a linear medium (free of saturation and hysteresis), free of sources. These hypotheses impose the conditions $\vec M(\vec r,t)=\vec P(\vec r,t)=\vec 0$ and $\vec J_a(\vec r,t)=\vec 0$
This implies that a linear medium is free of saturation. Then what's the meaning of saturation here? And how do we represent saturation or being free of saturation in mathematical sense and notation?
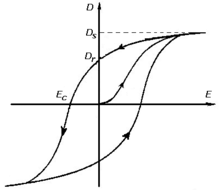
This also implies that a linear medium is free of hysteresis. What's the meaning of hysteresis? How do we represent hysteresis or being free of hysteresis in mathematical notation?
A linear medium is free of source and I think the mathematical notation for that will be $\vec J_a(\vec r,t)=\vec 0$. won't it? (Regarding the meaning of source here is the source to create electromagnetic field or electrical current)
In another Persian source, I have read that:
In a linear medium, if we have the value of one of the quantities, electromagnetic field intensity (H) or electromagnetic induction (B),the value of the unknown quantity can be computed in term of the value of known one
Would you explain the exact meaning of a linear medium in electromagnetism?
P.S.
In a ferromagnetic material saturation state occurs when all of the microscopic dipoles get the same direction and sense of the external electromagnetic field. So if the material or medium is free of saturation, that means these dipoles should neutralize each other and so $\vec P(\vec r,t)=\vec 0$, $\vec P$ is the polarization vector.
In a ferromagnetic material a portion of the microscopic dipoles remain in the same direction after removing the external electromagnetic field and cause the material to magnetize, the remaining magnetism in this way is called magnetic hysteresis. So if the material or the medium is free of hysteresis, that means that no magnetism remains after removing the external field and so $\vec M(\vec r,t)=\vec 0$, $\vec M$ is the magnetization vector.
That was an intuitive explanation for myself, please tell me what's the exact meaning and mathematical notation?