In terms of a dielectric, it means there is a linear constitutive relation between the vectors.
$${\boldsymbol D} = \epsilon_0 {\boldsymbol E} + {\boldsymbol P}$$
Or
$${\boldsymbol D} = \epsilon_r \epsilon_0 {\boldsymbol E}$$
where $\epsilon_r \epsilon_0$ is a scalar relative and vacuum permitivitty. This way, there is now a linear relation between the applied field and the electric displacement. There are certainly situations where
$$D_j = \epsilon_{ij} E_i$$
for some rank two tensor $\epsilon_{ij}$, ie it is more favorable to apply a field along one direction and get a response along another -- re: anisotropy. If a dielectric material is free of saturation, then you can apply as much of a field as you want and get a corresponding electric displacement.
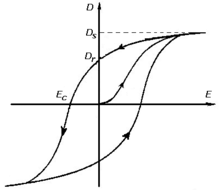
A hysteresis loop in a ferroelectric for example, does not allow this since there is a saturation for large field, so the mathematical way of expressing a dielectric material without a hysteresis is exactly the linear relation above for the electric displacement(and corresponding polarization). From Wiki:
In your first quote here, the author says the material is free of sources, which includes the bound charge source $\rho_b$ that arises $-\nabla \cdot{\boldsymbol P} = \rho_b \Rightarrow {\boldsymbol P} = 0$ as in the above comment. Think of a dielectric sphere in an external field problem that can be solved with separation of variables. There exists $\mathbf{no}$ bound charges within the material so the field inside is aligned with the external field and linearly increases with it.
You are also right that no polarization or magnetismmagnetization should remain when the applied fields are removed if$\mathbf{if}$ the material is free of hysteresis (see above hysteresis plot for zero ${\boldsymbol E}$${\boldsymbol E} \Rightarrow {\boldsymbol D} \neq 0 $). Please let me know if I can clarify or expand anything else in the comments.