Is it possible to explain what actually causes the force on a current carrying conductor in a magnetic field. I have read that this is due to the magnetic fields 'interacting' in some way.
This diagram encapsulates the idea
Apparently the force results from the field lines wanting to 'snap back'. Is this correct? Is there a way to explain the physics behind why the concentration of field lines results in a force experienced.
No, one cannot explain the "cause" any deeper than the explanation that Lorentz force and Maxwell equations are postulated as a description and experimentally are found to foretell correct results. One can give certain motivations why these might be the correct equations: for example, the Lorentz force law is one of the simplest Lorenz covariant laws one can write to calculate a force (it is given by the tensor equation $f_\mu = q\,F_{\mu\,\nu} \,v^{\nu}$ which is Lorentz covariant and $F$ is a tensor field describing electromagnetism, $v$ the four-velocity of a particle of charge $q$ and $f$ the 4-force).
However, there is an approach to the calculation which is vaguely like what you seek for currents and this is the method of virtual work; look this up in connexion with electrostatitcs and magnetostatics: it only applies in the low frequency limit and also only to steady state charge flows, i.e. steady state currents. In this method, you work out the total energies contained in the magnetostatic field with a conductor in two positions, infinitessimally displaced from one another. The force component / torque in a given direction is then equal to the derivative of this energy with respect to a linear displacement parameter for translation / rotation defined by this direction. In this method, to change a configuration that "squeezes" field lines together is equivalent to raising the magnetic energy density of the field, and thus requires the input of work.
As user7027 says, a current also feels a force in a uniform field, illustrating that it is very tricky to use virtual work for driven currents. In this case an outside current source is needed to keep the current constant against the back EMF that is generated by the currents motion across the field lines, so you need to include the outside source in a putative virtual work calculation. Virtual work methods are most useful for passive systems comprising intrinsic material magnetization and/ or undriven ring currents.
What is the cause of the Lorentz Force?
The "screw" nature of electromagnetism. Minkowski referred to it in Space and Time, as did Maxwell in On Physical Lines of Force: "a motion of translation along an axis cannot produce a rotation about that axis unless it meets with some special mechanism, like that of a screw". This is why the right hand rule applies to both electromagnetism and to screw threads. IMHO to really "get" this, you have to take note of Jackson's Classical Electrodynamics: "one should properly speak of the electromagnetic field Fμν rather than E or B separately". Then you need to depict Fμν for an electron. One simplistic way to do it is to combine the radial electric field lines with concentric magnetic field lines like this:
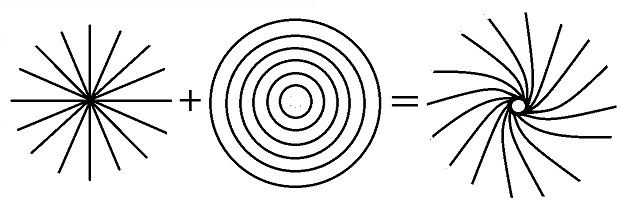
It's simplistic, but now you start to appreciate the electron's "spinor" nature. And if you've taken note of Maxwell's page title, you may appreciate that counter-rotating vortices attract and co-rotating vortices repel. Whilst an electron doesn't involve some fluid motion, there is the Poynting vector and a "circulating energy flow", so the analogy works. As a result if you set down an electron near a positron they will move towards one another in a straight line. But if you throw the electron past the positron they will also move around each other, something like this:
This is what we see in positronium, and now the Lorentz force $\mathbf{F} = q\left[\mathbf{E} + (\mathbf{v} \times \mathbf{B})\right]$ looks obvious. It's just a combination of the linear and rotational force that results from "spinor" electromagnetic field interactions. And it fits with QED in that the electron and positron are "exchanging field". Positronium is like hydrogen but lighter and short-lived, and as you know hydrogen doesn't have much in the way of a field$^*$.
Is it possible to explain what actually causes the force on a current-carrying conductor in a magnetic field?
Yes. You can understand the linear and rotational forces between charged particles easily enough. The next step is to understand the rotational force on a charged particle near a current-carrying conductor. That's essentially a stationary column of metal ions and a moving column of electrons. Ever read Einstein talking about a field as a state of space? OK, see the gravitomagnetic field, which is described as "twisted space" by NASA author Tony Phillips? You can think of the electromagnetic field as something similar but a tad more intense. Only if you had motion relative to it, you might think of it as "turning space" and start talking about curl aka rot which is short for rotor. IMHO this is the key to really understanding how magnets work. The electrons all have a negative electromagnetic field, and the metal ions all have a positive electromagnetic field with the opposite chirality. If the electrons weren't moving, the opposite "twist fields" would just about cancel each other out$^*$. However the electrons are moving up the wire:
So it's like you're moving through one set of twist fields but not the other. And when you have motion relative to a twist field, you think of it as a turn field, and that's what a magnetic field is. So what you "see" is a residual turn field, a magnetic field, around the wire. An electron thrown past the wire moves in a circular fashion not because of some magical action-at-a-distance force, but because it's "a dynamical spinor in frame-dragged space".
The last step is to understand why two wires move together. For this you can think of your electron being confined in the adjacent wire. It moves upwards, and it moves in a circular fashion. This rotation means the residual turn field looks like a twist field, and since the rotations are counter-rotations to the left and right, you're again in a situation where counter-rotating vortices attract. There's a net linear attraction between the two wires. For the catapult, bend one of the wires into a loop to make a primitive solenoid, then into multiple loops for a better solenoid, which is akin to a bar magnet. Then bend it into a horseshoe shape and put the other wire between the poles like this:
Image courtesy of SPM physics
Again, it moves.
$*$ There is a residual field, but we don't call it an electric field or a magnetic field. Or an electromagnetic field. Or a gravitomagnetic field.
Apart from philosophical debates: What is the cause of any force? A gradient in the energy. I'm not in the mood to do any actual calculation, but the energy density of a magnetic field is $\sim \vec{H} \cdot \vec B \sim B^2$ (here at least). Now, we are looking at a field that is created by superposition $\vec B = \vec B_1 + \vec B_2$ with $\vec{B}_i$ being the field around wire $i$. For sufficiently large distance of the two contributions will be virtually zero, but if they are close enough than you have to compute the vectorial sum the value of which depends on two things: a) the distance (creating along the connection vector) and b) the signs of the two currents (determining the sign of the force, $(a+b)^2\neq (a-b)^2$).
Yes, it is possible to explain it. The reason are the magnetic dipole moments of electrons and its intrinsic spin. More see my explanations in this paper.
Electrons have magnetic dipole moment and intrinsic spin. (This spin is really a rotation due to the Einstein-de Haas experiment.) When moving electrons came into a non-parallel to the electrons movement magnetic field the electron's magnetic dipole moment get aligned. This aligne the spin too.
Due to electron's gyroscopic effect the wire get a moment and get deflected sideways. Perhaps the full process is more complicated due to photon emission (electromagnetic radiation of the wire) and spin disalignement and due to permanent disruption of the electron's movement from the atomic structur of the wire.
