Why would the norm of these vectors be 1?

Question

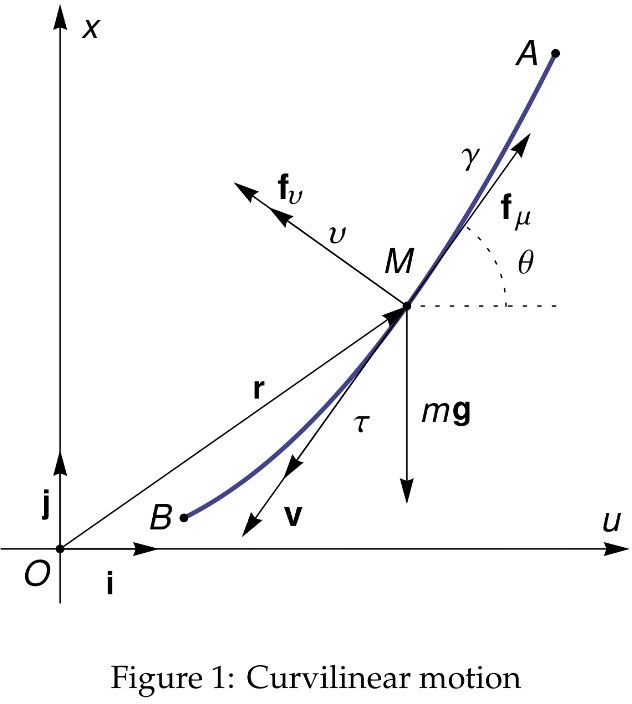
Let a Cartesian coordinate system $uOx$ coincides with a vertical plane so that $Ou$ is the horizontal axis and $Ox$ is the axis oriented vertically upwards (see Fig. 1). We are looking for the smooth curves connecting two fixed points $A = (u_0, x_0)$ and $B = (u_1, x_1)$ with $u_0, u_1 \geq 0$, $u_0 \neq u_1$, and $0 \leq x_1 < x_0$ so that a bead $M$ sliding with initial speed $v_0 \geq 0$ downward from $A$ and accelerated by gravity will slip with a nonlinear kinetic friction to $B$ in the least time $T$.

We suppose for the beginning that there exists a solution represented by a sufficiently smooth curve $\gamma$ and an arbitrary point $M$ lying on $\gamma$. Let $\mathbf{\tau}$ be the unit tangent vector to $\gamma$ and $M$, $\mathbf{v}$ be the velocity vector of the bead $M$, $\mathbf{\nu}$ be the unit normal vector to $\gamma$ at $M$, $\mathbf{g}$ be the acceleration gravity vector, $\mathbf{f}_\mu$ be the friction force, $\mathbf{f}_\nu$ be the normal component of the constraint reaction force, $\theta$ be the slope angle of the tangent, and $\mathbf{i}$ and $\mathbf{j}$ be the unit vectors of the Cartesian coordinate system $uOx$.

The position of a particle $M$ relative to the coordinate system $uOx$ is determined by the position vector $\mathbf{r}$ (see Fig. 1). The particle $M$ is moving from $A$ to $B$, so its position vector $\mathbf{r}$ is a function of time $t$, i.e.,....

Hi, I am having a lot of problems understanding this excerpt. The quote is an introduction, and later on they said that $\lVert\mathbf{\tau}\rVert=\lVert\mathbf{\nu}\rVert=\lVert\mathbf i\rVert=\lVert\mathbf j\rVert=1$. Why? Also, why is the velocity vector $\mathbf v=-v \cos\theta\ \mathbf i -v\sin\theta\ \mathbf j$?

Answer 1

The introductory quote defines $\tau$ and $\nu$ to be unit vectors. That means that their magnitude is 1. $\mathbb{i}$ and $\mathbb{j}$ are unit vectors along $x$ and $y$ axes. That's a standard notation. Some of the comments are stressing the fact that the basic understanding of vectors is missing. I have a feeling that you've jumped into a 'complicated' vector resolution problem directly without doing some basic exercises.

That is the reason for your difficulty in understanding $\mathbf{v}$. First, read this page on how to resolve vectors. It's simple, but you've to be sure of what you're doing. Then, read this pdf on circular motion. Notice there how $\hat{r}$ is written. I believe, you will get your answer.

A note: if you extend the dotted line, towards left of $M$, notice that the vector $\mathbf{v}$ makes an angle $\theta$ with it on the left side. Use this along with resolution of vectors, and you'll have your answer.

P.S. This is not an answer per se. There's no point in answering the question, without the OP understanding the general process.