What is the physical reason that the undamped driven oscillator has mean power zero?

Question

The instantaneous power absorbed by an undamped driven oscillator is given by:$$\mathbf{P} =-\omega\dfrac{F_o/m}{{\omega_0}^2 -{\omega}^2} F_0 \sin{\omega t}\cos{\omega t}$$. But my book says the mean power of an undamped driven oscillator is zero. What is the physical reason behind this? Can I infer mathematically the average power to be zero from instantaneous power?

Also can anyone help me visualise what the following para is saying?

The power input, being proportional to $\sin{2\omega t}$ , is positive half the time & negative for the other half, averageing out to be zero over any integral number of half-periods of oscillation. That is, energy is fed into the system during one quarter-cycle & is taken out again during the next-quarter cycle.

First, half-cycle is mentioned & then quarter-cycle is mentioned . What is it all about? Can anyone help me visualise what has been mentioned here?

Answer 1

You know that $2 \sin(\omega t) \cos(\omega t) = \sin(2\omega t)$ right?

So it is clear that the instantaneous power can be written as $$P = -\dfrac{1}{2}\omega\dfrac{F_o/m}{{\omega_0}^2 -{\omega}^2} F_0 \sin{2\omega t}$$

As your textbook says, this is symmetric around zero - it spends as much time being positive as it does being negative. When you integrate this over a full cycle of the oscillator, the total power would be zero. Since the definition of the average power would be this integral divided by the cycle period, then the average power must also be zero.

Now the period of the function $\sin (2\omega t)$ is half that of $\sin (\omega t)$. When your book talks about the the period of oscillation, it is talking about the latter, which equals $2\pi/\omega .$

However, during that oscillator-period, the function $\sin (2\omega t)$ goes through two cycles. It is therefore positive for quarter of an oscillator-period; then negative for quarter of a period, then positive and then negative.

The plot below shows the situation for an assumed $\omega =1$. I've shown a sine function, a cosine function and the product of the two (which represents the instantaneous power). I also have shown the period of the oscillator. Note that the instantaneous power goes through two full cycles during one period of the oscillator.

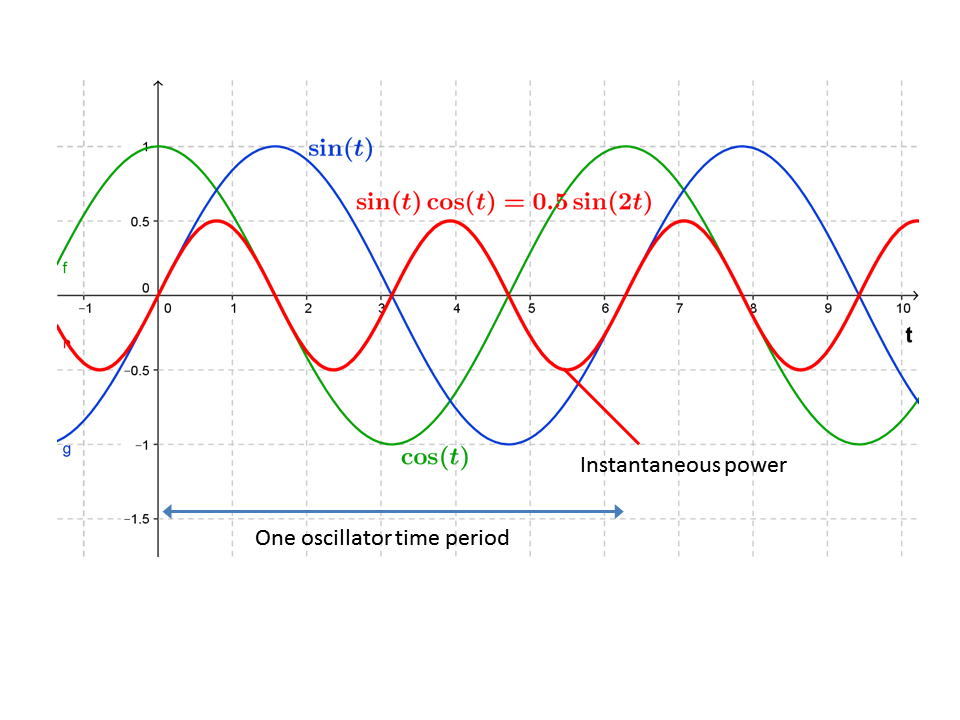
In terms of physics, what is going on is that the force is being applied $\pi/2$ out of phase to the velocity of the oscillation. The total energy of the oscillator (shared between kinetic and potential energy) is not changing; and because there is no damping, energy is not being lost either. Therefore on average no power is expended. Instantaneously the situation is more complex. Work must be done to stretch the spring for quarter of a cycle (assuming it is a spring); but then in an undamped system, all that potential energy is recovered as the spring comes back towards the equilibrium position and turned back into kinetic energy. And then repeated on the other side of the equilibrium position to complete one full oscillator-period.

Answer 2

$<P> = \lim_{\tau \to \infty} \frac{1}{\tau} \int_{0}^{\tau} P(t) dt = 0$

you also can take mean value in one period by take $\tau \to T$ where $T = \frac{2\pi}{2\omega}$ is the period of the oscillator.