Why does the apparent size of an object change when it is farther away from me compared to when it is closer to me?
Can someone perhaps explain this through an optics perspective?
$\begingroup$
$\endgroup$
2
asked Jun 6, 2015 at 21:00
-
1$\begingroup$ Because the 'size' we see is the solid angle subtended by the body at our eye, and that's inversely proportional to the square of the distance. $\endgroup$– Anurag BaundwalCommented May 15, 2018 at 4:58
-
2$\begingroup$ It's because your eye is curved. If your eye was flat, there would be no distortion, but your field of view would be very small. Evolution decided that was less useful. $\endgroup$– yarCommented Feb 11, 2022 at 10:33
Add a comment
|
6 Answers
$\begingroup$
$\endgroup$
Apparent size is not measured as an ordinary size, in meters. It is actually an angle, so it is measured in degrees or radians.
See this picture:
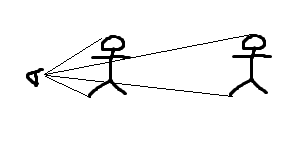
The object on the left is the eye. Looks like as the object moves further, the angle becomes smaller. That is what is called perspective.
Sometimes people try to compare apparent size (solid angle) and real size, but that makes no sense because they have different dimensions. For example, I've been asked:
Is the Moon bigger or smaller than a 1€ coin?
The answer is that it is much, much bigger: about 3000 km vs 2 cm. What the question is trying to ask is compare the apparent size of the Moon with the real size of a coin, and that makes no sense. You should compare the apparent size of the Moon with the apparent size of the coin, but then you should say what distance the coin is.
For reference, the Moon apparent size is about half a degree. That is about the size of your thumbnail, with the arm extended. It does not matter if your hand is big or small because a big hand will also mean a big arm!
$\begingroup$
$\endgroup$
1
Another way perspective on it is that we have only single a points of observation (i.e our eye only has 1 observation point) for which observe objects. This means that when we observe an object, the visual angle will be smaller for objects which are further (if their sizes are the same).
This is similar to mapping a point (our observation point), to any object on a 2D plane [However our eyes only have a set ranged angle for vision]
In some 3D modeling software you are able to change the perspective of the camera such that it is no longer a reference point, but rather more-so a plane (perspective to orthographic). In this case objects appear the same size regardless of distance since their lines will always be perpendicular (same angle) for every/any object in their view.
-
$\begingroup$ I like this answer best, because your point about orthographic perspective got to the heart of my confusion, and led me to the wikipedia article on en.wikipedia.org/wiki/Parallel_projection Another way of saying it is that far-away things appear small because we view them through a finite lens. Further, their apparent smallness is in proportion to the smallness of your lens (your eye). In other words, the difference between how big that far-off mountain actually is - and how big it appears - would be less dramatic if you were also as big as the mountain. $\endgroup$– yarCommented Feb 11, 2022 at 10:06
$\begingroup$
$\endgroup$
The size of an object while seeing it through eyes depends on the visual angle and not on the real size of the object. If the object is near to the eyes the visual angle will be higher and when it is far away the angle will be smaller. According to that, the size will differ. The size is proportional to the visual angle.
answered Jan 18, 2016 at 17:37
$\begingroup$
$\endgroup$
3
You could also understand why objects seem smaller from a certain distance and further and why they look bigger when they are to close as this:
The retina has in humans a mean distance from the lens of in the eye about 0.02 metres. Thus, from simple trigonometry, we have:$$ {O \over P} = {I \over Q}$$ $$ I=({Q \over P}) O$$, where O is the real size of the object, I is the size of the idol at the lens, P is the distance between the object and the observer and Q is the distance of the idol, that is the 0.02 m we mentioned before.
Thus, the distance where the object seems to the observer to its real size is where the O is equal to I, that is $P=Q=0,02m $
Hope this helps.
answered Jun 12, 2015 at 18:02
-
-
$\begingroup$ @JosephGarvin I am not sure if image is the correct word to explain it. $\endgroup$ Commented Apr 10, 2020 at 7:57
-
$\begingroup$ So you're just saying "things look their actual size when they're 2 centimeters from your face"? $\endgroup$– yarCommented Feb 11, 2022 at 9:55
$\begingroup$
$\endgroup$
You could also think about the perception of this in terms of spheres of space.
Let's say you are in a very small room which is hemispherical in shape. There is a 6-foot high door in the wall which seems to be very big - and relatively, it is - it takes up about 20% of the overall area of the wall.
Now imagine a much bigger hemispherical room that you are standing in the centre of. The door in the wall - further away now - is the same actual height, but it now takes up a much smaller percentage of the total space - perhaps 2% of the area of the wall.
$\begingroup$
$\endgroup$
Objects at a distance appear smaller because the visual angle they subtend becomes more acute with distance. The visual angle may be thought of as a triangle with the apex at the eye, and the distant object as its base.
The apparent height of an object is directly proportional to its actual height and inversely proportional to its distance from the eye. Apparent Height = Actual Height / Distance. So to find the actual height of a distant object, multiply its apparent height by its distance. Conversely, you can divide the known actual height of a distant object by its measured apparent height to arrive at the distance.
There is another geometrical distance relationship called the Inverse Square Law. This applies to all qualities projected by a distant object, including light bouncing off of its surface. Application of this law explains why a distant object may appear fainter than a near object: http://hyperphysics.phy-astr.gsu.edu/hbase/forces/isq.html.
