1
$\begingroup$

How can I measure a length of over 4 feet to an accuracy of 1/100th of an inch ?

I want to make several metal standard bars of a particular lengths, some of which are over 4 feet and I want the length accuracy to be within 1/100th of an inch ? How can I do this?

I have standard machinist tools (surface plate, precision level, micrometers, Grade 25 balls, gauge block set, pin gauge set, height gauge, etc.)

Improve this question
$\endgroup$
10
  • 3
    $\begingroup$ This question might be a better fit on engineering. Even better would probably be a Machinist's stack, if there was such a thing. $\endgroup$
    – The Photon
    Commented May 23, 2015 at 1:40
  • 1
    $\begingroup$ @TylerDurden when you machine steel it heats up; you want accuracy of 0.01 in 48 or 1 part in 5000. Coefficient of thermal expansion is about 1 part in 100,000 /K - so if the bar heats by 20 K it would use up all your tolerance. When you cut steel it can easily get much hotter. Worth keeping in mind, at least. $\endgroup$
    – Floris
    Commented May 23, 2015 at 4:00
  • 1
    $\begingroup$ What is the longest bar you can measure with your available tools? How big is your micrometer? If you can grind a 6" bar to a precision of better than 1 thou, a stack of 8 of those will measure 4 feet with better that 1/100th. $\endgroup$
    – Floris
    Commented May 23, 2015 at 4:03
  • 1
    $\begingroup$ Unless you tell the experienced metrologist exactly what tools you have available I am not sure anyone can help. Clearly you are missing the tool to measure this length directly, right? 1/100th inch is 0.25 mm - it ought not to be hard with the right tool. What is the greatest length you can measure? $\endgroup$
    – Floris
    Commented May 23, 2015 at 4:39
  • 1
    $\begingroup$ @Photon The first problem of physics is to measure the physical world. $\endgroup$
    – Ambrose Swasey
    Commented May 23, 2015 at 16:11

1 Answer 1

Reset to default
2
$\begingroup$

After some significant research, I have figured out how to do this. You make a linear measurement array using precision 1.0000" balls in right angle v-groove with a base plate, and then a matching block at the top. If this is done carefully, you can even reach 1/1000th precision. It looks like this:

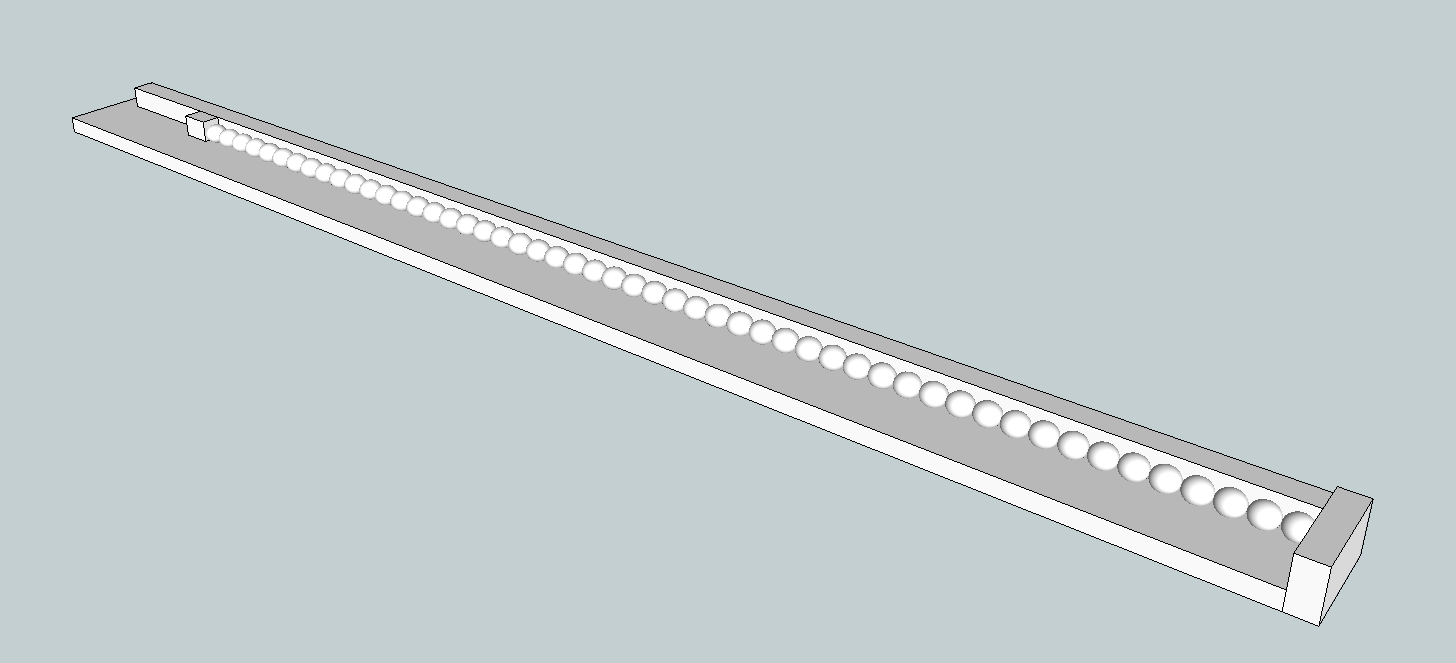
linear measurement array

Everything has to be scraped and square, especially the foot plate. You will need to pin this and set it up very carefully to ensure right angles. The base plate is inclined so the balls rest by gravity. The array is sized out at an inch height.

The block at the top is used to finish the measurement. For example, if you want a standard length of, say, 53.75 inches, then you use 52 balls and you make a 1.750" block. If you want hundreths precision, then the block needs to be to the nearest thou all around. If you want thousandths precision, then the block needs to be to the nearest tenth all around.

To make the standard get the bar and scrape it all around square except one end, which is matched to the block. Doing this match is the hard part. If you do not know how to flush two surfaces to a thou, talk to a toolmaker to learn a procedure for it.

As an alternative (expensive) way to do this, which is superior, is that there are long length gauge block sets. The typical set has blocks in the following lengths:

20 + 16 + 12 + 10 + 8 + 7 + 6 + 5 = 85 inches

So, you can measure out lengths up to 7 feet using this kind of gauge block set (or combine multiple sets to go even higher). The problem is that a long-length gauge block set sells for between \$1,500 and \$3,000.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Have you considered making the track for the precision balls out of two lengths of, say, 1" diameter steel rod running parallel and in contact? Not sure how errors in diameter would cause errors in total length, but it's off the shelf and no machining... $\endgroup$
    – DJohnM
    Commented May 23, 2015 at 17:31
  • $\begingroup$ @User58220 That would not be rigid. $\endgroup$
    – Ambrose Swasey
    Commented May 23, 2015 at 22:38
  • 1
    $\begingroup$ Depending on the cost of this setup (including labor if it's a professional job), it might be worth getting a large set of calipers instead. For instance: medfordtools.com/metalworking/extendedCalipers.html $\endgroup$
    – Mark H
    Commented May 26, 2015 at 9:43
  • $\begingroup$ @MarkH That is pretty interesting. I did not know such a thing existed. That would a lot better than a custom setup if they were accurate. $\endgroup$
    – Ambrose Swasey
    Commented May 26, 2015 at 10:35
  • 1
    $\begingroup$ @Floris Right, that is why using long gauge blocks is useful. There is no need to perfectly square three angle plates together. $\endgroup$
    – Ambrose Swasey
    Commented Jun 24, 2015 at 16:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.