3
$\begingroup$

We all know how an electric current causes magnetic fields. But when a single electron is flowing through the wire, how is this electron creating the magnetic field or how are electrons responsible for magnetic field around a wire?

Improve this question
$\endgroup$
1
  • $\begingroup$ The idea of "a single electron flowing through a wire" makes about as much sense as a single molecule of water flowing through a pipe. $\endgroup$
    – Solomon Slow
    Commented May 15, 2015 at 2:27

2 Answers 2

Reset to default
-2
$\begingroup$

I could give to you only an answer to some part of your question. The comment from gonenc points to an answer, there the first sentence is:

If you are not well-acquainted with special relativity, there is no way to truly explain this phenomenon.

There is a simple explanation how accelerated electrons create a magnetic field. It has to do with the intrinsic spin and the related magnetic dipole moment of the electrons. If you are not familiar with these phenomena of electrons please read Wikipedia, search in StackExchange or ask in separate questions.

Now, if you are familiar with the basics please read my explanations about how works the phenomenon of electromagnetic induction.

This is only the first step to answer your question. Now you could imagine how a accelerated charge - for example an electron in a coil - induces a magnetic field. How the magnetic field in a straight wire get induced I have explained in other posts.

But my posts were greeted with scepticism. I've no problem with that. What disturbs me is the general reaction that they are "wrong". My question is summarised as follows: Did any of these people actually follow my explanations and can anyone tell me where a mistake was made? (This sentences are adopted from a question of @G. 't Hooft.)

Improve this answer
$\endgroup$
-2
$\begingroup$

How are electrons responsible for magnetic field around a wire?

Via the "screw" nature of electromagnetism. Minkowski referred to this in Space and Time, as did Maxwell in On Physical Lines of Force: "a motion of translation along an axis cannot produce a rotation about that axis unless it meets with some special mechanism, like that of a screw". This why we have the right-hand rule which applies not just to electromagnetism, but to screw threads. Note section 11.10 of Jackson's Classical Electrodynamics where he says "one should properly speak of the electromagnetic field Fμν rather than E or B separately". Like Christoph said in the possible duplicate, the electron doesn't actually have an electric field, it has an electromagnetic field. And to envisage this field you take a tip from Maxwell's combination of curl and convergence:

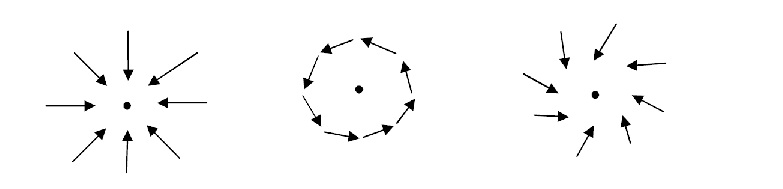
enter image description here

Combine radial electric field lines with concentric magnetic field lines to give electromagnetic field lines like so:

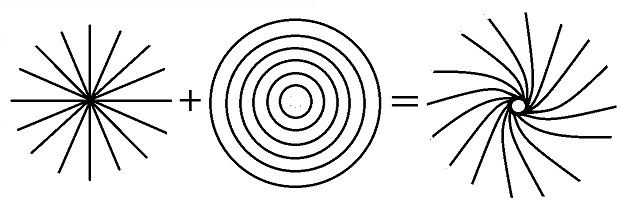
enter image description here

The above is a flat depiction with no spin ½ dipole, but I think it's enough to get across this screw nature of the electromagnetism. It's also reminiscent of depictions of a vector field and a spinor, and something like the gravitomagnetic field, which is described as twisted space by NASA author Tony Phillips. The electromagnetic field isn't totally different, see Heaviside's 1893 paper. IMHO you get the gist of it by imagining you're walking into it with your arms outstretched: think of the electromagnetic field as a "twist" field, wherein if you were moving through it but didn't know it, you might say it was a "turn" field. That's kind of what a magnetic field is. We talk of curl aka rot which is short for rotor, and if you throw an electron through a magnetic field, it goes round in circles.

Anyway, in the current-in-the-wire you have in essence a column of electrons and a column of metal ions. The electrons all have a negative electromagnetic field, and the ions all have a positive electromagnetic field with the opposite chirality. If the electrons weren't moving, the opposite "twist fields" would cancel each other out$^*$. However the electrons are moving up the wire:

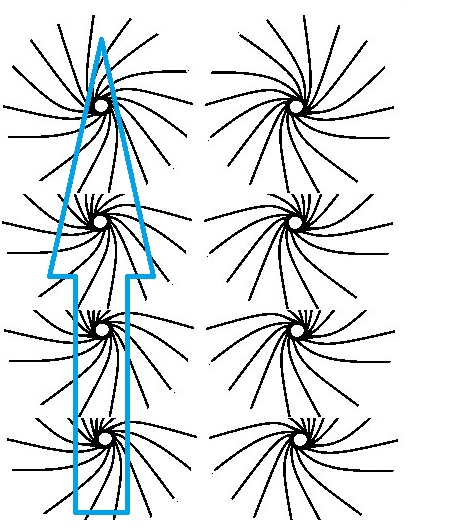
enter image description here

So it's like you're moving through one set of twist fields but not the other. And when you have motion relative to a twist field, you think of it as a turn field, and that's what a magnetic field is. So what you "see" is a magnetic field around the wire.

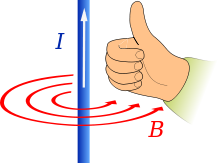enter image description here GNUFDL image by Jfmelero see Wikipedia

$*$ Actually they don't quite cancel each other out, but that's one for another day.

Improve this answer
$\endgroup$
0

Not the answer you're looking for? Browse other questions tagged or ask your own question.