1
$\begingroup$

I have difficulty understanding forces involved in moving magnet and conductor problem.

When a ring conductor is at rest and an ordinary bar magnet is moving, there's electric force. I have trouble understanding how the electric force arise here. Based on my understanding of Faraday's law, there should be induced current flowing in the ring conductor. So the electric force is responsible for creating current?

Here is what I mean by electric force creating current: Consider this example where the magnetic field out the page is moving to the left towards the stationary wire loop

Faraday's law tells me that the current should be flowing clockwise. I am not sure what are forces involved here. There is no magnetic force since the loop is stationary so there's electric force somewhere and I'm not sure where it came from and its direction. According to this equation $\nabla \times \vec{E} = -\frac{\partial \vec{B}}{\partial t}$, it looks like there is electric field flowing clockwise direction like the current. So the clockwise electric field which produces electric force is responsible for creating clockwise current?

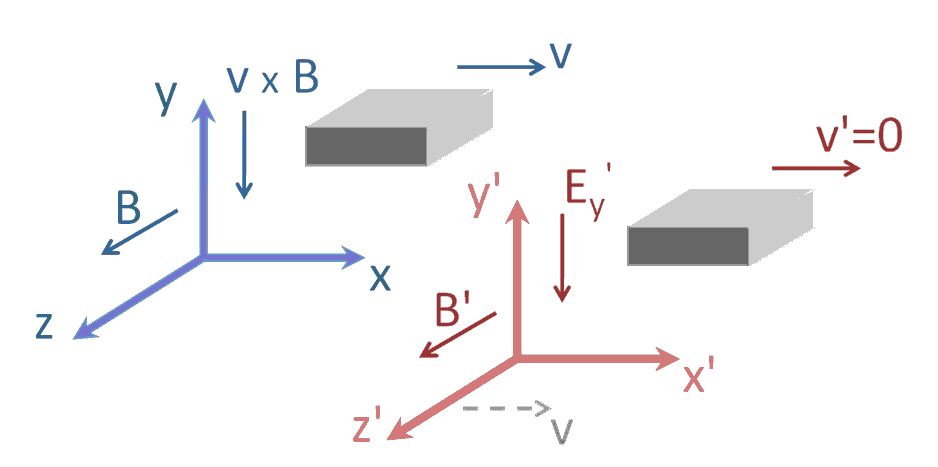
Take a look at this image from this Wikipedia article,

I understand where the magnet force is coming from in the magnet frame (one with blue coordinates). But in the conductor frame (one with primed red coordinates), I have trouble understanding where the electric force came from. I don't know the source of electric field and why it points downwards. Also, I'm not sure why the primed red coordinates is moving in +x direction (see the dotted arrow). It is supposed to move in -x direction?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

According to this equation $\nabla \times E = -\frac{\partial B}{\partial t}$, it looks like there is electric field flowing clockwise direction like the current. So the clockwise electric field which produces electric force is responsible for creating clockwise current?

Yes.

Improve this answer
$\endgroup$
9
  • $\begingroup$ So how do you apply Faraday's law to explain the electric field in the second image? $\endgroup$
    – Jimmy Yang
    Commented Nov 27, 2022 at 9:21
  • $\begingroup$ @JimmyYang I don't understand your confusion, unfortunately. Isn't that the same situation as the first image? $\endgroup$
    – Andrew
    Commented Nov 27, 2022 at 12:08
  • $\begingroup$ There’s a bar conductor in the second image and I have no idea how and in what direction current flows in the bar. $\endgroup$
    – Jimmy Yang
    Commented Nov 27, 2022 at 20:11
  • $\begingroup$ Hmm, consider a situation different from the first image: wire loop is moving to the right to stationary magnetic field. I am told that magnetic force is responsible for creating current but it seems like I can similarly argue using Faraday’s law that electric force is also responsible for creating current? So when the loop moves towards magnetic field, both magnetic and electric force is responsible for creating current in the loop? $\endgroup$
    – Jimmy Yang
    Commented Nov 27, 2022 at 20:16
  • 1
    $\begingroup$ @JimmyYang The time derivative of the magnetic field is zero in the case when the loop is moving toward the magnetic field. Then Maxwell's equation becomes $\nabla \times E = \partial B/\partial t = 0$, so there is no time-dependent magnetic field sourcing the electric field. The fact that $\partial B/\partial t = 0$ is maybe more obvious if you just take away the loop -- obviously the loop being present or not cannot affect the applied magnetic field. $\endgroup$
    – Andrew
    Commented Nov 27, 2022 at 23:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.