How to get the pressure amplitude at any spatial point?

Question

Working on the pressure equation from the linearized euler equations, I stumble across a very simple problem :

How, from the pressure solution of the specific equation (see http://www.acs.psu.edu/drussell/Publications/MDQSources.pdf EQ. 2) such as :

$$ p(r,\theta,t) = i\frac{Q\rho c k}{4\pi R}e^{i(wt - kr)} $$ Which is the pressure response to a harmonic point source. R : Distance between source and receiver, $\rho$ fluid density, $c$ fluid adiabatic velocity, $w = 2\pi f$ wave pulsation, $k = w/c$ wave number, $Q$ a constant, the complex source strength.

Can you get the pressure amplitude against time at any point $\mathbf{x}$ of the physical domain ?

Thanks a lot

Quentin

Answer 1

Well, if I'm not mistaken, it's pretty straightforward. Let $p(r, \theta, t)$ be separated in two functions with variables of time $T$ and spatial variables $\Theta$ (I'm not using $R$, cause it's already defined):

$$ p(r,\theta,t)=\Theta(r,\theta)T(t) $$

then:

$$ T = e^{i\omega t} $$

$$ \Theta = i\frac{Q\rho c k}{4\pi R}e^{-ikr} $$

$T$ is given ("amplitude for given time"), so you only need to calculate $\Theta$.

Now, the only problem is how to distinct $r$ and $R$. If I get you right, you basically want an intensity plot for "test receivers" and in that case $r=R$, therefore:

$$ \Theta = i\frac{Q\rho c k}{4\pi R}e^{-ikR} $$

so you'll get circles of intensity with center in $R=0$.

Is that it?

Answer 2

Solution

As already stated in a comment, there's no dependency on the angle (either $\theta$ for a 2D domain or $\theta$ and $\phi$ for a 3D domain) since the solution has spherical symmetry.

The equation you presented then becomes

$$ p \left( r , t \right) = \frac{j \omega \rho Q}{4 \pi r} e^{j \left(\omega t - k r \right)} \tag{1} \label{pressure} $$

Where, as already stated in another answer, you can separate the radial and temporal components such that

$$ p \left( r, t \right) = \frac{j \omega \rho Q}{4 \pi r} e^{-j k r} \cdot e^{j \omega t} \tag{2} \label{separated} $$

with the first term being dependent only on the radial distance and the second only on time.

A useful observation made in equation \eqref{separated} is that if you fix $r$ then the first term is just a constant. Denoting it with $C$, equation \eqref{separated} becomes

$$ p \left( r, t \right) = C e^{j \omega t} \tag{3} \label{harmonic-oscillator} $$

which resembles a simple harmonic oscillator with amplitude $C$ and temporal frequency $f$ related to the angular frequency by $f = \frac{\omega}{2 \pi}$. Thus, you can see that the solution for a fixed point in space (either 2D or 3D since the solution has the exact same form) is a (co)sine which is the real part of the exponential $e^{j \omega t}$ and the peak amplitude will depend on the distance from the source $r$ and the source strength $Q$.

Initial conditions

As already stated in the comments, you seem to have doubts about the causality of the wave propagation. You are missing the point that the solution of equation \eqref{pressure} is the steady-state solution.

Nevertheless, it would be quite easy to impose the travelling nature of a propagating spherical wave. All you have to do is multiply the solution with a Heaviside function which depends on the time of travel. The Heaviside function would be denoted like

$$ \mathcal{H} \left(x \right) = \begin{cases} 0, & x < 0 \\ 1, & x \geq 0 \end{cases} \tag{4} \label{heaviside} $$

Below is an example graph I created with MATLAB for a sinewave with temporal frequency $f = 50 ~ Hz$ and $Q = 1$. The wave propagation speed is $c = 343 ~ \frac{m}{s}$, the density of the medium is $\rho = 1.21 ~ \frac{kg}{m^{3}}$ and the distance from source is $13 ~ m$. I have used known equations to calculate $\omega = 2 \pi f$ and $k = \frac{\omega}{c}$.

The "analytical" expression I used is

$$ p \left( r, t \right) = \mathcal{H} \left( t - \frac{r}{c} \right) \frac{j \omega \rho Q}{4 \pi r} e^{-j \left(\omega t - k r \right)} $$

where $t - \frac{r}{c}$ is the time the wave propagating with speed $c$ needs to reach the point of observation at distance $r$ from the source.

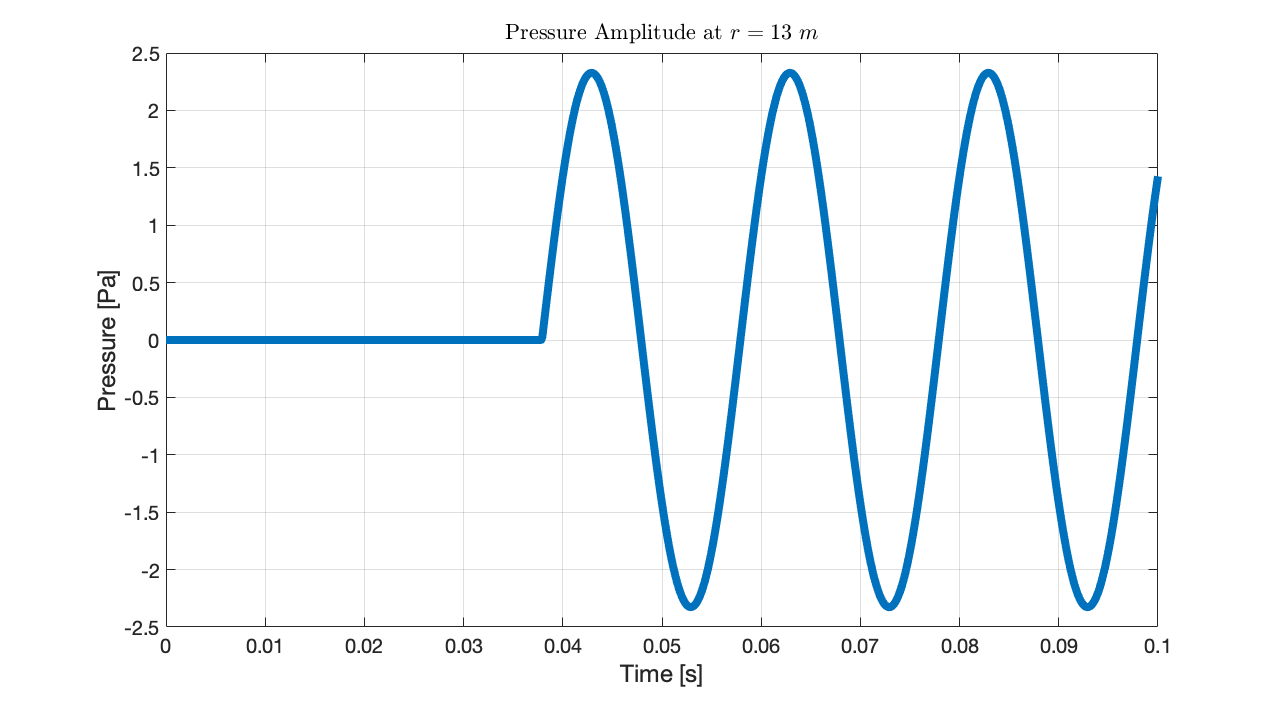
This shows that the causality of the solution is not violated in any way.