How to use Ampere's Law for a semi-infinite wire with current?

Question

Suppose that there is a semi-infinite wire which extends to infinity only in one direction. There are no other circuit elements at the other end(finite end) of the wire and the current does not loop. The magnetic field obviously has cylindrical symmetry when the Amperian contour is taken as a circle with its center on the wire.

However, due to charge accumulation there is a time-dependent electric field; hence a displacement current. How can I formulate the Ampere's law and show that the magnetic field is the half of that the infinite wire at the finite end of the wire? Do you think treating charge accumulation as a point charge with changing amount of charge right at the finite end of the wire will suffice?

The original question is:

Answer 1

Ampere's law (for a steady current) states that $$ \oint \vec{B} \cdot d\vec{l} = \mu_0 I$$

If we consider an infinite wire, then symmetry tells us that the B-field at the point $A$ and all other points on a circle of radius $(R+y)$ is constant in magnitude and is in the azimuthal direction. Hence the magnitude of the B-field is given by $$ 2 \pi (R+y)B = \mu_0 I$$ $$ B = \frac{\mu_0 I}{2\pi(R+y)}$$

So now, for a semi-infinite wire, I take away half the wire and hence half the vector field. But, before I just say that the new field is half of the original one, I need to establish that the B-fields from each "half" of the infinite wire are in fact in the same direction so that they add in parallel fashion. The Biot-Savart law tells us that each wire element produces a B-field that is perpendicular to the current and perpendicular to a displacement joining the wire element and the point at which I wish to know the field. So, the B-field is always in a direction azimuthal to the wire, whichever piece(s) of wire we consider. This means that the new B-field for the semi-infinite wire is in the same direction as for the full infinite wire but has half the magnitude.

Answer 2

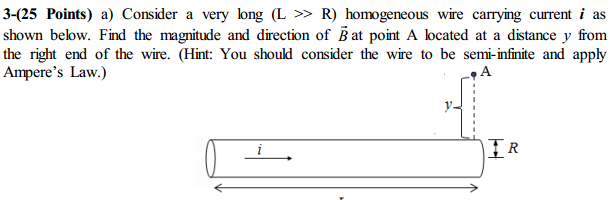
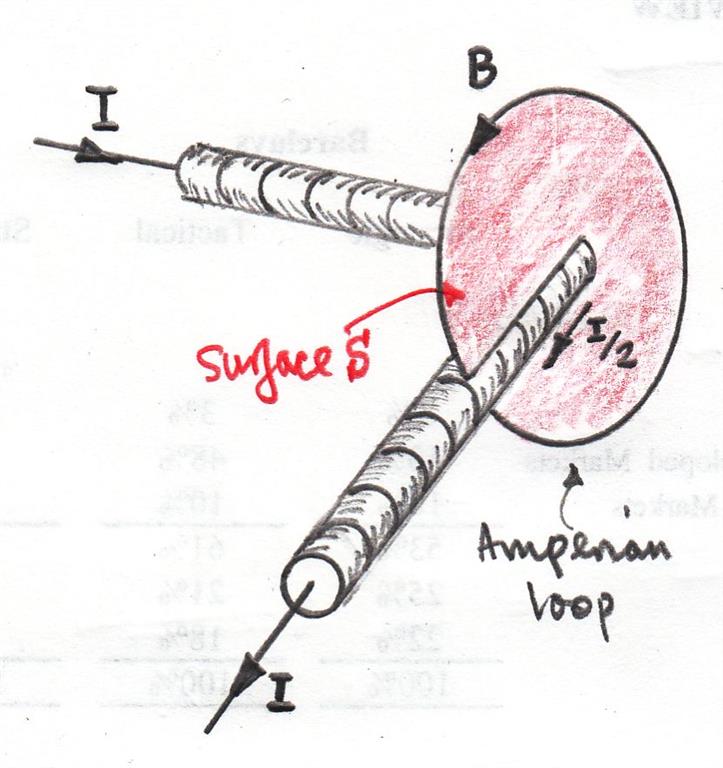
To be clear, you can use Ampere's Law: $$\oint \vec B\cdot \mathrm{d}\vec\ell=\mu_0I_\text{enc}.$$ Specifically, it is the form without the displacement current, and it works because you are in magnetostatics. And Rob Jeffries' answer is totally satisfactory. But to specifically address your concern with the charge build-up lets look at an example of a proper time dependent generalization of Biot-Savart.

An example of a solution to Maxwell can be provided if both the electric and magnetic field are computed as the electric and magnetic parts of the electromagnetic field given by Jefimenko's equations:

$$\vec E(\vec r,t)=\frac{1}{4\pi\epsilon_0}\int\left[\frac{\rho(\vec r',t_r)}{|\vec r -\vec r'|}+\frac{\partial \rho(\vec r',t_r)}{c\partial t}\right]\frac{\vec r -\vec r'}{|\vec r -\vec r'|^2}\; \mathrm{d}^3\vec{r}' -\frac{1}{4\pi\epsilon_0c^2}\int\frac{1}{|\vec r-\vec r'|}\frac{\partial \vec J(\vec r',t_r)}{\partial t}\mathbb{d}^3\vec r'$$ and $$\vec B(\vec r,t)=\frac{\mu_0}{4\pi}\int\left[\frac{\vec J(\vec r',t_r)}{|\vec r -\vec r'|^3}+\frac{1}{|\vec r -\vec r'|^2}\frac{\partial \vec J(\vec r',t_r)}{c\partial t}\right]\times(\vec r -\vec r')\mathbb{d}^3\vec r'$$ where $t_r$ is actually a function of $\vec r'$, specifically $t_r=t-\frac{|\vec r-\vec r'|}{c}.$

These reduce to Coulomb and Biot-Savart only when those time derivatives are exactly zero, which is statics. So Jefimenko is an example of proper time dependent laws for the electromagnetic field. Note that both the electric and the magnetic part of the electromagnetic field have parts that depend on the time variation of current.

When the time variation of the current is zero, the magnetic field is solely determined by the current. Full stop. Do not worry about displacement current. Instead you have to worry that for a point $\vec r$ at a time $t$ you have to consider those places $\vec r'$ that could have current and look at what the current was back at time $t_r=t-\frac{|\vec r-\vec r'|}{c}.$ And if that current was changing back then, you need to look at its time derivative back then too. But for magnetic fields that is it.

For electric fields you will care about the charge, and the rate charge is piling up, and the time rate of change of the current. If you want to use Ampere in a situation where the current never changes, then you can.

If the current changes then this will produce an additional magnetic field, and it will also produce an additional electric field. And you can compute the additional magnetic field by looking at the rate of change of the displacement current.

But Jefimenko's equations make a casual version obvious. The current and its change, both in the past, cause the magnetic field here in the present. And the fields here in the present bear some relationships to each other because of their common cause.

So if you use these equations, the change in current directly causes both electric and magnetic fields. But when the current changes at place-time $(\vec r_1,t_1)$, there is an electric and a magnetic field. But the field exists only at place-times $(\vec r_2,t_2)$ where $t_2=t_1+\frac{|\vec r_2-\vec r_1|}{c}$.

Answer 3

As you can see from an example of electrodynamics book by Griffiths:

For ANY wire,equation (5.35) still holds.And you can see that for an infinite wire,θ1=π/2 and θ2=-π/2.So,in your situation you can use only θ1 οr θ2 by changing the other angle to zero.It does not matter which angle you keep,mathematically,they will both give you a B of the same magnitude and direction.

And a neat trick,because for an infinite wire you use θ1=π/2 and θ2=-π/2,for sinθ the two angles give you the same answer.So,for half-infinite wire,you can just use the B for an infinite wire divided by 2!Its only half of μοI/2π(r+y)!

Answer 4

I think that you can use the Biot Savart law in the electro quasistatic approximation even when displacement current are present. For example you can compute the magnetic field generated by a semi infinite segment with a intensity $I$ using the Biot Savart law.

You have to put a charge $Q(t)$ at the end of the segment with $I=\frac{dQ}{dt}$. But if you use the Biot Savart law, this charge plays no role.

An other method woud be to use the Maxwell Ampere equation in integral form $\oint_{\Gamma }{\overrightarrow{B}\cdot \overrightarrow{\text{d}l}=\iint_{\Sigma }{{{\varepsilon }_{0}}{{\mu }_{0}}\frac{\partial \overrightarrow{E}}{\partial t}\cdot \overrightarrow{\text{d}S}}}$ with the field $\overrightarrow{E}$ created by $Q(t)$ computed by Coulomb law $\overrightarrow{E}(M,t)=\frac{Q(t)}{4\pi {{\varepsilon }_{0}}{{r}^{2}}}\overrightarrow{{{e}_{r}}}$.

You can check that we find the same result.

Answer 5

This example can be done using the simple form of Ampere's law.
Rather than get an accumulation of charge at the end extend the conductor out at right angles and off to infinity. You then have an infinite L-shaped conductor.