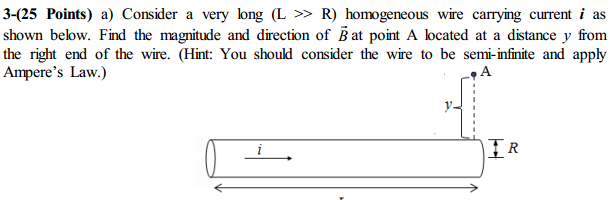
Suppose that there is a semi-infinite wire which extends to infinity only in one direction. There are no other circuit elements at the other end(finite end) of the wire and the current does not loop. The magnetic field obviously has cylindrical symmetry when the Amperian contour is taken as a circle with its center on the wire.
However, due to charge accumulation there is a time-dependent electric field; hence a displacement current. How can I formulate the Ampere's law and show that the magnetic field is the half of that the infinite wire at the finite end of the wire? Do you think treating charge accumulation as a point charge with changing amount of charge right at the finite end of the wire will suffice?
The original question is: