7
$\begingroup$

Kerr Black Holes have usually (excluding extrema $a=0$, $a=1$) due to their spinning activity an ellipsoidal ergosphere.

So why does the photon-sphere does not have an ellipsoidal form?

On the possibility of observation of the future for movement in the field of black holes of different types. Yu.V. Pavlov. Gen. Relativ. Gravit. 45, 17 (2013), arXiv:1203.4000.

Improve this question
$\endgroup$
1
  • $\begingroup$ Please read our comprehensive paper on photon spheres published in Journal of Mathematical Physics entitled The Geometry of Photon Surfaces, which is available on the Arxiv. For related areas you might like to look at this publication list. $\endgroup$
    – Kumar
    Commented May 6, 2015 at 13:10

3 Answers 3

Reset to default
1
$\begingroup$

So why does the photon-sphere does not have an ellipsoidal form?

It does have an an ellipsoidal form, or, more exactly, that of an oblated sheroid; in Boyer Lindquist coordinates where

$${x} = \sqrt {r^2 + a^2} \sin\theta\cos\phi \ , \ \ {y} = \sqrt {r^2 + a^2} \sin\theta\sin\phi \ , \ \ {z} = r \cos\theta$$

the r-coordinate of all the possible photon orbits is constant, but if you transform the pseudospherical coordinate system into the cartesian background space the constant r does indeed transform into an ellipsoid.

For comparison see the ergospheres and horizons of a rotating black hole in pseudospherical $r, \theta, \phi$ (left, for comparison see Fig. 3 in Nigel Sharp's paper "On embeddings of the Kerr geometry") and $x,y,z$ (right, comparison at Fig. 3 in Matt Visser's paper "The Kerr spacetime: A brief introduction" ) coordinates:

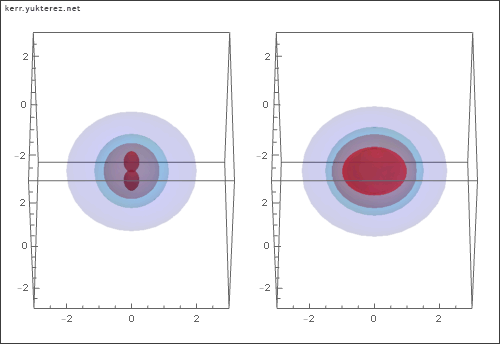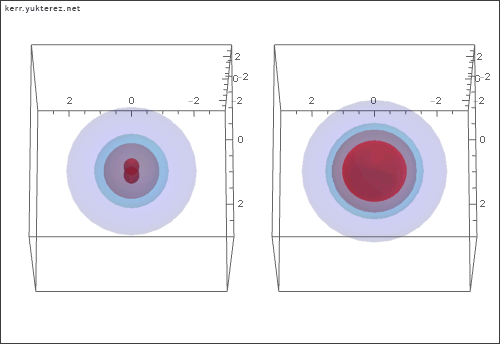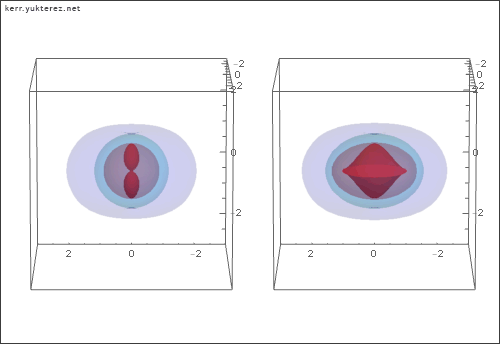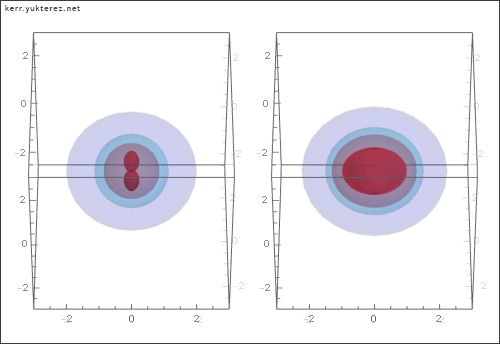
r,θ,φ and x,y,z coordinates

While the horizons have a constant $r$ in Boyer Lindquist coordinates, they don't have a constant $R$ in cartesian coordinates. That of course also goes for photon orbits:

ellipsoid photon orbit

As you can see, the shape of the photon trajectory in cartesian background space is not sperical, but an oblated spheroid. In Fig. 4 of Edward Teo's paper "Spherical photon orbits around a Kerr black hole" (pdf), you can find the same orbit, but in pseudospherical Boyer Lindquist coordinates, where the orbit looks spherical again.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Please fix the broken links in this post by providing stable (DOI) references. $\endgroup$
    – Emilio Pisanty
    Commented Mar 11, 2019 at 15:36
  • 1
    $\begingroup$ We do not judge people who use it, but linking to Sci-Hub is inappropriate here. Similarly, linking to non-stable links is just shooting your answer in the foot, basically ensuring that in one to five years your links will have rotted away. Further, you should not link directly to pdfs without explicitly marking them as such, and only when a landing page isn't available. $\endgroup$
    – Emilio Pisanty
    Commented Mar 11, 2019 at 17:54
  • $\begingroup$ I didn't know that, I was linking to the specific pages where the figures I was talking about can be found so the reader would not have to search the whole books $\endgroup$
    – Yukterez
    Commented Mar 11, 2019 at 19:19
  • 1
    $\begingroup$ Then specify the page in the answer text. Linking to pdfs is rather aggressive to users on mobile or lower-speed connections - link to the landing page and they can then decide if they want to download it. $\endgroup$
    – Emilio Pisanty
    Commented Mar 11, 2019 at 20:11
0
$\begingroup$

I dont' see much discussion on the linked article about the photon sphere being a true sphere -- they talk about the photon orbit at $r=3M$, but this is only valid for an equatorial orbit (and I'd expect that it would depend on the value of $a$ and you'd have a different radius for corotation and anti-rotation), and when you go away from the equatorial plane, you'll have different values for the orbit.

I'll admit that I"m talking from intuition here, I haven't done the calculation for closed null geodesics in the kerr spacetime.

Short, non-rambly answer: I don't know if the kerr hole even has a photon-sphere in the Schwarzschild case, and if it did, my intuition screams that it will not be a sphere. (also note, $r=const, t=const$ does not define a sphere in the Kerr spacetime)

Improve this answer
$\endgroup$
-3
$\begingroup$

Photon "spheres" of Kerr metric are not yet found. That needs a lot of work. Two closed photon circles are known.

Improve this answer
$\endgroup$
1

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.