I'm reading the Britannica guide to Electricity and Magnetism, and I came across the following quote:
A fundamental property of a magnetic field is that its flux through any closed surface vanishes.
Can someone explain this in simpler terms?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ This isn't going to mean much right now, but it will later: the fact that magnetic flux through a closed surface vanishes is actually a convention. It is equivalent to assuming that there are no "magnetic charges". In fact,the slightly more generally true statement would be that, as far as we know from experimental data, any theory we cook up to describe it must have the ratio between electric and magnetic charge fixed at all points in space. This is explained in Jackson's EM book. $\endgroup$– DanielSankCommented Apr 11, 2015 at 23:09
-
$\begingroup$ @DanielSank : product, my friend, not ratio. $\endgroup$– KphysicsCommented Oct 9, 2019 at 11:06
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
4
Can someone explain this in simpler terms?
Typically, the closed surface is a mathematical surface (Gaussian surface) which simply defines an 'inside' and 'outside'.
Since, as far as we know, there are no magnetic charges from which magnetic field lines start or end, any magnetic field line entering must exit through the surface; any magnetic field exiting must enter through the surface.
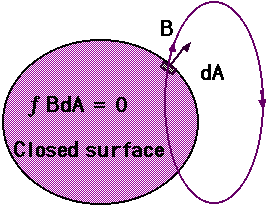
Thus, the number of field lines entering equals the number of field lines exiting and the flux of the magnetic field through the surface is zero.
answered Apr 11, 2015 at 22:38
-
$\begingroup$ This has not helped me understand it. I already know that flux is the same as magnetic field lines. If you could illustrate this with a picture or just explain it more simply, I would be very grateful. Particularly, I don't understand what the volume and surface are. $\endgroup$ Commented Apr 11, 2015 at 22:40
-
$\begingroup$ @user1917407, magnetic flux isn't the same as magnetic field lines. The magnetic flux through the closed surface is proportional to the number of field lines leaving the volume enclosed minus the number of field lines entering. If no field lines start or stop within the volume enclosed, the number of lines entering equals the number of lines leaving and, thus, the flux is zero. $\endgroup$ Commented Apr 11, 2015 at 22:44
-
$\begingroup$ Is the closed surface the magnet? That is what I am trying to understand. Also, does magnetic flux only apply to the interior of the magnet, while field lines apply to the outside of the magnet? Also, shouldn't the field lines leaving and entering always be equal, and flux would always be 0? $\endgroup$ Commented Apr 11, 2015 at 22:50
-
$\begingroup$ @user1917407, I've added additional details to address some of your comments. $\endgroup$ Commented Apr 11, 2015 at 23:09
$\begingroup$
$\endgroup$
2
If the flux in and out of a surface cancles, there is no need for magnetic charge in which field lines can end or start (e.g. like the electric charge). One expresses this like
$$ \nabla \cdot \vec{B} = 0 $$
wich means $$ 0 = \int_V \nabla \cdot \vec{B} ~ dV = \int_S \vec{B} \cdot d\vec{S} $$ where $S$ is the surface of the volume $V$.
-
$\begingroup$ Thank you for your response, but I don't know calculus. I'm really just looking for a barebones explanation of what the surface represents and how the flux relates to that. $\endgroup$ Commented Apr 11, 2015 at 22:50
-
$\begingroup$ @user1917407: Just take the first sentence of my annswer then: It means that there is no excess charge within the surface. Since this holds for all surfaces, there is no magnetic charge. $\endgroup$– image357Commented Apr 11, 2015 at 22:53
$\begingroup$
$\endgroup$
2
There are no magnetic monopoles. i.e. Unlike electric field flux, there are no sources or sinks of magnetic flux.
Therefore the amount of flux entering any closed volume must equal the amount exiting.
-
$\begingroup$ - That's more of a flat statement than any sort of explanation. I'm happy to accept that oodles of experimental evidence supports it, but why can't a monopole exist? I suspect the short answer is conservation of energy, but that leaves me wondering, given the requirement for mass/energy conservation, how the universe around me can exist. Zero sum game? $\endgroup$ Commented Apr 12, 2015 at 8:46
-
$\begingroup$ @PeterWone It is the physical reason for the statement in the question. Magnetic monopoles could exist, but then the statement in the question would be false. If you look around on Physics SE you will see there are formulations of Maxwell's eqns that do admit monopoles, but then the RHS of the solenoidal law is not necessarily zero. $\endgroup$– ProfRobCommented Apr 12, 2015 at 12:22