Do photons lose energy due to gravitational redshift? If so, where does the lost energy go? [duplicate]

Question

In the gravitational redshift, the frequency of photons radiated from some source is reduced. As the energy of a photon is given by $\hbar\omega$, if the frequency is reduced where is the lost energy?

Answer 1

Update:

According to this paper, "On the Interpretation of the Redshift in a Static Gravitational Field", the answer I give below is a common but misleading interpretation.

The classical phenomenon of the redshift of light in a static gravitational potential, usually called the gravitational redshift, is described in the literature essentially in two ways: on the one hand the phenomenon is explained through the behaviour of clocks which run the faster the higher they are located in the potential, whereas the energy and frequency of the propagating photon do not change with height. The light thus appears to be redshifted relative to the frequency of the clock. On the other hand the phenomenon is alternatively discussed (even in some authoritative texts) in terms of an energy loss of a photon as it overcomes the gravitational attraction of the massive body. This second approach operates with notions such as the “gravitational mass” or the “potential energy” of a photon and we assert that it is misleading.

---

Do photons lose energy due to gravitational redshift?

More precisely, the redshift is how the loss of energy is manifest.

For a massive particle moving radially away from a (Newtonian) gravitational source, kinetic energy is 'traded' for gravitational potential energy. Since the KE is proportional to the speed squared, the loss of KE is manifest as reduced speed.

Since the speed of a photon is always $c$, it might seem that photons would not lose energy propagating away from a gravitational source. However, as Einstein demonstrated with a simple thought experiment, if photons did not lose energy, we could in principle build a perpetual motion machine. From page 119 of "A first course in general relativity":

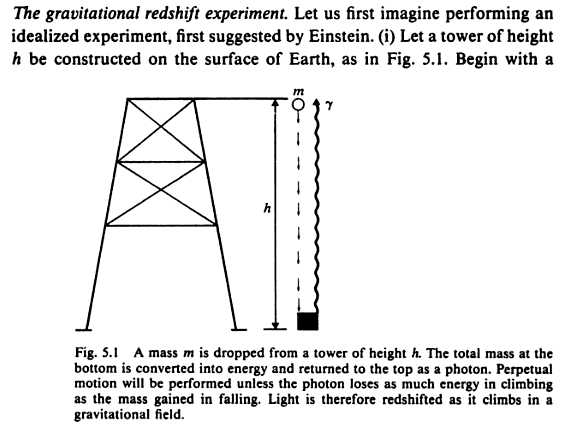
Thus, photons must lose energy. And, since the photon's energy is proportional to the frequency, it follows that this loss of energy will be manifest as reduced frequency.

---

if the frequency is reduced where is the lost energy?

In the outlined experiment, before the mass $m$ is dropped, there is energy stored in the system since, at some point, work was done to raise the mass to height $h$.

During the fall, conversion of mass to photon, climb of photon, and conversion of photon to mass, the energy of the system is unchanged though energy is converted from one form to another.

---

As has been suggested in the comments, the issue of energy conservation in general relativity is subtle when the spacetime is dynamic. However, that is not the context of this idealized thought experiment.

Answer 2

First possible point of view: In the Pound–Rebka experiment the redshift / blueshift of photons is measured in small distances. This experiment one explain by the influence of gravitational field on the photon: "When the photon travels through a gravitational field, its frequency and therefore its energy will change due to the gravitational redshift."(https://en.wikipedia.org/wiki/Pound-Rebka_experiment)

Second point of view: The frequency of photons do not changes during its life. Light "... consists of a finite number of energy quanta which are localized at points in space, which move without dividing, and which can only be produced and absorbed as complete units."A. Einstein Concerning an Heuristic Point of View Toward the Emission and Transformation of Light

In accordance with the second point of view the Pound-Rebka experiment has to be interpreted by an other way. The source and the receiver are located in points with different gravitational potential and that is the reason they capable to emit and receive photons at different frequencies. The statement in the first point of view is wrong.

Answer 3

Some redshifts are an example of the Doppler effect, familiar in the change in the apparent pitches of sirens and frequency of the sound waves emitted by speeding vehicles. A redshift occurs whenever a light source moves away from an observer.

The energy balance is with the source of the photons. If the source is moving away the photons have less energy than the photons of a source that moves towards the detector.

Another kind of redshift is cosmological redshift, which is due to the expansion of the universe, and sufficiently distant light sources (generally more than a few million light years away) show redshift corresponding to the rate of increase in their distance from Earth.

Finally, gravitational redshift is a relativistic effect observed in electromagnetic radiation moving out of gravitational fields.

The energy is balanced by the system "gravitational field/photon"

Reminder: redshifts and blue shifts are detected by the changes in the spectrum of specific atoms

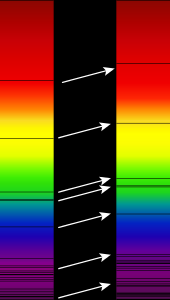
Absorption lines in the optical spectrum of a supercluster of distant galaxies (right), as compared to absorption lines in the optical spectrum of the Sun (left). Arrows indicate redshift. Wavelength increases up towards the red and beyond (frequency decreases).

To clarify about energy conservation and General Relativity, there is no problem in this case:

Very massive objects emitting light

Light from the Sun appears redshifted to an Earth bound astronomer. In quasi-newtonian terms, we might say that light loses kinetic energy as it climbs out of the gravitational well of the Sun, but gains potential energy. General relativity looks at it differently. In GR, gravity is described not by a "potential" but by the "metric" of spacetime. But "no problem", as the saying goes. The Schwarzschild metric describes spacetime around a massive object, if the object is spherically symmetrical, uncharged, and "alone in the universe". The Schwarzschild metric is both static and asymptotically flat, and energy conservation holds without major pitfalls.