Puzzling : Relative motion of two points on a rotating disc

Question

Consider two points on a radial line for a rotating disc. One point, $A$, is at the circumference and the other, $B$, is at distance $R/2$ from disc's centre. Relative velocity of $B$ w.r.t. $A$ should be ($\omega R/2$) by usual subtraction of individual velocities. However as seen by $A$, $B$ remains at fixed distance and also doesn't rotate (relative angular velocity is zero). Then how can $B$ have a relative velocity w.r.t. $A$?

Answer 1

If I understand your question correctly you are saying that:

$$ v = r\omega $$

and therefore:

$$\begin{align} v_A &= r\omega \\ v_B &= \tfrac{1}{2}r\omega \\ v_A &= 2v_B \end{align} $$

but how can $A$ and $B$ have different velocities when they are both attached to the disk so the separation between is fixed?

The answer is that $A$ and $B$ have different accelerations, because the acceleration is given by:

$$ a = r\omega^2 $$

$A$ and $B$ do indeed have different velocities, but $A$ accelerates twice as fast as $B$ does and this keeps the magnitude (not the direction) of the separation constant.

Answer 2

Yes, $B$ does rotate when seen from a static frame of coordinates outside the disk: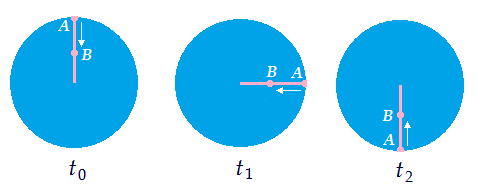

As to velocities and accelerations, see the article in Wikipedia. It says,

$$\vec {v_s} = \vec {v_r} + \vec {\Omega} \times \vec r,$$

where $v_s$ is the velocity in the static frame and $v_r$ in the rotating. If you apply this formula for both points $A$ and $B$, their velocities in the static frame are zero, s.t. they are at rest with respect to one another. But if you subtract the formula for $A$ from the formula for $B$, you find that in the static frame they have relative velocity due to the term with $\vec {\Omega}$.

Answer 3

A rotating reference frame is an accelerated reference frame so $A$ and $B$ are at rest in an accelerated reference frame.

Assume an inertial reference frame $S_0$ and another reference frame $S$, with a common origin and rotating with respect to $S_0$. Let the (constant) angular velocity vector of $S$ be $\mathbf \Omega$.

Then, the time rate of change of a vector $\mathbf Q$ in the inertial frame is given by

$$\left(\frac{d\mathbf Q}{dt} \right)_{S_0} = \left(\frac{d\mathbf Q}{dt} \right)_S + \mathbf \Omega \times \mathbf Q$$

For your problem, assume the disk is in the $xy$ plane and $\mathbf \Omega$ is along the $z$ axis

$$\mathbf \Omega = \omega \hat{\mathbf z}$$

Let $\mathbf r_{BA}$ be the separation vector between $B$ and $A$. Since $\mathbf r_{BA}$ is in the $xy$ plane and radially directed, it follows that

$$\mathbf \Omega \times \mathbf r_{BA} = \omega\, r_{BA}\; \hat{\boldsymbol \phi}$$

In the inertial reference frame, the separation vector is constant in magnitude and radially directed thus

$$\left(\frac{d\mathbf r_{BA}}{dt} \right)_{S_0} = \dot \phi\, r_{BA}\; \hat{\boldsymbol \phi} = \omega\, r_{BA}\; \hat{\boldsymbol \phi}$$

So, in the rotating frame of reference

$$\left(\frac{d\mathbf r_{BA}}{dt} \right)_S = \left(\frac{d\mathbf r_{BA}}{dt} \right)_{S_0} - \mathbf \Omega \times \mathbf r_{BA} = \omega\, r_{BA}\; \hat{\boldsymbol \phi} - \omega\, r_{BA}\; \hat{\boldsymbol \phi} = 0$$

In the inertial frame of reference, the separation vector changes with time, i.e., $B$ and $A$ have a relative velocity, but, in the accelerated frame of reference, their separation vector is constant.

Answer 4

However as seen by A , B remains at fixed distance and also doesn't rotate (relative angular velocity is zero).

But it does rotate, if your reference frame does not rotate, but just gets centered on your point of interest. If you want to consider a rotating reference frame, then all points (that are fixed to the disc, or to the frame) are obviously and by definition stationary inside it, and there is no "relative motion" between them in such a reference frame.