Yes, $B$ does rotate when seen from a static frame of coordinates outside the disk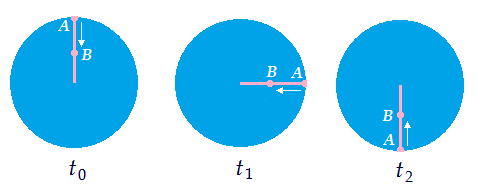 :
:
As to velocities and accelerations, see the article in Wikipedia. It says,
$$\vec {v_s} = \vec {v_r} + \vec {\Omega} \times \vec r,$$
where $v_s$ is the velocity in the static frame and $v_r$ in the rotating. If you apply this formula for both points $A$ and $B$, their velocities in the static frame are zero, s.t. they are at rest with respect to one another. But if you subtract the formula for $A$ from the formula for $B$, you find that in the static frame they have relative velocity due to the term with $\vec {\Omega}$.
