I have previously heard that some scientists are interested in trying to reformulate statistical mechanics in different ways to try and create new ways to solve novel problems.
This got me wondering, what are the fundamental "components" that you would need to re-derive to have a fully (or mostly) equivalent formulation of statistical mechanics? In essence, if you were to create a list of concepts, like the ergodic principle, partition function, quantum distributions, law of entropy, etc., what fundamental tools would you need to have in order to be able to derive many of the useful results of statistical mechanics, (for example the equipartition of energy, fermi/bose energies, the classical limit for gases)?
For example, we need the idea of discrete states, self-interference, the pauli exclusion principle, and some kind of energy relationship (like the hamiltonian) before we would be able to make ladder operators for the QM SHO, or derive it's energy levels.
To clarify, let's just say we were interested in constructing a tree graph of statistical mechanics in a way that was analogous to this outline of thermodynamics
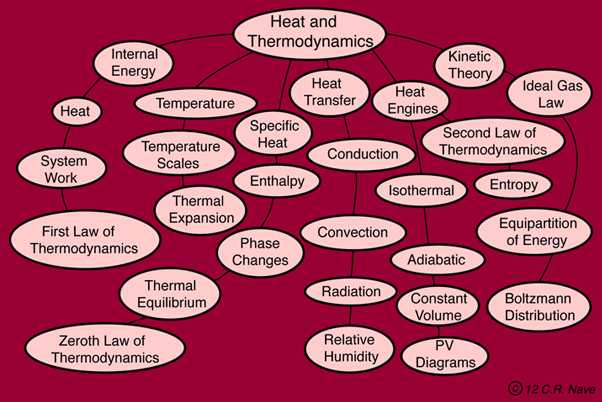
What would be the concepts the first "step" down on the tree for Statistical Mechanics?
