How does the Moon cause the tides?
By gravitation.
I'll start with an overly simplistic view of the tides. Suppose that the Moon was in a geosynchronous orbit and that the Earth was completely covered by water. In that case, the Earth's oceans would adjust themselves to form an equilibrium tide, first proposed by Newton, in which the surface of the oceans form an equipotential surface. The equilibrium tide is depicted below.
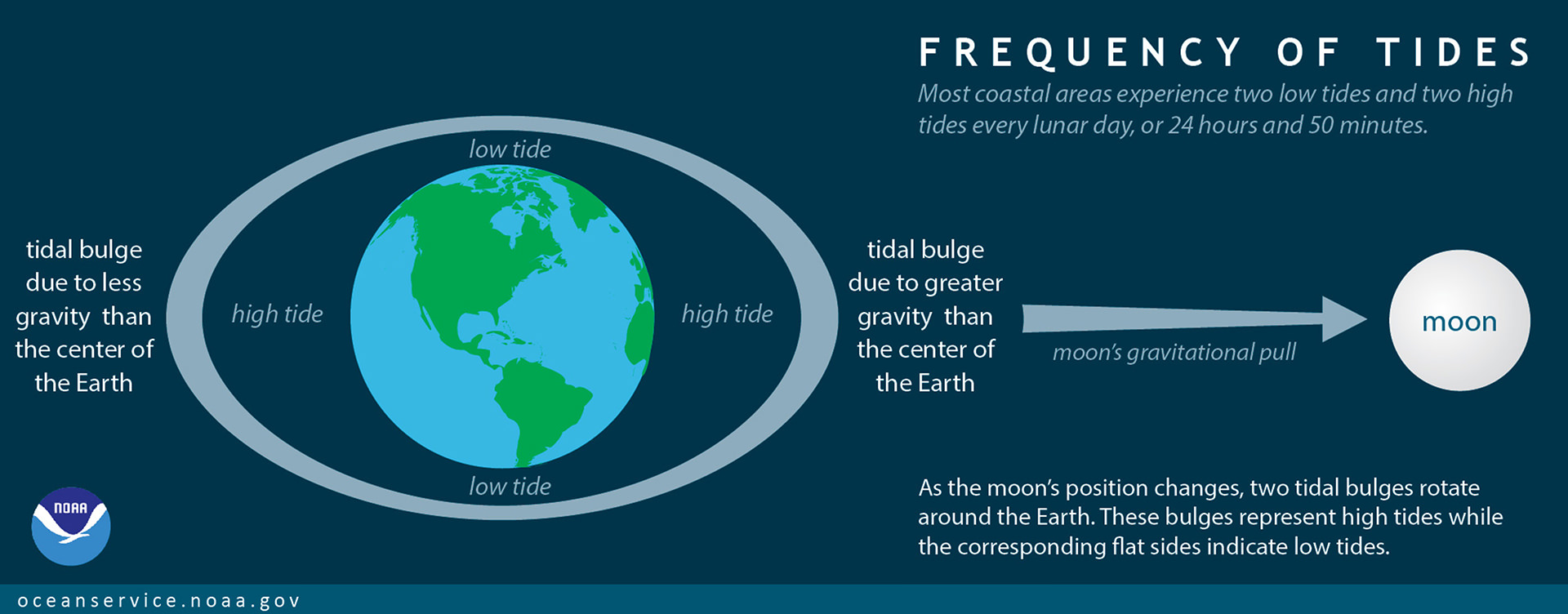
Image source: http://oceanservice.noaa.gov/facts/tidefrequency.html
I chose this image because unlike most, it does not invoke centrifugal force to explain the bulge opposite the Moon. The near side bulge, if it existed, results from the gravitational acceleration toward the Moon on the point on the Earth closest to the Moon being slightly stronger than the action on the Earth as a whole, while the far side bulge results from the gravitational acceleration there being slightly less than the action on the Earth as a whole.
A number of things prevent that equilibrium tide from existing: The Moon is not in a geosynchronous orbit, the Earth is not completely covered by water, a month is much longer than is a day, and others. The equilibrium tide, aka the tidal bulges, does not exist. This means the Earth's oceans are perpetually in a state of disequilibrium with respect to the forces that generate the tides.
To arrive a better view of those forces, I'll look at things from the perspective of a reference frame with its origin at the center of the Earth. From the perspective of this frame, the gravitational acceleration at a point on the Earth's surface toward the Moon is the vector difference between the gravitational acceleration at that point toward the Moon and the gravitational acceleration of the Earth as a whole toward the Moon. This is depicted in the left half of the below image.
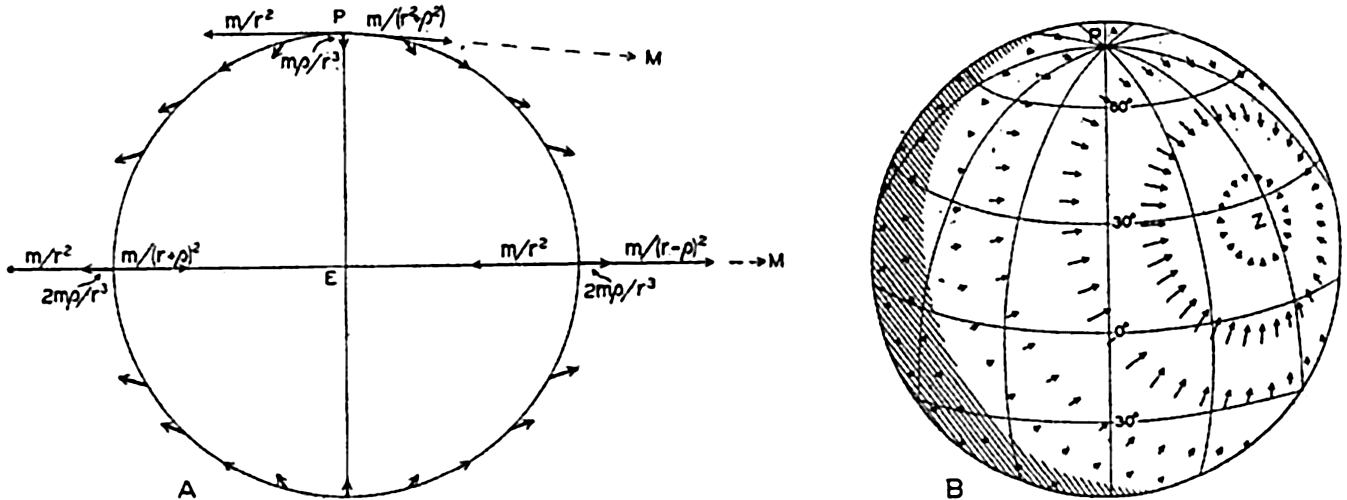
Image source: GH Darwin, "The tides and kindred phenomena in the solar system, 3rd ed.", Houghton, Mifflin (1911), via HU Sverdrup, MW Johnson, and RH Fleming, "The Oceans: Their Physics, Chemistry, and General Biology, Vol. 7." Prentice-Hall (1942) at http://publishing.cdlib.org/ucpressebooks/view?docId=kt167nb66r;brand=eschol.
Note that this force has both vertical and horizontal components. The vertical component of this force has a negligible effect on the Earth's oceans. The horizontal component however has a significant effect. This is what drives the tides in the oceans. The horizontal component this force, the tide-generating force, is depicted in the right half of the above image. (Note that a similar effect arises from the Sun, which also raises tides, but to a lesser extent. The tides raised by the Sun are a bit less than half those raised by the Moon.)
While this tide-generating force is very small, less than 10-6 newtons per kilogram of water, it is ever-present and acts in a coordinated manner over half of the globe, and in an opposite coordinated way over the other half of the globe. Tsunamis (sometimes erroneously called tidal waves) form an extreme example of an impulse response. Because the tide-generating force is ever-present, the tides are a forced response rather than an impulse response.
The tide-generating force at some point on the surface of the Earth is oscillatory over time, with multiple frequency components. The response of a resonant system to an oscillatory forcing function is to oscillate ate the frequency of that forcing function, but modified by the natural frequency of the resonant system. This results in a dynamic (as opposed to equilibrium) theory of the tides.
Laplace first proposed his dynamical theory of the tides 240 years ago. Variations in the tidal forcing, coupled with bathymetry and the Coriolis effect, results in a number of tidal resonance systems. Improvements to Laplace's dynamical theory of the tides in the latter part of the 19th century resulted in a very successful predictive of the tides. The tides can be predicted locally with remarkable success thanks to the late 19th / early 20th century works of George Darwin (Charles Darwin's son), Ernest William Brown, Arthur Thomas Doodson, A.E.H Love, and others.
That 100 year old theory is capable of predicting tides locally by observing the tides at that point over a span of time and performing harmonic analyses. A global point of view was out of reach to those late 19th / early 20th century scientists. Thanks to global observations and to modern computing, a global point of view is now becoming within reach.