Coordinates for FLRW metric

Question

In GR, coordinate are just a tool for us to describe the physics, they should be equivalent. However, in standard form of FLRW metric, it can be inferred that the universe is expanding, but we can do a coordinate transformation to make the spatial part static or changing in a different way with respect to time. Is there a notion of expanding universe which does not depend on coordinates?

Answer 1

The standard coordinate system is the mathematically simplest, but I don't think it's actually the most physically intuitive. This is because we live on objects that are gravitationally bound, and admist objects that are electromagnetically defined. This means that our local length scales are not affected by cosmological expansion. But, if you look at the FRLW metric, in its standard form (I choose the flat cosmology for simplicity):

$$ds^{2} = - dt^{2} + a(t)^{2}\left(dr^{2} + r^{2}d\theta^{2} + r^{2}\sin^{2}\theta d\phi^{2}\right)$$

you can tell that, for some constant-t observer, the ruler actually expands with time by a factor $a$. For this reason, when describing cosmological observations, I actually like to use a different coordinate system, where you replace $r$ with $R = a(t)r$. Then, you have $dR = {\dot a}r\,dt + a\,dr \rightarrow dr =dR- H (R/a)\,dt$, and the metric becomes (note that I used the relation $H = \frac{\dot a}{a}$, to replace $a$ with Hubble's "constant"):

$$ds^{2} = -\left(1-H^{2}R^{2}\right)dt^{2} + 2dR\,dt\left(-HR\right) + dR^{2} + R^{2}d\theta^{2} + R^{2}\sin^{2}\theta d\phi^{2}$$

In terms of direct physics, this coordinate system is a lot clearer. You see that, for a constant-t observer, there is a coordinate singularity at $R = \frac{1}{H}$, corresponding to the cosmological horizon. Furthermore, this coordinate system has a $g_{tr}$ coordinate, which, it can be shown, corresponds to the frame dragging of the system -- so space naturally expands away at a velocity proportional to $HR$, which gives you Hubble's law.

Answer 2

Comments to the question (v3):

1. It is true that there exists a huge freedom to choose local coordinates in GR, but it is not possible to alter the metric tensor $g_{\mu\nu} dx^{\mu}dx^{\nu}$ (when we include the basis elements $dx^{\mu}$ and $dx^{\nu}$).

2. Given an arbitrary but single fixed spacetime point $p$, there exist Riemann normal coordinates.

3. We cannot get the metric components $g_{\mu\nu}$ on an arbitrary prescribed symmetric form (with Minkowski signature) in an open neighborhood, no matter how small. It is not a free lunch!

Answer 3

All coordinate systems are equal, but some systems are more equal than others ;)

In case of Friedmann universes, there's a distinguished set of coordinates that corresponds to a family of freely-falling observers which see the universe as isotropic and chosen so that matter is distributed homogeneously within a spatial slice at constant time.

Furthermore, we could choose our coordinates so that the time-like coordinate agrees with proper time of our observers and the space-like coordinates agree with proper distance within a spatial slice.

This is just one possible choice among many: For example, observers in relative motion would not see the universe as isotropic, and their description of the matter distribution would be as valid as the one we chose - just less convenient.

Even if we keep our set of observers, we're free to scale our coordinates as we see fit. For example, using conformal time and comoving coordinates makes FLRW spacetime look deceptively like Minkoswki space with a static matter distribution:

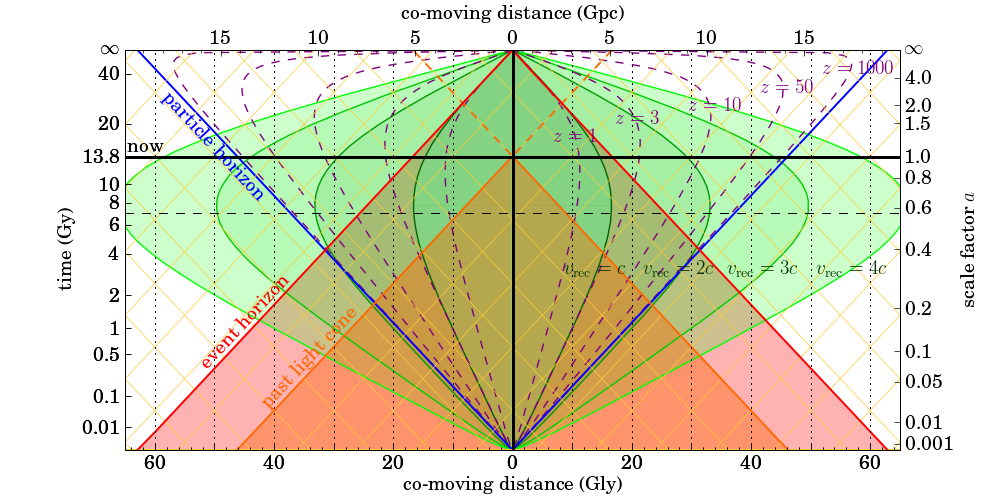
(source)

Note how the yellow light rays are given by straight lines and that galaxies would sit at a fixed comoving distance.

This, however, is misleading, the same way that doing a logarithmic plot does not change the underlying function. Note that whatever the coordinates, the math will of course still work out thanks to the magic of tensor calculus: spacetime will remain curved, proper distance within spatial slices will increase and light will experience redshift.