Prevent a box from tipping over: height of CG?

Question

I'm on a problem that can be simplified in the following way:

A box is placed on a horizontal plane, and is pinned to the ground on one side only such that it can tip over that side. Given a disturbance force normal to one of its side faces, how high does it need to be to make the box tip over?

Diagram:

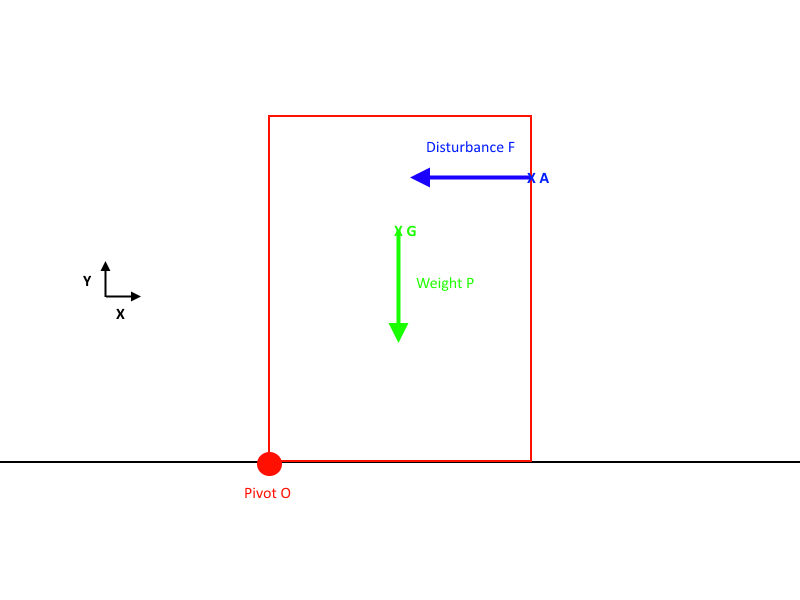
I think it is a very simple problem, and the way I see it the answer should be: $$M(O) = y_F F-x_P P \Rightarrow F_{tipover}=\frac{x_P P}{y_F}$$

Which means that force does not depend on the height of the center of gravity. Someone insists the lower the CoG, the higher this force (the more stable the box). Intuitively it seems to make sense especially on an inclined plane, but the maths are against it at least on a horizontal plane.

What do you think?

Answer 1

In a sense, you're both right. You're correct in that the force required is independent of the height of the centre of gravity, while your friend is correct that a lower CoG makes the box more stable. This may seem contradictory, but it's not, and here's why: the force required to move the box is independent of the height of the centre of gravity, but the work done to the box is not. The stability of the box is essentially defined as how far do you need to top it for it to topple over. This winds up being how far do you need to tip it for the CoG to pass over the pivot point so that it starts pulling the box down onto its side. If the CoG is very high, then it doesn't need to be tipped as far as if it's very low. And how far you tip the box is measured by the work done to it, not just the force that's applied. So while the magnitude of force needed to move the box is independent of the height, how long that force needs to be applied to get the box to tip over is not.

Answer 2

Your are correct, it only depends on the distance x, same with the applied force, it doesnt matter if you apply it at the back opr at the front as far it is at the same height.