0
$\begingroup$

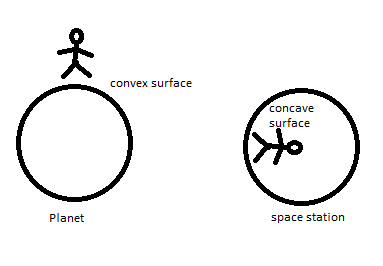
I read this in a comment to an answer in physics.stackexchange.com. The comment was

An easier method might be to just place a straight, rigid beam on the floor. If you find the floor is concave, you're probably on a space station. The technique you describe would only work on a relatively small station (which would therefore require a rather large angular velocity to achieve 9.8m/s centrifugal acceleration) where you would probably notice the concavity of the floor just by looking at it. This phenomenon would also be observed on Earth, but the angular velocity of the planet is sufficiently small that atmospheric drag tends to overshadow its affect on ballistic objects

The discussion was about distinguishing between real gravity and rotating space station. My question is what does the commentator mean by concavity of the floor?

Note: Since I have only a few reputation, I cannot comment on that answer to ask the commenter to explain it.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

That the floor is not flat but circular, an the centrifugal force acts radially, and is the same at the same radius. If you cover the concavity of the floor with a planar surface or either make the stations of planar segments (a polygon instead of a circle), then you will measure different accelerations at different points of the floor, because they are not equidistant to the center of rotation.

Improve this answer
$\endgroup$
6
  • $\begingroup$ @Wolfram: What do you mean by "That the floor is not flat but circular"? I can follow the rest of your argument though. $\endgroup$
    – Sathish
    Commented Dec 3, 2014 at 11:20
  • $\begingroup$ I mean that the concavity is due to the rotating station being a cylinder (I should have used that word instead of circular) $\endgroup$
    – user65081
    Commented Dec 3, 2014 at 11:44
  • $\begingroup$ @Wolfram: Sorry. I am still not able to get it. Concavity in my understanding means that a concave surface cross section (cut along a plane of symmetry) is a concave function (say like a parabola). What is the surface that is being concave here? If it is the space station floor, why it is not flat? In other words, why a flat floor of space station at rest becomes curved when spinning? $\endgroup$
    – Sathish
    Commented Dec 3, 2014 at 11:53
  • 1
    $\begingroup$ it is not curved because it spins, but the reverse, the cross section is made circular so that g is the same at every point on the floor. If you do not make it circular, but flat, then g will change with the horizontal position. In an actual space station I think they make the floor plygonal, it the diferences in g are not that large to justify an annoying circular (cilindrical) floor $\endgroup$
    – user65081
    Commented Dec 3, 2014 at 12:10
  • $\begingroup$ Just to make this more concrete, some hypothetical station designs do have partially flat floors. Take like 12 individual modules, string them in a circle, and rotate this. Since the walls of the modules were originally flat, the floor is also flat. But walking to one end is like climbing a hill. $\endgroup$
    – Alan Rominger
    Commented Dec 3, 2014 at 12:12
1
$\begingroup$

what does the commentator mean by concavity of the floor?

He or she means that the surface you walk on is in fact the inside of a cylindrical surface. Like a very large version of the inside of a wedding-ring. The curvature of this surface can be measured.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ This Skylab video youtube.com/watch?v=S_p7LiyOUx0 illustrates the process. The concavity is measured with a bar laid along the direction the astronaut is running. $\endgroup$
    – DJohnM
    Commented Dec 3, 2014 at 15:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.