7
$\begingroup$

I've landed in a physics debate amongst bike mechanics. In a typical bicycle hub you have a simple bearing; the cups are set in the hub, the race (cone) threads onto the axel and there are just loose ball bearings in between (no ball retainer). When properly adjusted, there is no play in the system, and the axel turns smoothly.

I imagine this is an elementary question, but when you put weight on the axel (rider on the bike) is the force concentrated on the bottom of the cone, or is it evenly distributed to all of the balls around the cone? What forces are at work in a bicycle hub?

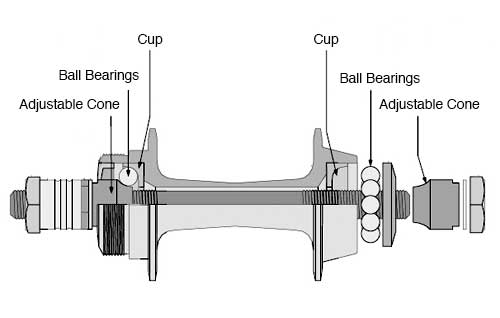
hub image

I made this image for the sake of convenience if anyone feels so inclined to be awesome and use it to make a diagram/illustration:

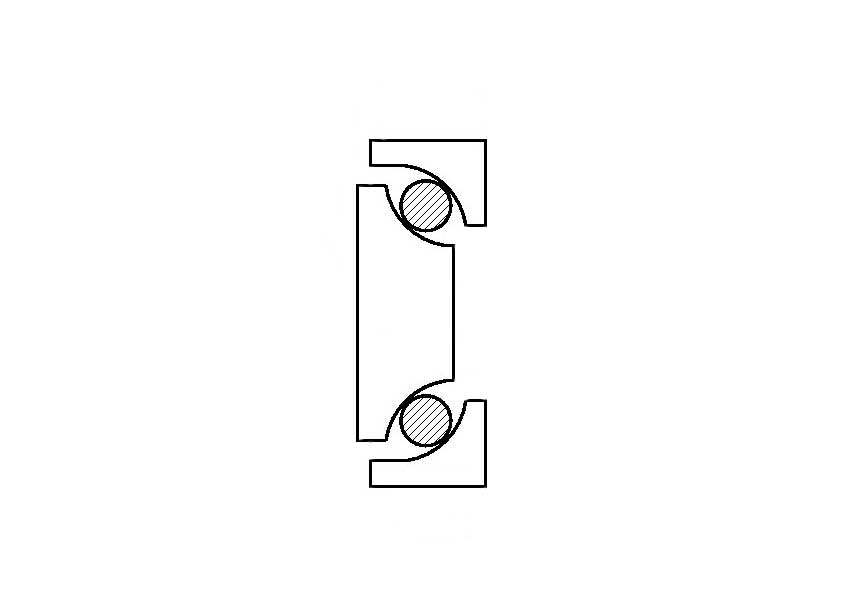
For illustration purposes?

Exceptionally illustrated answers will be worthy of additional bounty (more up-votes means more bounty to give :) I will offer a bounty of everything I have for an exemplary answer to this question).

Improve this question
$\endgroup$
0

3 Answers 3

Reset to default
4
+100
$\begingroup$

The distribution of force on the ball bearings, and therefore inner, and outer races of the bearing depend on the Adjustable tension. This tension preloads the bearings. First consider the case where the bearing is supporting no weight but the cones have been tensioned.

Bearing pre-load free body diagram

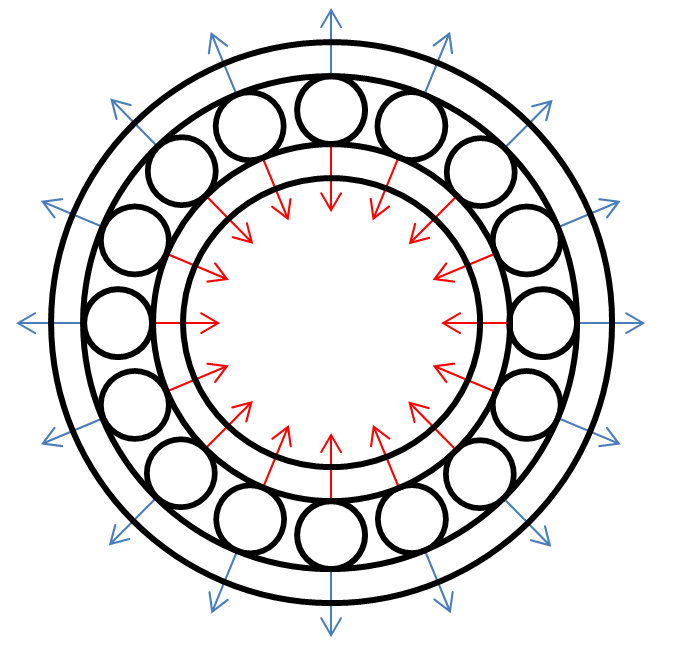
The tension from the nut compresses the bearing axially, compressing the balls. Viewed along the axis the loads from the ball would be evenly distributed across the balls and each load would intersect the center axis.

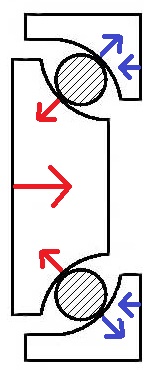
Axial view of Bearing pre-load free body diagram

When a load is applied to the bearing the inner race attempts to displace within the outer race, however the bearings get in the way, slightly relocating changing their contact angle and either increasing or decreasing the radial component of their force depending on the direction of the displacement. The direction of the loads would still remain intersecting with the center axis.

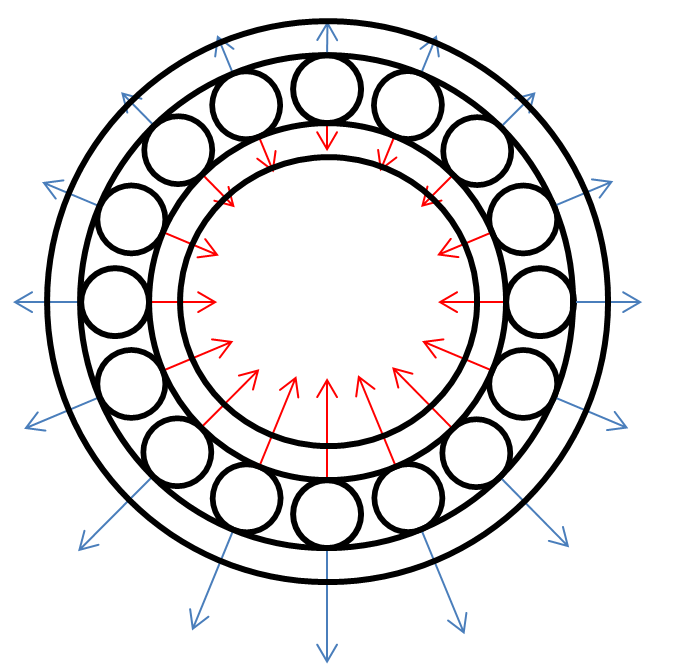
Axial view of Bearing loaded free body diagram

The net force on the inner race from the bearings is now upwards, balancing the downward load provided by the frame via the axle.

Notice that the force reduces on the upper ball bearings. If the bearings were not tightened enough to provide sufficient pre-load force on the bearings then this force would drop to zero and the ball bearing would be loose, potentially vibrating and causing rapid wear.

Regarding forces in the hub: The outer race is what makes contact with the hub, so the hub must provide the pre-load for the bearings as well as the lifting force. The pre-load from the bearings acts as a compressing force along the axis of the hub. The lifting force is applied on the outer surface of the outer-race as well as some tangential force on the outer surface. The reaction force of the bearing pushing down on the hub is then balanced by a change in spoke tension very similar to the change in ball compression inside the bearing.

If you're curious about the load distribution on an individual ball bearing. The load distribution is not actually all concentrated at a single point as that would create infinite stress ripping through the ball. The force is distributed over an area, and the pressure within that area approximates a paraboloid.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Don't suppose you have any references you can add to this answer? $\endgroup$
    – ShemSeger
    Commented Dec 9, 2014 at 16:19
  • $\begingroup$ Unfortunately my kinematics of machinery class didn't even use a textbook. But I'll see if I can dig something up. $\endgroup$
    – Eph
    Commented Dec 9, 2014 at 16:24
  • $\begingroup$ I added a couple references, but they don't really address what I think is the heart of your question "what is the load distribution across the bearings?" I saw some research papers on the topic, but I don't want to site something you'd have to pay for. I also saw some evidence that not all bearings require a positive force on all the balls during opertion (ei only some of the balls would be holding the weight while the others would be coasting). However, I believe in the instance of the loose ball bearings, that in order to have smooth operation, all bearings would have at least some load. $\endgroup$
    – Eph
    Commented Dec 9, 2014 at 17:17
  • $\begingroup$ If you could go ahead and add the citations you found I'd appreciate it. I work with the administration of an academic library, I don't have to pay to read research papers. $\endgroup$
    – ShemSeger
    Commented Dec 9, 2014 at 18:05
  • $\begingroup$ Sadly, having left academia, I no longer have free access. So I'd just be guessing from the abstracts. I did find a NASA paper discussing radial ball bearings, rather than the angular contact ball bearings shown in the drawings. It does talk about reduced life for loose bearings though, so I think the results could still be somewhat applicable. I might be able to find you a better paper if I knew exactly what you're looking for. $\endgroup$
    – Eph
    Commented Dec 9, 2014 at 18:34
1
$\begingroup$

All bearings have what is called a load zone. That is a distribution of loaded rolling elements concentrated along the line of force (load). The most loaded ball is in the center and the loading drops off roughly parabolically away from there. So the load is not concentrated, but distributed not evenly though.

The axial play affects the distribution. If there is axial play the distribution in more or less concentrated on a few balls. With no axial play but not press fit either the distribution is roughly 180°. With pressfit the distribution can reach all the balls, with a load zone approaching 360° around. Steering bearings are like that.

Improve this answer
$\endgroup$
0
$\begingroup$

The balls will feel a force only on the point of contact, not uniformly. In priciple, it is correct thatthe bottom part of the adjustable cone will excert more force on teh lower bals tan on the top ones, dues to the fact that the bottom balls, feel the added force of both the person and the part of the cone abobe it. However, for all pratical purposed, the weight of the cone is negligible compared to that of a person, so that you could say that the forces on the top and bottom balls are practically the same.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.