"Falling upward" - how far you have to be from Earth to start falling to the Moon?

Question

Talking about gravity with my 9 y/o she asked when do we start "falling upward" to the Moon. What is the distance at which the Moon's gravitational attraction is higher than that of the Earth and thus makes you accelerate towards it, and how to get to that answer?

Answer 1

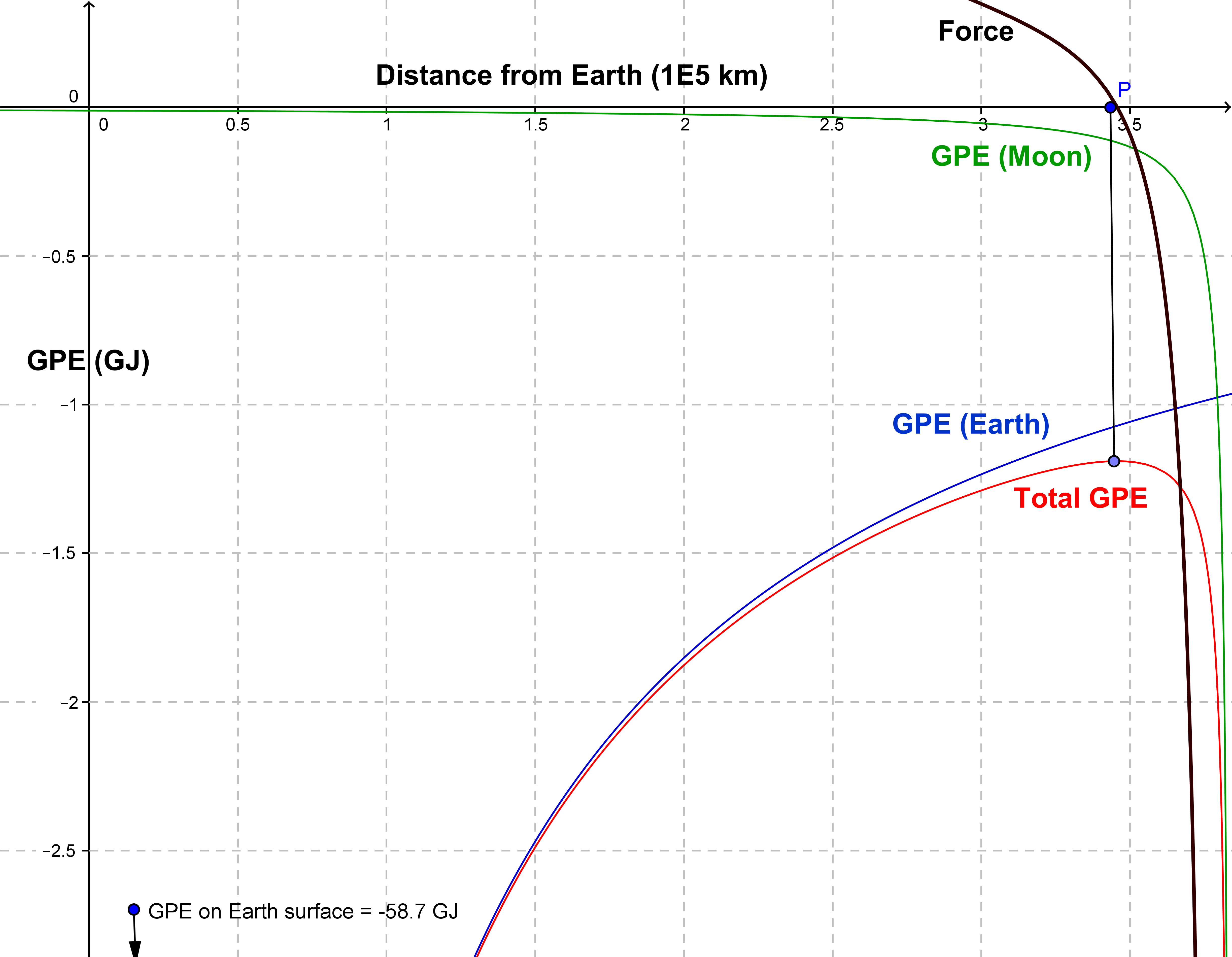
The main plot below shows the potential energy of a mass in the Earth-Moon system under the unrealistic assumption that the system is not rotating.

i.e. This mirrors (at present) all but one of the 4 answers given, in assuming that this point is defined where the gravitational force on a mass due to the Earth and the Moon are equal and opposite (i.e. at the point where the total potential energy [red curve] is at a maximum, because force is of course the gradient of the potential, and I show this as a black line).

This is wrong, because it neglects the centrifugal potential caused by the orbital motion. Whilst the inclusion of this potential only changes the third significant figure of the amount of energy it takes to get something to the moon, it moves the point at which a co-rotating object starts to fall towards the moon significantly closer to the earth.

In the plot I used the mean Earth-Moon distance of 384,000 km. The point P where the force (neglecting centrifugal force) is zero is about 344,000 km.

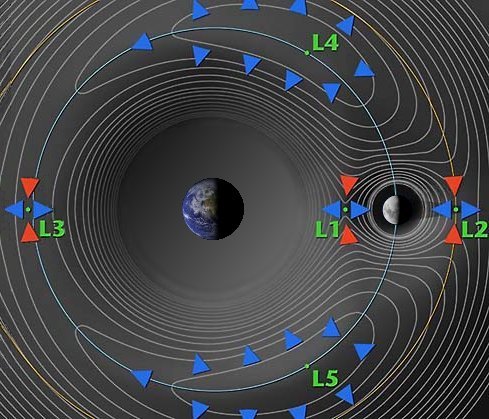
Including the centrifugal potential (see the plot below: credit NASA) in the co-rotating frame and calculating the "L1 point" where the potential is actually maximised, is described here and involves solving a quintic function. However as the moon mass is much less than the Earth mass we can use the "Hill sphere" approximation, that the L1 point is separated from the moon by $r= R (M_2/3M_1)^{1/3}$, where $R$ is the Earth-Moon separation and $M_2/M_1$ is the Moon/Earth mass ratio. Putting in the numbers gives $R-r=$323,000 km, so this is not a small correction.

Note however that a body that passes through the L1 point that was previously orbiting the earth cannot simply fall onto the moon. It has too much angular momentum. The L1 point marks the point where it stops orbiting the earth and starts orbiting the moon. In that sense it is "falling" towards the moon.

Edit: Final complications are that (i) the Earth-Moon distance is not constant and so neither is the L1 point. In fact a better wat to quote the solution is that gravitational force balance is achieved at 90% of the Earth-Moon distance, whilst the distance at which the object falls towards the moon is about 84% of the Earth-Moon distance. (ii) The Earth-Moon system is not isolated and the gravity of the Sun plays a role.

I also note that this was part of the mission concept for the SMART-1 mission to the moon, where an orbit was designed so that the satellite spiralled outward from the Earth to the L1 point and was then captured by the moon. It "passed through a position 310,000 km from the Earth and 90,000 km from the Moon in free drift".

Including the effects of the centrifugal potential.

Answer 2

Set the forces on the test particle from the Earth and Moon equal: $$F_E=F_M$$ $$G\frac{M_EM_{\text{ test particle}}}{R_E^2}=G\frac{M_MM_{\text{ test particle}}}{R_M^2}$$ The $G$s and $M_{\text{ test particle}}$s cancel, leaving you with $$\frac{M_E}{R_E^2}=\frac{M_M}{R_M^2}$$ but you know that $R_M$, the distance between the test particle and the Moon, is the distance between the Earth and the Moon minus the distance between the test particle and the Earth ($R_E$). We simplify, and get $$\frac{M_E}{R_E^2}=\frac{M_M}{(D_{E \to M}-R_E)^2}$$ and then $$D_{E \to M}^2-2R_E \times D_{E \to M}+R_E^2=R_E^2\frac{M_M}{M_E}$$ This simplifies to $$\left(1-\frac{M_M}{M_E} \right)R_E^2-2R_E \times D_{E \to M} + D_{E \to M}^2=0$$ You can solve this equation to get: $$R_E=\frac{D_{E \to M}}{1+\sqrt{\frac{M_M}{M_E}}}$$

---

$F_E$ is the force of the Earth on the test particle.

$F_M$ is the force of the Moon on the test particle.

$M_E$ is the mass of the Earth.

$M_M$ is the mass of the Moon.

$G$ is the universal gravitational constant.

$M_{\text {test particle}}$ is the mass of the test particle.

$R_E$ is the distance from the test particle to the center of Earth.

$R_M$ is the distance from the test particle to the center of the Moon.

$D_{E \to M}$ is the distance between the Earth and the Moon.

Answer 3

Earth is about 100x more massive than the moon, and since $F \propto M / r^2 $, the distance from Earth to the astronaut would have to be about $\sqrt{100}$ = 10x further than from the moon to the astronaut. Therefore, the astronaut falls "up" about 90% of the way to the moon.

[The earlier answers go a lot more into detail (and are more technically accurate), but it's worth a quick approximation, as few nine-year-olds are going to understand Lagrangian points.]

Answer 4

At Lagrange point L1. Specifically for Earth-Moon L1, these calculations show 326054 km.

Answer 5

To calculate this by yourself, you need to know that gravity force exerted on an object (for exapmle You) is equal to $F=GMm/r^2$, where $G$ is gravity constant, $M$ is the mass of the big object ($M_m$ for moon, $M_e$ for earth), $m$ is the mass of small object. $r$ is the distance from the center of the mass.

Now you need to know masses of earth and moon and distance between them. Point in which earth and moon are attracting you with the same force (after which you will fall on the moon) is given by those equations: $GM_m m/r^2_m=GM_e/r^2_e$ and $r_m+r_e=\text{Distance Between Moon And Earth}$

Note that beyond this point, Moon atracts you better than Earth so You'll start falling.

In first equation you can replace one of $r$'s with $\text{Distance Between Moon And Earth}-\text{another R}$ Moreover gravity constant $G$ may be reduced.

All needed data you can find on Wikipedia

Note that this is simplified solution that assumes that you are going straight up to the Moon.. Moon and earth are in constant movement, so you need to make some better and more complex calculations in case of spaceships.

Answer 6

Just use an equation derogating from the two forces which pull the objects (universal gravitation)to get the equilibrium point, something like (already simplifyed): M/d^2 = m/(384000000 - d)^2

Where M is the mass of earth, m the mass of moon and d the distance from earth. As d gets bigger than this value, you start falling into the moon

I get a value of roughly 3.4 10^8 metres (but I'm not using my calculator so calculate again, sorry!)

Answer 7

The distance I got was 346 084km. Here are the maths I used:

• ($E_m$) Earth mass = $5.9736\times10^{24}$ kg
• ($M_m$) Moon mass = $7.3477\times10^{22}$ kg
• ($D_{em}$) average Earth-Moon distance = 384 467km
• ($G$) gravitational constant = $6.67384\times10^{-11}$
• ($W$) my weight = 85kg
• ($D_{fe}$) distance from earth = ?

The attraction force between two objects is calculated by $$F=\frac{G M_1 M_2}{d^2}$$ So the attraction force from earth is $$F_e = \frac{G Em W}{D_{fe}^2}$$ and the attraction force from moon is $$F_m = \frac{ G M_m W}{(D_{em}-D_{fe})^2}$$

I did a script that started with $D_{fe} = 1$ km, calculated $F_e$ and $F_m$, and if $F_e$ was higher than $F_m$, $D_{fe}$ would increase by 1km and the forces would be calculated again. At $D_{fe}$ = 346 084km, $F_e$ is 282 922.71N and $F_m$ is 282 923.03N and that is the point where the attraction force from the moon will be stronger than the one from earth.

Answer 8

Here's how I went about solving this problem:

1. The force on the object (mass m) from the Earth (mass Me) has to be equal to the force from the Moon (mass Mm).
2. The distance of the object from Earth is R, and so with Rem being the distance between Earth and Moon, then the distance from the Moon is Rem-R

Newton's law: G.Me.m/R^2 = G.Mm.m/(Rem-R)^2 Solve for R: R=Rem(Me-(sqrt(MeMm))/Me-Mm

With this mathematics, I get a result of 346,019 km (varies depending on the values for Me, Mm and Rem).