0
$\begingroup$

As a newbie in physics I imagine this device and I would like to know why it can't increase its energy.

Note I study the sum of energy during one second (or less) after set friction ON.

The device:

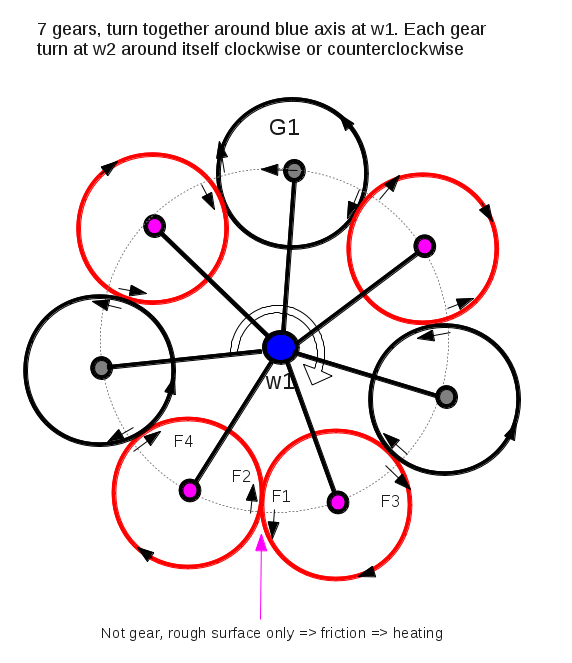
I imagine 7 gears in rotation around blue axis in clockwise. Each gear has a mass $m$. In the same time, gears are turning at $w2$ around themselves. One clockwise next one counterclockwise like gears. But like I have 7 gears (odd number), 2 gears rotate in the same direction (2 red gears at bottom). I know 2 red gears at bottom can't turn like gears but I don't want gears but friction, it's possible to imagine a part of gear with rough surface only and study the device during a little while. So, between these gears I would like only friction. This friction give heating and forces $F1$ and $F2$, others gears give forces too, $F3$, $F4$ etc. I try to draw all forces but maybe I'm wrong.

G1 can have mass or not

To draw forces, I'm starting from forces F1 and F2 and after I draw forces at right and at left in the same time, until I arrive at G1 (I'm not sure).

I define, $F = | F_1 |$, $r$ the radius of a gear, $t$ the time.

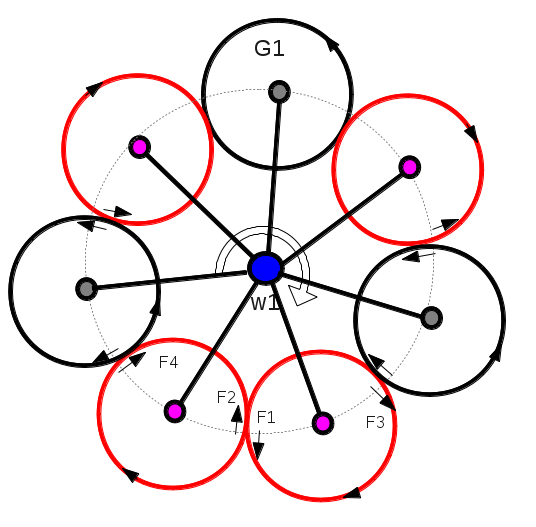
1/ G1 has mass. I have a counterclockwise torque on blue axis, the energy from this torque is $E_1=-2Frtw_1$. I have a torque on gear G1, the energy is $E_2=+2Frtw_2$. $E_1 + E_2 != 0$.

enter image description here

2/ G1 don't have mass. The same conclusion than 1/

enter image description here

3/ With 2 red disks only (not others gears), there is the same torque on disk, a torque on blue axis, but there is heating too. The heating seems to be an extra energy if I compare to 1/ and 2/. The work from heating is $E_3=2Frt(w_1-w_2)$, here the sum of $E_1 + E_2 +E_3 = 0$

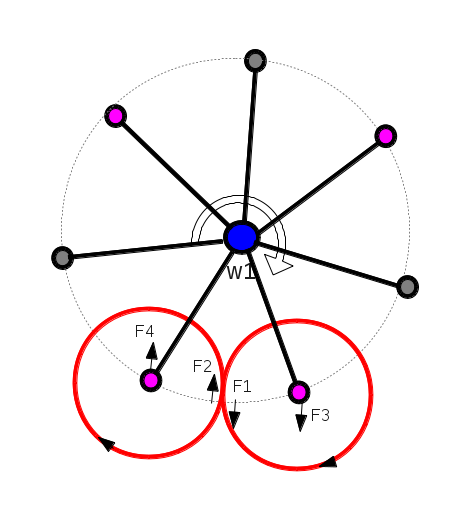
enter image description here

So, it seems in cases 1/ or 2/ the sum of energy is not constant.

The cycle is:

  1. Friction is OFF. I launch gears at $w1$ and $w2$ without friction between red/red gears, this step need the energy $E$.
  2. I set friction ON between red/red gears
  3. I count all energies (heating too), $E$ must be constant. I'm interesting about the transcient analysis when there is friction between red/red gears. From time $t=0$ to $t=t_x$, with $t_x$ very small.

How $E$ could be constant?

Note:

I need to press 2 red gears at bottom for have friction, but I don't drawn these forces.

I guess no friction elsewhere than red/red gears surface.

I don't believe in perpetuum mobile but I imagine devices, and like puzzles to resolve, I try to understand how the sum of energy is conserved, like that I learn physics in the same time. It's like a game for me.

Improve this question
$\endgroup$
14
  • 2
    $\begingroup$ "I guess no friction elsewhere than red/red disks surface." - if you have enough frictionless surfaces, it's easy to have a system that doesn't lose energy. It can't generate any, though, ever. $\endgroup$
    – John Dvorak
    Commented Nov 11, 2014 at 16:07
  • $\begingroup$ How exactly are the gears kept in motion? If they are driven by an external force, said force will provide energy into the system. If not, they will stop moving soon as their kinetic energy turns into heat. $\endgroup$
    – John Dvorak
    Commented Nov 11, 2014 at 16:10
  • $\begingroup$ I think you need a strong review on how touching gears rotate. The animation at the top of the Wikipedia page on Gears gives an excellent reason why your diagram seems to be inaccurate. $\endgroup$
    – Kyle Kanos
    Commented Nov 11, 2014 at 16:17
  • $\begingroup$ I added an image, you can understand like that ? @Jan: I would like to understand the transcient analysis, from start to tx, with tx small $\endgroup$
    – Sx7
    Commented Nov 11, 2014 at 16:23
  • 2
    $\begingroup$ The two red wheels at the bottom are turning against each other. If they have friction, them all the kinetic energy turns into heat energy. If they are gears, then the wheels can't even start. $w_1$ has nothing to do with it. $\endgroup$
    – Mike Dunlavey
    Commented Nov 11, 2014 at 17:40

1 Answer 1

Reset to default
1
$\begingroup$

Even if you assume that there are no loses by friction, $\omega_1$ will slow down by conservation of angular momentum. So the total kinetic Energy will diminish. In terms of forces, the orbital angular speed ($\omega_1$) will slow down because, even if there is no net external torque, there are internal forces and torques. To see this, look at the black disk at the top. Both friction forces will have a horizontal component to the left, so as each disks increases $\omega_2$ clockwise, it feels a force/torque opposite to the direction of $\omega_1$.

Third case: In this case the internal torques actually speed up $\omega_1$ until the equilibrium situation is reached (so all $\omega$s are the same, there is no relative motion between the red gears, and they rotate with the same point always facing the blue revolution center). Angular momentum is still conserved and Energy is constant. The energy lost by the two disks ($\omega_2$ becomes zero), compensates exactly the increase in $\omega_1$ plus the heat dissipated).

Improve this answer
$\endgroup$
4
  • $\begingroup$ I added information, imagine 2 red disks only at bottom (no others gears), there is torque on blue axis, torque on disk and heating. But with all gears with mass, each time I draw forces, a torque on blue axis is cancel by a torque on a disk. It's like with 2 disks there is more energy from heating. $\endgroup$
    – Sx7
    Commented Nov 11, 2014 at 19:39
  • $\begingroup$ I am not sure to understand which torque on the blue axis is cancelled by which torque on the disk. Can you give me a specific pair (such as torque from F1 cancels torque from F2, or whatever you think is the case)? $\endgroup$
    – user65081
    Commented Nov 12, 2014 at 3:11
  • $\begingroup$ Plus what is the initial sign of $\omega_2$ at the start? they look clockwise as $\omega_1$? Am I correct? $\endgroup$
    – user65081
    Commented Nov 12, 2014 at 3:19
  • $\begingroup$ You're rigth it's ok ! $\endgroup$
    – Sx7
    Commented Nov 12, 2014 at 10:50

Not the answer you're looking for? Browse other questions tagged or ask your own question.