Suppose a child were to ask you why a tetherball (picture below) seems to speed up as it wraps around the pole. How would you explain this to them? Certainly you wouldn't say something like, "Angular Momentum is conserved throughout the process."
Favor will be shown to for creativity and simplicity.
Start with the force felt while holding weights and spinning with arms at full extension. Ask if it is easier or harder than when not spinning. Here you are forcing the weights to move from a straight line and to go in a circle, the force has to be felt all the time to keep pulling the weights into a circle. To make the circle smaller requires even more force (intro to the idea of work). The line on a tether ball winds up on the pole and this is just like pulling your arms in while spinning weighs. Compare to a gradual turn in a car versus a sharp cornering.
Ask what is meant by speed? Is there a difference between how many times it goes around in a minute and how fast, say, a bird would have to fly to keep up with it? Ask if the weights are really going faster or just more RPMs because the circle is smaller. Does the ball get dangerous as the circle gets smaller? Does it move dangerously fast and cause injuries? By the way, does a skateboard speed up when you turn a corner? Wouldn't that mean you can go faster and faster on a skate board without a motor or going down hill?
Try some reductio ad absurdum. If it is speeding up, would it break the sound barrier if the pole allowed a small enough radius? Would it approach the speed of light if the pole and ball were microscopic and the line started out the same length as the regular tether ball? It is great if kids learn to ask these kinds of questions early. "What can I ask that assumes my idea is true and leads to an impossible conclusion or one obviously false by observation?"
I think you have to use, at a minimum, the concepts of 1) force, 2) force changes speed 3) one force can be imagined as two different forces into two perpendicular directions. Staring with that, you can watch the pole from the top. I'll leave the details to you, an concentrate in the basics steps, using a figure like the one below.
a) The cord always makes a force towards the center
b) Look at the position of the ball just before it reaches the "horizontal" position. Then, one component of the force changes the speed in the vertical direction, the other in the horizontal direction. Then, the speed "upwards" increases and the speed "outwards" decreases.
c) When the increase in speed upwards matches exactly that of the increase inwards (or decrease outwards), then the movement looks circular because the net effect is a change in total speed direction, but not total magnitude.
d) If you push the string inwards (the details doesn't matter, you can either stay at the center and actually push it in, or let it wrap around the pole), then the force increases and the two components no longer match each other to keep the total speed constant. The balance is lost and the ball will not move in a circular path, but will move both, inwards and upwards in a way faster than when the motion was circular. So the ball motion "upwards" becomes faster than before, and also there is a net motion inwards and the ball gets closer to the center.
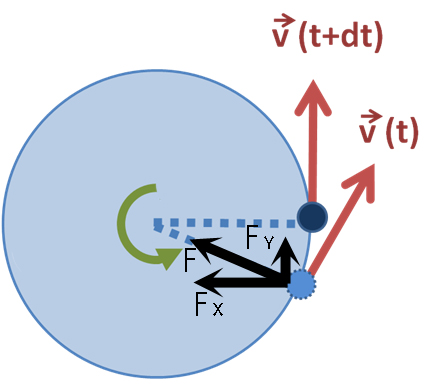
Well, after I wrote this, I realize that the picture given is still a little inaccurate and the explanation more complex than I was hoping. But I hope it still could help.
Warning: Copyright infringement. Part of the the picture was stolen from Wikipedia without permission. Do not attempt this at home, Federal charges could apply.
Since $v=r\omega$ Where $v$ is velocity and $r$ is radius and $\omega$ is angular velocity
So $\omega=v/r$
This equation shows that if $r$ decreases $\omega$ increases
Did not notice the intention of the question (updating with other answer)
Tell the child to throw a ball ($m$) on the groud from a given height $h_1$. This will take an amount of time $t_1$ (or speed $u_1$). Now tell to throw the same ball $m$ again from another height $h_2 < h_1$. This will take time $t_2 < t_1$. Why?
edit after comment:
Technicaly the (terminal) velocities ($v_1$, $v_2$ will be related as $v_2 < v_1$), by uniform accelerated motion.
However if one wants to use the velocity analogy, one can use the negative velocities (meaning not the terminal, but assumed initial velocities which get to zero when ball hits the ground). In this sense $v'_2 > v'_1$ ($v'_2=-v_2$, $v'_1=-v_1$)
Or if one wants to use a rapidity analogy one can use the magnitudes $t^{-1}_2 > t^{-1}_1$ (this is just an analogy, the magnitudes used can be changed accordingly)
The ball ($m$) is the same, what changed was the height, so less height, more quickly (or more rapid) for same ball.
Now when this is understood, tell the child to wrap this experiment in a circle(s) (of different radii). Then you have the ball in the picture (and an tentative explanation of conservation of angular momentum).
If we observe the path of the ball as radius reduces, we can see that the path followed is not circular. So between the radius and tangent, angle formed will not be 90 degrees..
This creates a component of tension along the path of the ball thus increasing its velocity
