An exercise about angular momentum in "The Mechanical Universe" (Frautschi)

Question

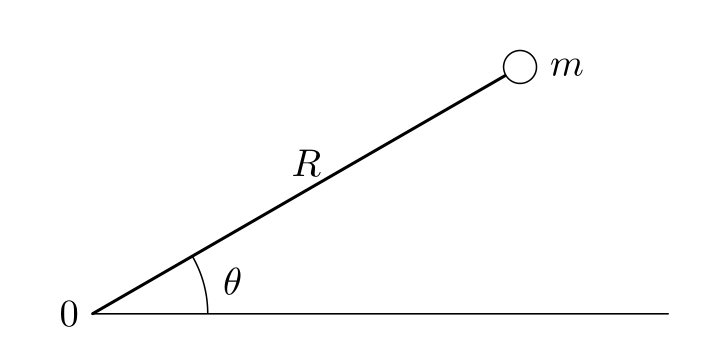
I'm reading through "The Mechanical Universe" and I'm stuck with one exercise in the chapter about angular momentum. It goes like this:

A particle of mass $m$ is at the end of a rod of negligible mass and length $R$.

1. If at any instance the rod makes an angle $\theta$ with the horizontal as it falls, calculate the torque about $0$ acting on the mass as it falls.
2. If the mass is started from the vertical position ($\theta=\pi/2$) with initial speed $v$, find its angular momentum about $0$ as a function of $\theta$.
3. Use your results to find the angular speed $d\theta/dt$ of the mass.

(FWIW, this exercise belongs to a section where angular momentum conversation hasn't been introduced yet, so I guess I'm not supposed to use that. Not that I knew how that would help...)

For the first point, I think we have the position $\vec{\mathbf r}$ of the mass and the gravitational force $\vec{\mathbf F}_g$ acting downward, so the torque is $\vec\tau = \vec{\mathbf r}\times\vec{\mathbf F}_g$ with magnitude $\tau = mRg\cos\theta$ as the angle between $\vec{\mathbf r}$ and $\vec{\mathbf F}_g$ is $\theta+\pi/2$. This makes sense as the torque would be zero for the vertical position and it would increase as the mass comes nearer to the bottom

But I have no idea how to address the second point. I guess "with initial speed $v$" means that the velocity is directed to the right (in the picture). But how does this affect the motion of the mass? What forces, apart from gravity, are acting on $m$? Is it the tension of the rod pulling the mass towards the center?

I was also wondering what would happen if there were no gravity: Let's suppose the picture is a view from above where $m$ is attached to the rod (which is fixed at $0$) and everything happens on a frictionsless table. If the mass were started with some initial velocity what would the outcome be? Would it circle around $0$ forever? That would contradict conservation of momentum, wouldn't it? But if it stopped moving, wouldn't that contradict conservation of energy?

Answer 1

For this problem to make any sense, you need to assume that the rod rotates about a horizontal axis at point, O. Then your expression for the torque is correct. You have the torque as a function of position, but to get the change in angular momentum, you would need to integrate the torque over time. Instead, use conservation of energy to get the speed of, m, as a function of angle. From that you can calculate the angular momentumm, and the angular velocity. In the absence of friction, this system conserves energy, but linear forces from the axle prevent conservation of momentum, and a torque from gravity causes a change in angular momentum.

Answer 2

Following R.W. Bird's hint, I'm coming to the following conclusions:

We already had from part 1 the magnitude $\tau = mRg\cos\theta$ of the torque.

In part 2, conservation of energy gives $$\frac12mv^2+mgR = \frac12mv_\theta^2 + mg(R\sin\theta)$$ where $v_\theta$ is the speed at angle $\theta$ and the left hand side is the situation at the start. This can be solved for $v_\theta = \sqrt{v^2+2gR(1-\sin\theta)}$. This seems to make sense for $\theta=\pi/2$ as well as for $v=0$.

$v_\theta$ must be perpendicular to the rod, so the magnitude of the angular momentum as a function of $\theta$ should be $L(\theta)=mRv_\theta$.

For part 3, I can differentiate $L(\theta)$ with respect to $t$ (treating $\theta$ as a function of $t$) and compare with part 1:

\begin{aligned} mRg\cos\theta &= mR2gR\frac{d\theta}{dt}(-\cos\theta)\frac12(v^2+2gR(1-\sin\theta))^{-\frac12} \\ \frac{d\theta}{dt} &= -\frac1R\sqrt{v^2+2gR(1-\sin\theta)} = -v/R \end{aligned}

It again makes sense that the angular speed is proportional to the speed. The minus sign comes from the fact that the angle $\theta$ decreases when the mass falls.