4
$\begingroup$

We know from 2nd law of motion that $$\vec{F} = \frac{d\vec{p}}{dt}.$$

Now, a rate of change can be instantaneous. So, rate of change of momentum is instantaneous. But I doubt how can there be change of momentum at an instant?

But when it comes to impulse, it always acts for a certain time, however small (infinitesimal) it might be. If rate of change of momentum is instantaneous and force is instantaneous, why can't the impulse be instantaneous? What is the cause?

Can anyone also tell how can there be change of momentum at an instant?

Improve this question
$\endgroup$
8
  • 3
    $\begingroup$ If the rate of change is instantaneous, the derivative is infinite, and the force is infinite. There are no infinite forces. $\endgroup$
    – mmesser314
    Commented Nov 4, 2014 at 12:02
  • 2
    $\begingroup$ Instantaneous doesn't mean $t=0$. $\endgroup$
    – user49111
    Commented Nov 4, 2014 at 12:08
  • 1
    $\begingroup$ @mmesser314: So are you telling instantaneous rate doesn't exist? $\endgroup$
    – user36790
    Commented Nov 4, 2014 at 12:21
  • 1
    $\begingroup$ @imakesmalltalk: Instantaneous velocity, for instance, is believed to apply to a point on a graph, which is interpreted as $\Delta_t=0$. $\endgroup$
    – bright magus
    Commented Nov 4, 2014 at 12:24
  • 1
    $\begingroup$ Google "Green Function" $\endgroup$
    – Carl Witthoft
    Commented Nov 4, 2014 at 12:52

4 Answers 4

Reset to default
12
$\begingroup$

Maybe this graph helps to illustrate the point. Consider a constant force that is applied for a finite interval $\Delta t$. The slope in the $p$ graph at any given time is $\frac{dp}{dt}$. Now, in order to have a step in $p$ (an instantaneous impulse), it is necessary to get an infinite slope, hence an infinite force is required which is physically not possible.

enter image description here

Having said that, there are some situations where a having an infinite force for an instant is a good model of a real situation. For example, hitting a ball with a hammer. In this case, the force is not really infinite nor instantaneous but in good approximation it can be seen like that. In such cases the force is mathematically modeled by a Dirac delta which is just that; an infinite force applied instantly. This case would be like taking the limit of the above picture where $\Delta t \rightarrow 0$ and $F_0 \rightarrow \infty$ while keeping the area $F_0 \Delta t$ constant.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Perhaps it would hammer the point home even more if you eliminated the lines going up and down on the $F$ and $\frac{dp}{dt}$ graphs? $\endgroup$
    – jpmc26
    Commented Nov 4, 2014 at 23:45
  • $\begingroup$ @jpmc26 I just tried your suggestion but it is difficult to keep the visual cohesion between the different parts of the line. $\endgroup$
    – Asaf
    Commented Nov 5, 2014 at 1:05
5
$\begingroup$

Consider a superball falling toward a hard tile floor. The ball will bounce rather nicely. How to model this collision?

If you look very closely at the bounce, a normal force from the floor starts acting on the ball when the bottom of the ball hits the floor. At this point in time, the top of the ball doesn't "know" that the bottom of the ball has hit the floor. That information propagates upward as a finite velocity pressure wave in the ball. The top of the ball keeps falling downward for a while. The ball gets compressed, rebounds, and flies back upwards. Some energy is lost as the ball is compressed and then relaxes back to its round shape.

One way to model this is to ignore all those details of the bounce. Since the duration is so very short, the bounce can be modeled as an instantaneous change in momentum determined by the ball's coefficient of restitution. This is a simplification that intentionally ignore the details of the bounce. While the maximum force is very high compared to the weight of the ball, the force is never infinite, and the change in momentum is not instantaneous.

The same applies to any application where one models forces as impulsive. It's just a model, a simplification that lets one ignore the details. The force is never truly infinite, so the change in momentum is never truly instantaneous.

Improve this answer
$\endgroup$
3
$\begingroup$

All objects interact via a force that has a finite range. Ultimately this would be one of the four forces electromagnetic, strong, weak and gravity. Since these forces change smoothly with distance the rate of change of momentum can never become infinite.

Improve this answer
$\endgroup$
1
  • $\begingroup$ True, but that doesn't address the reality that we often use mathematical models which do have either IIR or FIR (Infinite/Finite Impulse Response) functions. $\endgroup$
    – Carl Witthoft
    Commented Nov 4, 2014 at 12:53
3
$\begingroup$

but I doubt how can there be change of momentum at an instant?

It isn't clear to me what you mean here. Do you mean you doubt that momentum can be discontinuous?

If so, then your doubt is justified. Classically, momentum cannot actually be discontinuous at some time $t_0$ since that would imply an actually infinite force acting at $t_0$.

If you're trying to say something else, please clarify your question.

But when it comes to impulse, it always acts for a certain time,

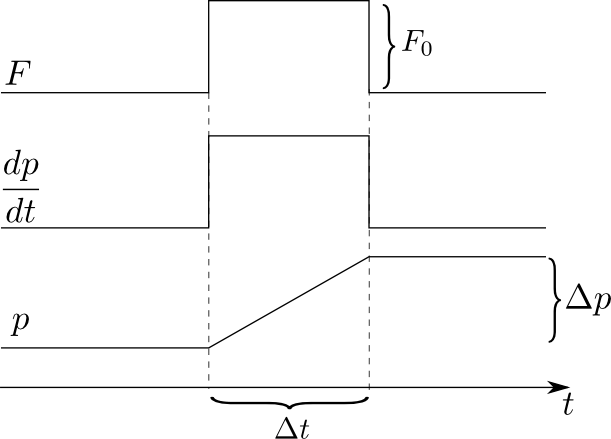
It isn't clear to me what you're thinking here. An impulse does not act for a certain time, a force acts for a certain time.

When we integrate the force (with respect to time) over that certain time, the result is the impulse of the force acting for that time - impulse has time 'built in'.

So, for example, if a force acts over some time $\Delta t$ and we integrate the force over that time, the result is the time average of that force multiplied by $\Delta t$ and this is called the impulse produced by the force:

$$\mathbf J = \mathbf F_{avg}\Delta t $$

Can anyone also tell how can there be change of momentum at an instant?

If you mean a finite (rather than infinitesimal) change at an instant, this requires an actually infinite force which isn't physical. However, mathematically, we can abstractly describe such a force with a Dirac delta distribution which can be useful. For example, take a look at Green's functions.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Sir, I am not talking about Zeno's paradox ; instantaneous velocity ie. velocity at a certain instant :- since motion occurs at an instant, to quantify the fastness of motion, instantaneous velocity is used ie. if the body moved after that instant uniformly, then it would travel at that instantaneous velocity, right???.... $\endgroup$
    – user36790
    Commented Nov 4, 2014 at 14:00
  • $\begingroup$ @user36790, I'm not talking about Zeno's paradox either. Evidently, we are unable to effectively communicate (and this isn't the first time). $\endgroup$
    – Alfred Centauri
    Commented Nov 4, 2014 at 14:02
  • $\begingroup$ ...sir, I am not telling that you have told about Zeno. I was just describing how I described instantaneous velocity. I want to know sir, how can I describe instantaneous rate of change of momentum like that of velocity??? $\endgroup$
    – user36790
    Commented Nov 4, 2014 at 14:10
  • $\begingroup$ @user36790, the instantaneous rate of change of momentum is (Newtonian wise) proportional to the instantaneous rate of change of velocity. $\endgroup$
    – Alfred Centauri
    Commented Nov 4, 2014 at 15:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.