How to correctly resolve forces radially in vertical circular motion?

Question

I have always had problems at resolving forces and relating to Centripetal Force. Il show an example that I am stuck at right now. Please help me understand.

---

This is the diagram:

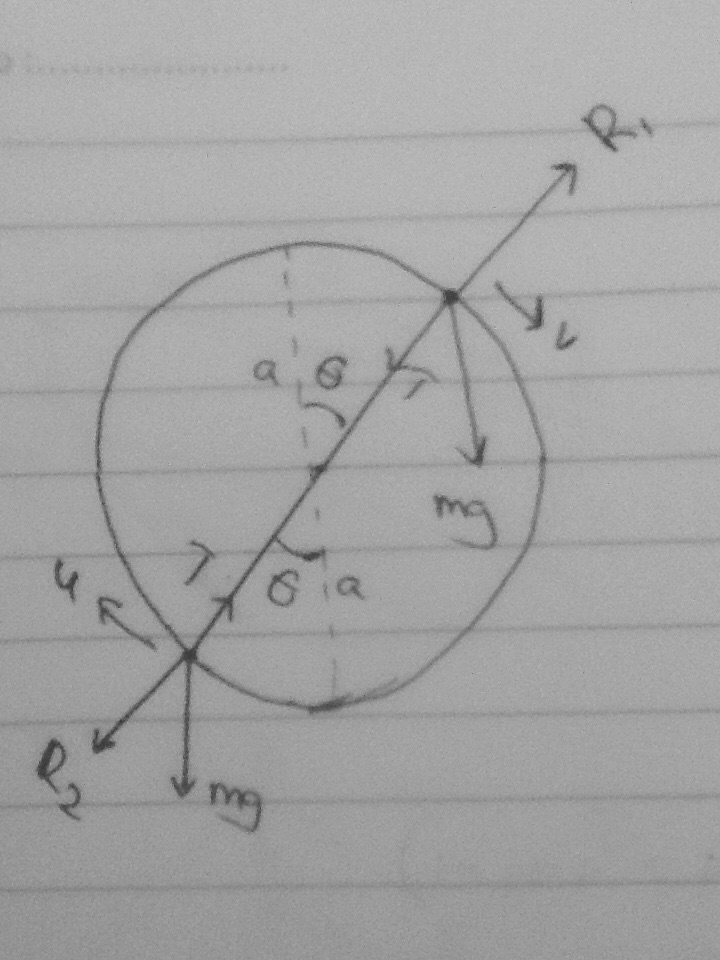
This is the scenario of a particle with mass $m$ at two different locations. A ball is released from the top of the circle and moves in a circular motion (in a clockwise direction)

---

Now what I wrote :

At the first location on diagram:

The resultant force towards the center= Centripetal Force

$$mg \cos \theta - R_1 = \frac{mv^2}{a}$$

This is correct the problem comes in next part

---

At the second location, I used the same concept:

Resolved towards the center:

$$mg\cos \theta+ R_2 = \frac{mu^2}{a}$$

To me this looks perfect, but it's wrong for a reason I don't know. Here is the correct answer :

$$R_2 = mgcosθ + \frac{mu^2}{a}$$

---

Please shed some light as to what's happening. How does one apply these correctly? And be satisfied that it is correct. This is only one example. I fail to get these correct all time.

Answer 1

First rewrite the equation as $R_2 - mg\cos \theta = \frac{mu^2}{a}$. The reason the signs are different is that here $R_2$ should point towards the center, unlike in your drawing. Gravity points outwards and so it appears with a minus sign.