0
$\begingroup$

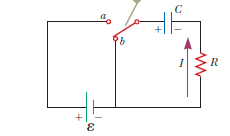
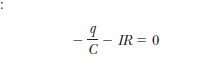enter image description here enter image description here

When the capacitor is fully charged and the switch as it B, how can we apply KVL at that loop to reduce the equation to (-IR)? Why is it negative? At time = 0 is the current not zero? And it will move from the plates so the resistance polarity should be reversed since it is power consuming why we do not say IR =q/c ?

Improve this question
$\endgroup$
1
  • $\begingroup$ Is there a sign error in one of your equations? Well, regardless, let's suppose that IR = q/c. This is mathematically equivalent to IR - q/c = 0. The equations are the same, just in slightly different formats. $\endgroup$
    – honeste_vivere
    Commented Oct 26, 2014 at 19:29

2 Answers 2

Reset to default
1
$\begingroup$

The total voltage along a closed circuit without a power source such as a battery is zero. If you analyze that loop counterclockwise, there will be a drop of voltage at the resistance, that is why it has a negative sign. But the charged term has the wrong sign, because the voltage increases from right to left across the capacitor. If it is not a typo, it means that they are considering q as negative (perhaps because the moving charges are electrons). This is non-standard, but you are correct, either q is negative or the sign is wrong

Improve this answer
$\endgroup$
0
$\begingroup$

The current comes out negative because of the direction you assumed for it. This is a nice self-correcting feature of Kirchhoff's Laws. With the capacitor fully charged and then isolated from the battery, it acts as an effective source of emf. The polarity of this effective source is such to move current in the direction to the opposite that you have assumed.

Generally, the following rule is useful: draw a voltage arrow for a battery from the negative terminal to the positive terminal, the voltage arrow across a resistor in the opposite direction to the current flow through it and for the capacitor draw the voltage arrow in the direction from the plate that is accumulating negative charge to the plate that is accumulating positive charge. This gives the first figure.

Kirchhoff's voltage law is used by starting at any point in the circuit and going around in a closed loop, counting a voltage as positive if you travel in the same direction as the arrow and negative otherwise. In the first circuit this starting at A and going around the circuit in a clockwise direction gives $+E - \frac{Q}{C} - IR = 0$.

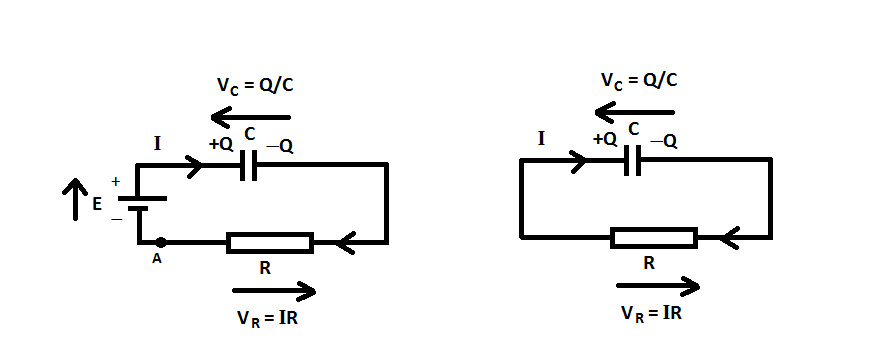
enter image description here

When the battery is removed if you follow the same conventions you see the current comes out negative.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.