2
$\begingroup$

Here the study:

An external system (not drawn) give energy for rotate disks around themselves and around green axis. All disks have energy at start, at $t=0$ friction is ON and at $t=0$ external system is OFF (it don't add energy to disks). I think this energy must be conserved in this case.

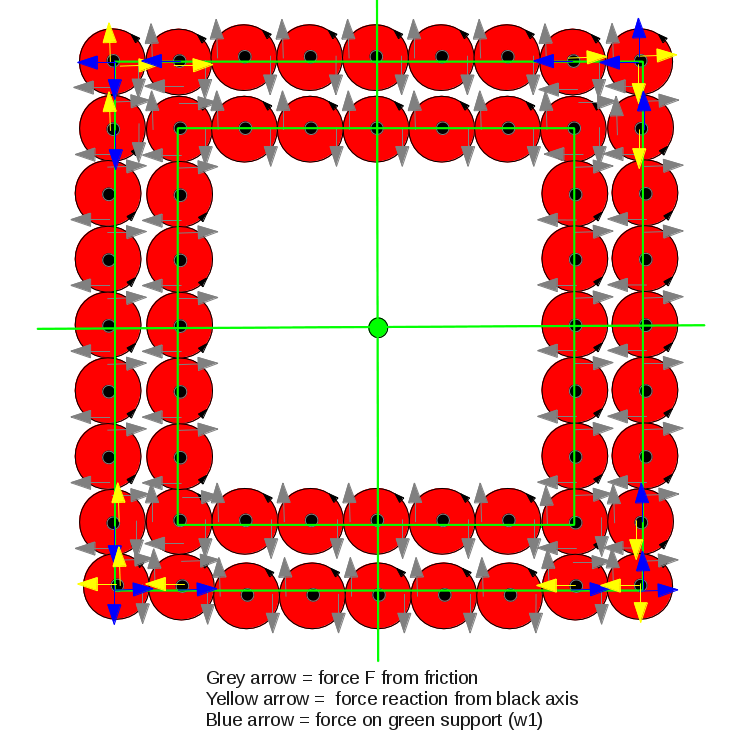
2 Layers of red disks turn at $w_1$ clockwise around green axis (center of the image). All red disks are link together with green stems. All red disks turn at $w_2$ anticlockwise around their center of gravity for the first layer and at $w_2'$ anticlockwise around their center of gravity for the second layer. There is force F when disks touch another one. Red disk touch disk side by side but it is only at the 4 corners that disks touch layer1/layer2.

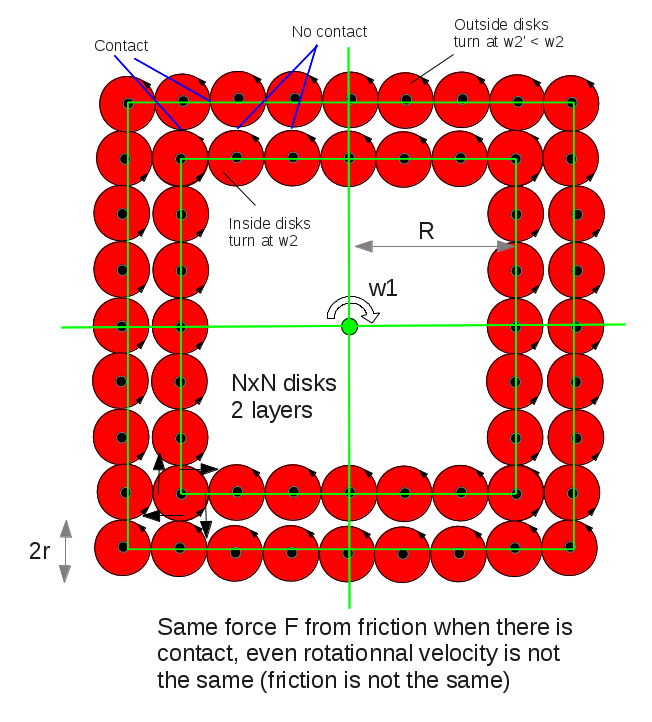
enter image description here

$R$ = Radius of first square layer (a side of inside square = 2R)
$r$ = radius of red disk
$F$ = basic force from friction
$t$ = time
$w_2' < w_2 $
$R = 6r $
$w_1 > w_2$

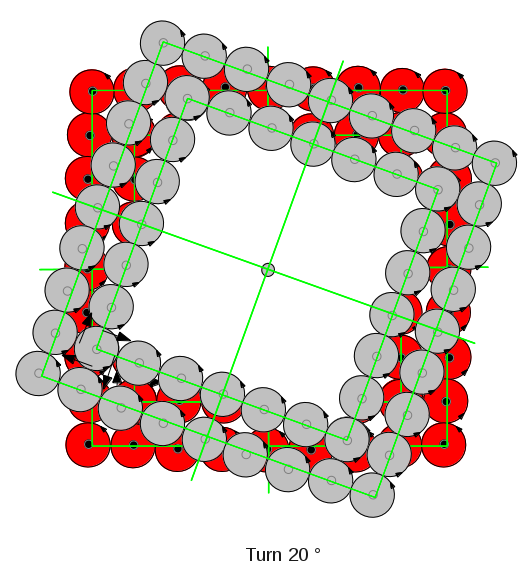
enter image description here

I compute work from torques:

There are 24 disks inside and 32 disks outside.

  • Disks: +24+32+8 torques on red disks = $Frt( 48(w_1-w_2)+64(w_1-w_2')+8(w_1-w_2)+8(w_1-w_2')$
  • Friction : $+ 24Fr(w_2+w_2)t + 32Fr(w_2'+w_2')t + 8Fr(w_2+w_2')t $
  • Corners: -8 torques layer1 - 8 torque layer2 $= -8(R+2r)Fw_1t -8(R+2r)Fw_1t= -8(6r+2r)Fw_1t-64Frw_1t = -128Frw_1t$

The sum of works from torque = $128Frtw_1-56Frtw_2-72Frtw_2' +56Frtw_2+72Frtw_2' -128Frw_1t$

The sum = 0

All is fine !

All forces from friction for watch where there is contact

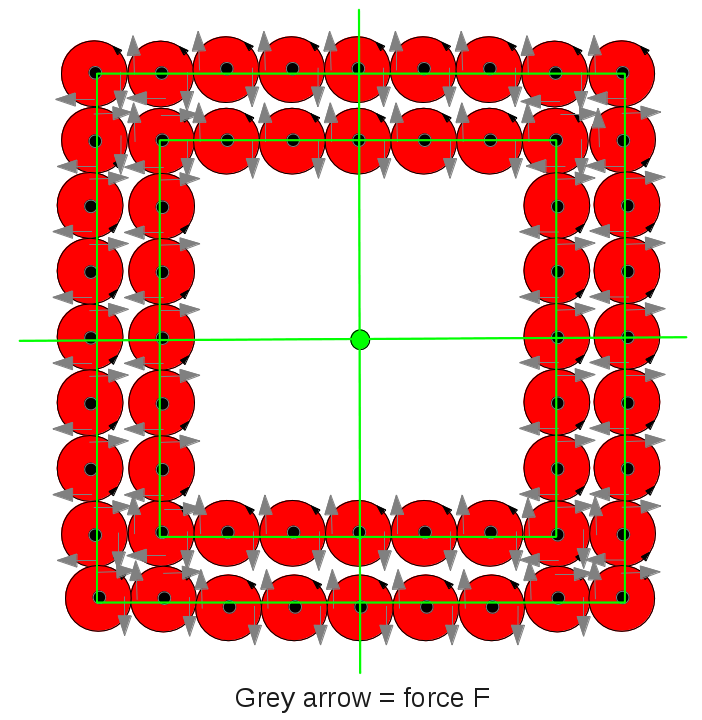
enter image description here

I hope I don't forgot one :)

All force

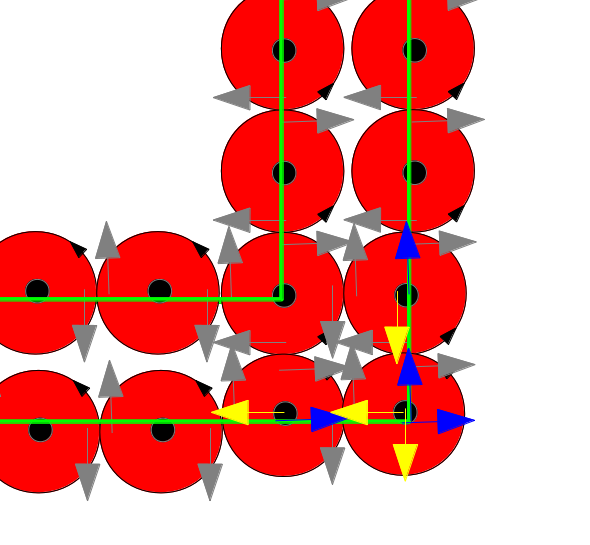
enter image description here

Zoom of a corner

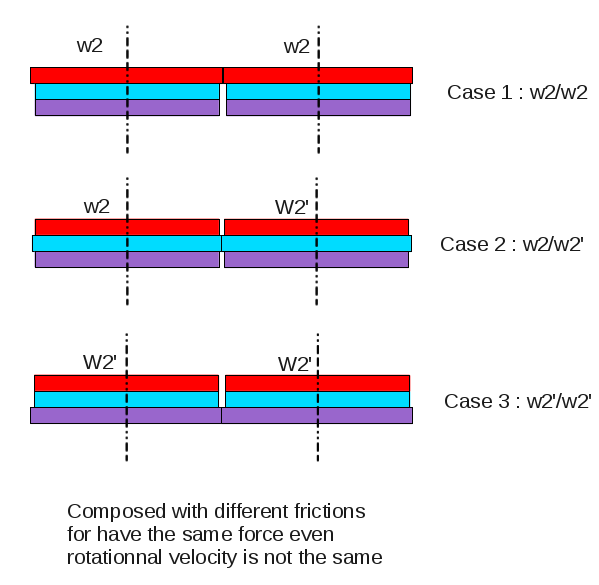
enter image description here

Disks can be like that:

enter image description here

And this give the possibility for composed friction :

enter image description here

Improve this question
$\endgroup$
7
  • $\begingroup$ What drives the disks? $\endgroup$
    – John Alexiou
    Commented Oct 26, 2014 at 4:43
  • $\begingroup$ I reply in the question, it's an external system that give energy just before t=0. At t=0, friction is ON. And at t=0 the external system is OFF. $\endgroup$
    – Sx7
    Commented Oct 26, 2014 at 8:18
  • 1
    $\begingroup$ I added all informations I can, what's wrong with my question ? It's because it is too specific ? But I don't find another question in this field in Physics ST or another forum in Google. $\endgroup$
    – Sx7
    Commented Oct 27, 2014 at 7:33
  • $\begingroup$ 2nd law applies to isolated systems, and when you have friction it is no longer and isolated system (unless you are capturing the heat generated). $\endgroup$
    – John Alexiou
    Commented Oct 27, 2014 at 12:57
  • 1
    $\begingroup$ If two disks are contacting each other, then the clockwise rotation of one induces a counter-clockwise rotation in the other. So unless you are driving the rotation of those disks, this will not work. $\endgroup$
    – Kyle Kanos
    Commented Oct 27, 2014 at 14:02

0

Reset to default