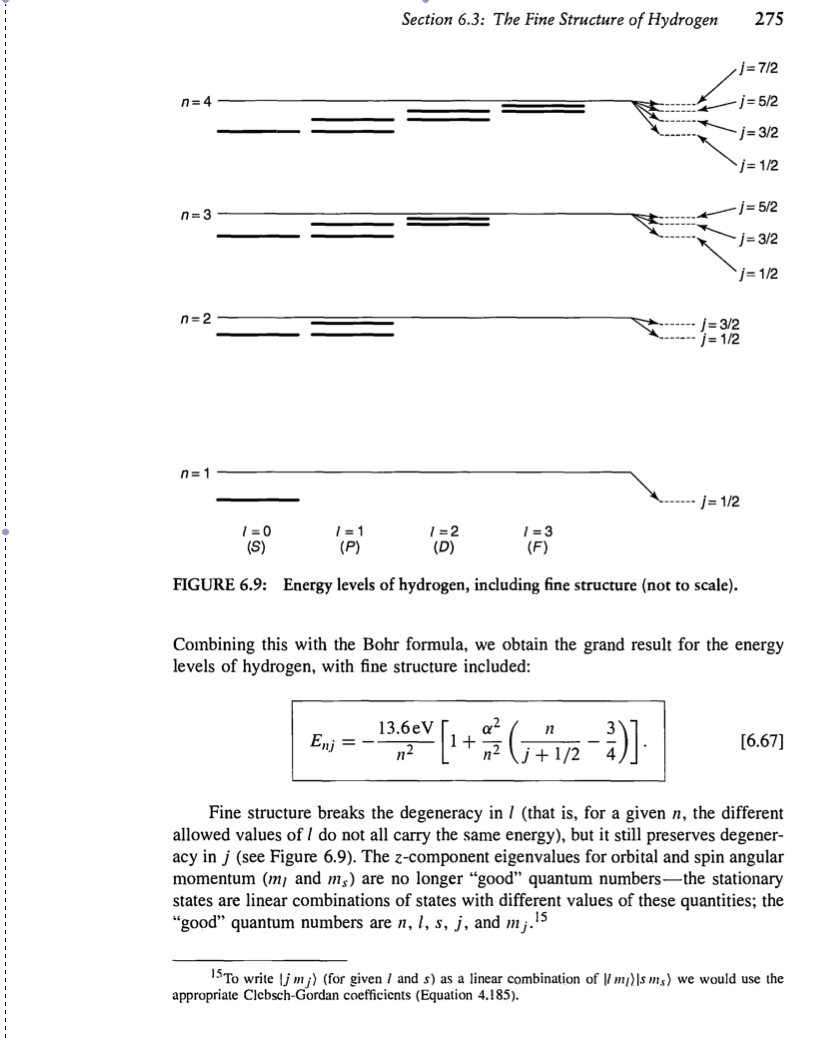
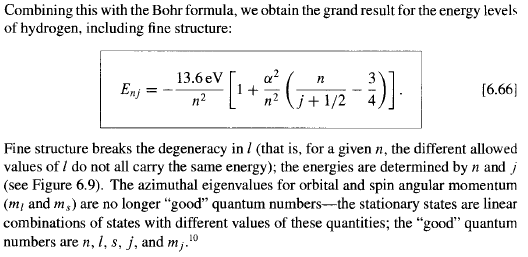4
$\begingroup$

Note: We are dealing with perturbation on the states $|nlm_lm_s>$ where n is the principle quantum number, l is the angular momentum quantum number, and $m_l$ and $m_s$ are the eigenvalues of $L_z$ and $S_z$ corresponding to this particular eigenstate. j is the Total angular momentum quantum number: $j = l \pm 1/2 $.

In page 275 of Griffiths, he finishes the derivation of the energy perturbation due to the fine structure correction. He then says that the fine structure breaks degeneracy in l but not in j. Why is this? It seems to me that the degeneracy in j is broken, and that there is still degeneracy in l.

Griffiths Text

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

In my 1995 edition the text (on page 242) reads:

1995 edition

So presumably this is an error in whatever edition you have.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks. I would upvote, but I have not the reputation to do so. $\endgroup$
    – Zane Dufour
    Commented Oct 3, 2014 at 1:32
  • $\begingroup$ @ZaneDufour: if this answers your question please click on the tick symbol to accept the answer. $\endgroup$
    – John Rennie
    Commented Oct 3, 2014 at 4:55
  • $\begingroup$ Sadly, the 3rd edition (2018) reverted back to the error reported by @ZaneDufour $\endgroup$
    – Job Stancil
    Commented Jul 20, 2021 at 10:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.