I can't reconcile some facts about entropy and irreversibility. This depresses me, because I feel I can't quite grasp the importance of entropy.
I will illustrate my problems with an example given by my book:
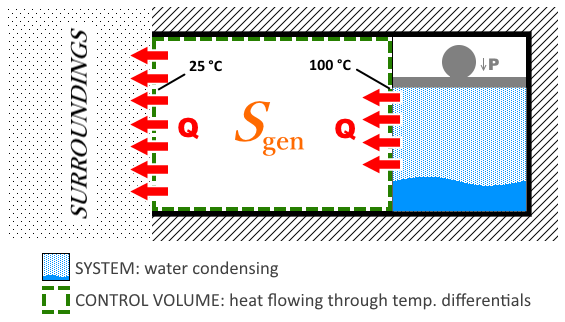
1 kg of liquid water at 100 °C is condensed at constant pressure via heat transfer to the surrounding air which is at 25 °C. What is the net increase of entropy? ($s_{lv}$ = 6.048 kJ/kg·K, $h_{lv}$ = 2,257 kJ/kg)
Solution
$\Delta S_\text{system} = m \cdot \left(-s_{lv}\right) = -6.048 \frac{\text{kJ}}{\text{K}}$
$Q_\text{to surr} = m \cdot h_{lv} = 2257 \text{ kJ}$
$\Delta S_\text{surr} = \frac{Q}{T_0} = \frac{2257}{273.15+25} = 7.570 \frac{\text{kJ}}{\text{K}}$
$$\boxed{\Delta S_\text{net} = \Delta S_\text{system} + \Delta S_\text{surr} = 1.522 \frac{\text{kJ}}{\text{K}}}$$
I can easily follow that. However...
Problem 1: entropy variation is just like that of a reversible process
My book says that, for a system undergoing an irreversible process: $$dS \ge \frac{\delta Q}{T}$$ Specifically, $$dS = \frac{\delta Q}{T} + \delta S_\text{gen}$$
However, the example above, which is an irreversible process (finite temperature difference), has exactly $\Delta S = \frac{Q}{T}$, completely disregarding the generated entropy (which is $\Delta S_\text{gen} = \Delta S_\text{net}$).
In other words, we have $Q = T\Delta S$, which everyone knows is just for reversible processes.
Problem 2: dissipated heat is just like that of a reversible process
The book is quick to do $Q = \Delta H$, but it is known that that equation is only for reversible processes -- this is written in the chapter about the First Law.
Also, there's this equation, which says that, in an irreversible process, the exchanged heat must be less than that of a reversible one between the same states: $$\delta Q_\text{irr} = T\ dS - T\ \delta S_\text{gen}$$
Once again, the generated entropy is ignored and we have, like above, $Q = T\Delta S$.
Because of the above, I really can't see what difference would it make to the process if it were reversible, that is, if it exchanged heat with a reservoir at the same temperature as the water. Please, can anyone make sense of it?